You are here
Question 2 - Whole row/column zero - Chapter 4 Class 12 Determinants
Last updated at Dec. 16, 2024 by Teachoo
Chapter 4 Class 12 Determinants
Concept wise
- Finding determinant of a 2x2 matrix
- Evalute determinant of a 3x3 matrix
- Area of triangle
- Equation of line using determinant
- Finding Minors and cofactors
- Evaluating determinant using minor and co-factor
- Find adjoint of a matrix
- Finding Inverse of a matrix
- Inverse of two matrices and verifying properties
- Finding inverse when Equation of matrice given
- Checking consistency of equations
- Find solution of equations- Equations given
- Find solution of equations- Statement given
- Verifying properties of a determinant
- Two rows or columns same
-
Whole row/column zero
- Whole row/column one
- Making whole row/column one and simplifying
- Proving Determinant 1 = Determinant 2
- Solving by simplifying det.
- Using Property 5 (Determinant as sum of two or more determinants)
Transcript
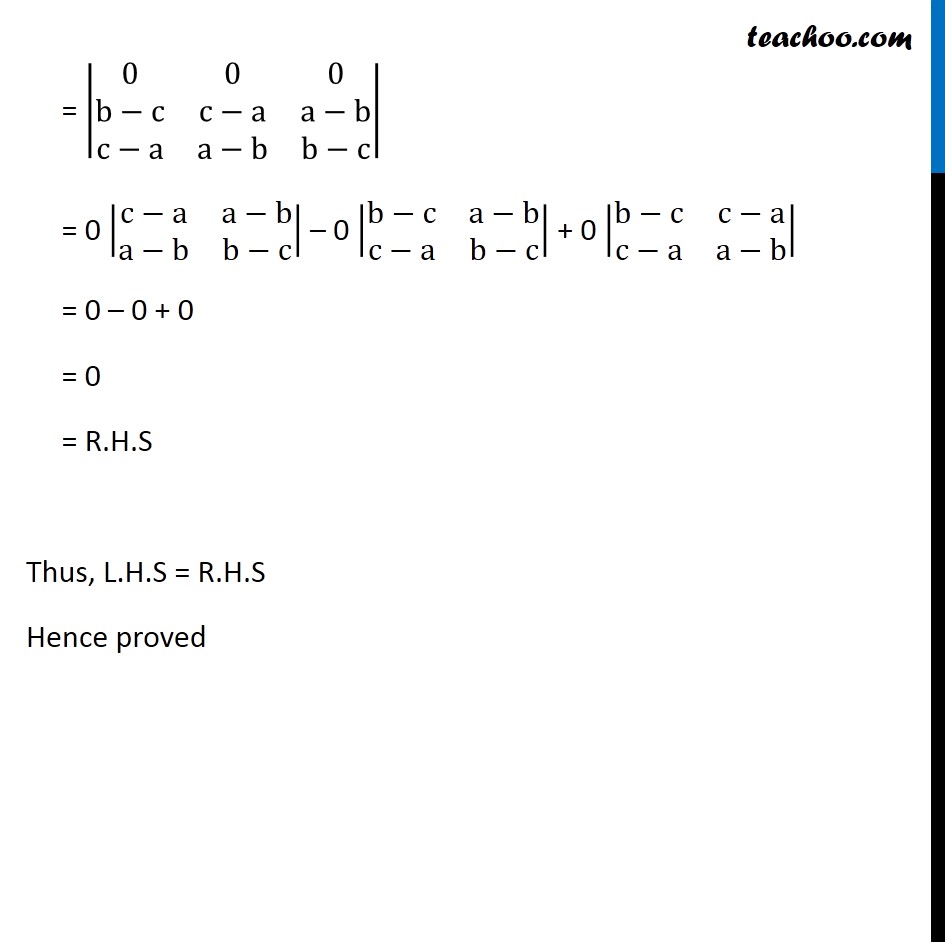
Question 2 Using the property of determinants and without expanding, prove that: |■8(a−b&b−c&c−𝑎@b−c&c−a&a−b@c−a&a−b&b−c)| = 0 |■8(a−b&b−c&c−𝑎@b−c&c−a&a−b@c−a&a−b&b−c)| Making first row 0 by Applying R1 →R1 + R2 + R3 = |■8(a−b+b−c+c−a&b−c+c−a+a−b&c−a+a−b+b−c@b−c&c−a&a−b@c−a&a−b&b−c)| = |■8(0&0&0@b−c&c−a&a−b@c−a&a−b&b−c)| = 0 |■8(c−a&a−b@a−b&b−c)| – 0 |■8(b−c&a−b@c−a&b−c)| + 0 |■8(b−c&c−a@c−a&a−b)| = 0 – 0 + 0 = 0 = R.H.S Thus, L.H.S = R.H.S Hence proved
