Example 18 - Chapter 3 Class 12 Matrices (Important Question)

Transcript

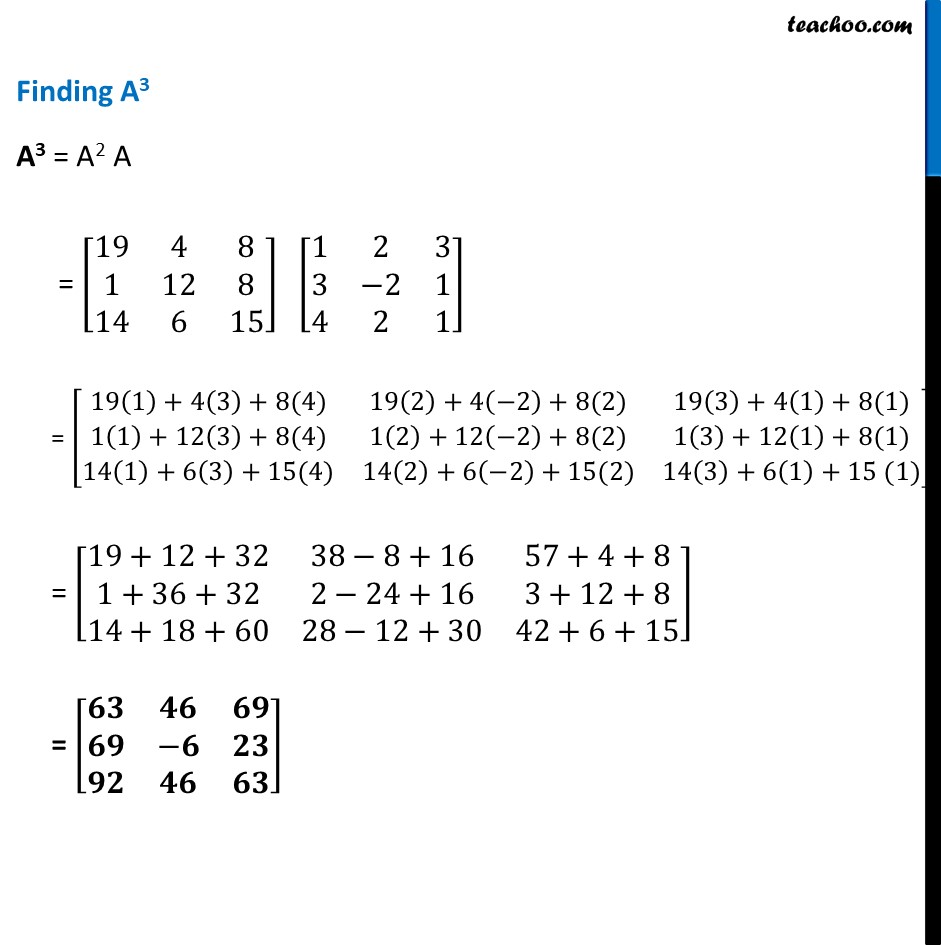
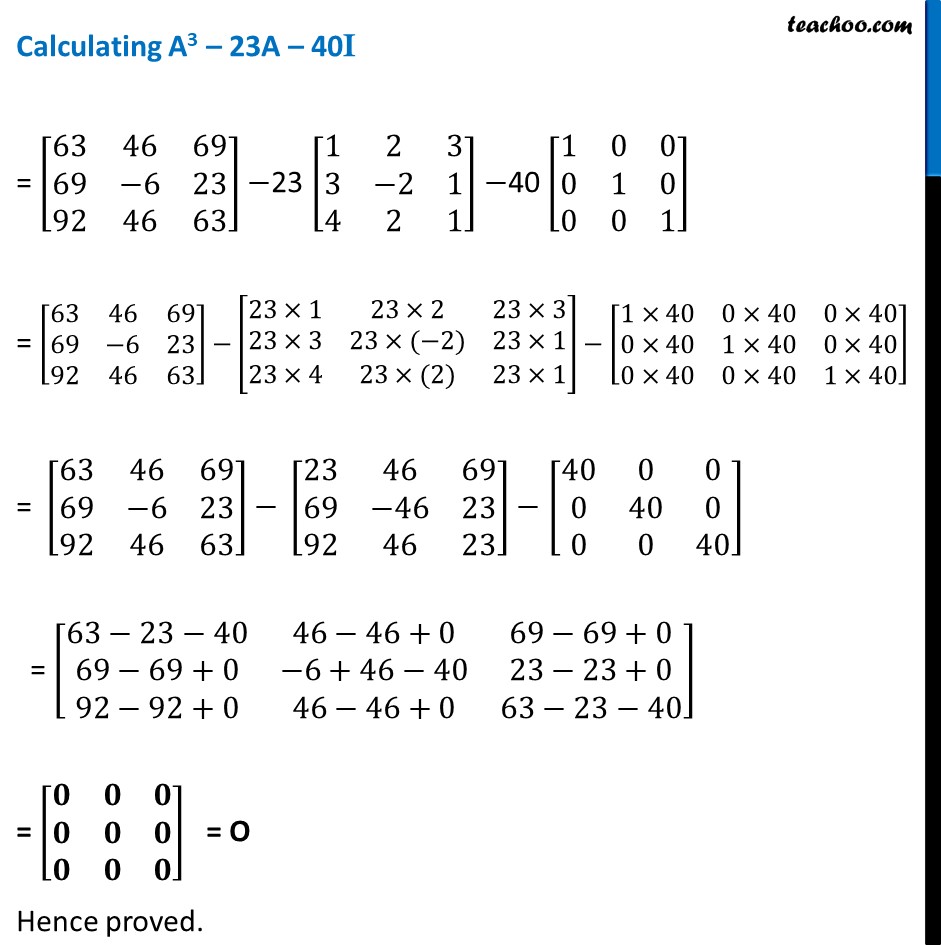
Example 18 If A = [■8(1&2&3@3&−2&1@4&2&1)] then show that A3 – 23A – 40I = O Finding A2 A2 = AA = [■8(1&2&3@3&−2&1@4&2&1)] [■8(1&2&3@3&−2&1@4&2&1)] = [■8(1(1)+2(3)+3(4)&1(2)+2(−2)+3(2)&1(3)+2(1)+3(1)@3(1)+(−2)(3)+1(4)&3(2)+(−2)(−2)+1(2)&3(3)+(−2)(1)+1(1)@4(1)+2(3)+1(4)&4(2)+2(−2)+1(2)&4(3)+(2)(1)+1(1))] = [■8(1+6+12&2−4+6&3+2+3@3−6+4&6+4+2&9−2+1@4+6+4&8−4+2&12+2+1)] = [■8(𝟏𝟗&𝟒&𝟖@𝟏&𝟏𝟐&𝟖@𝟏𝟒&𝟔&𝟏𝟓)] Finding A3 A3 = A2 A = [■8(19&4&8@1&12&8@14&6&15)] [■8(1&2&3@3&−2&1@4&2&1)] = [■8(19(1)+4(3)+8(4)&19(2)+4(−2)+8(2)&19(3)+4(1)+8(1)@1(1)+12(3)+8(4)&1(2)+12(−2)+8(2)&1(3)+12(1)+8(1)@14(1)+6(3)+15(4)&14(2)+6(−2)+15(2)&14(3)+6(1)+15 (1))] = [■8(19+12+32&38−8+16&57+4+8@1+36+32&2−24+16&3+12+8@14+18+60&28−12+30&42+6+15)] = [■8(𝟔𝟑&𝟒𝟔&𝟔𝟗@𝟔𝟗&−𝟔&𝟐𝟑@𝟗𝟐&𝟒𝟔&𝟔𝟑)] Calculating A3 – 23A – 40I = [■8(63&46&69@69&−6&23@92&46&63)] −23 [■8(1&2&3@3&−2&1@4&2&1)] −40 [■8(1&0&0@0&1&0@0&0&1)] = [■8(63&46&69@69&−6&23@92&46&63)] −[■8(23×1&23×2&23×3@23×3&23×(−2)&23×1@23×4&23×(2)&23×1)] − [■8(1×40&0×40&0×40@0×40&1×40&0×40@0×40&0×40&1×40)] = [■8(63&46&69@69&−6&23@92&46&63)] ⤶7− [■8(23&46&69@69&−46&23@92&46&23)] ⤶7− [■8(40&0&0@0&40&0@0&0&40)] = [■8(63−23−40&46−46+0&69−69+0@69−69+0&−6+46−40&23−23+0@92−92+0&46−46+0&63−23−40)] = [■8(𝟎&𝟎&𝟎@𝟎&𝟎&𝟎@𝟎&𝟎&𝟎)] = O Hence proved.