You are here
![Example 10 - Find x and y: 2[x 5 7 y-3] + [3 -4 1 2] - Examples - Examples](https://cdn.teachoo.com/412f683b-90bd-4748-892b-bd37d19b180d/slide22.jpg)
Example 10 - Chapter 3 Class 12 Matrices
Last updated at August 21, 2026 by Teachoo
Chapter 3 Class 12 Matrices
Concept wise
- Formation and order of matrix
- Types of matrices
- Equal matrices
-
Addition/ subtraction of matrices
- Statement questions - Addition/Subtraction of matrices
- Multiplication of matrices
- Statement questions - Multiplication of matrices
- Solving Equation
- Finding unknown - Element
- Finding unknown - Matrice
- Transpose of a matrix
- Symmetric and skew symmetric matrices
- Proof using property of transpose
- Inverse of matrix using elementary transformation
- Proof using mathematical induction
Transcript
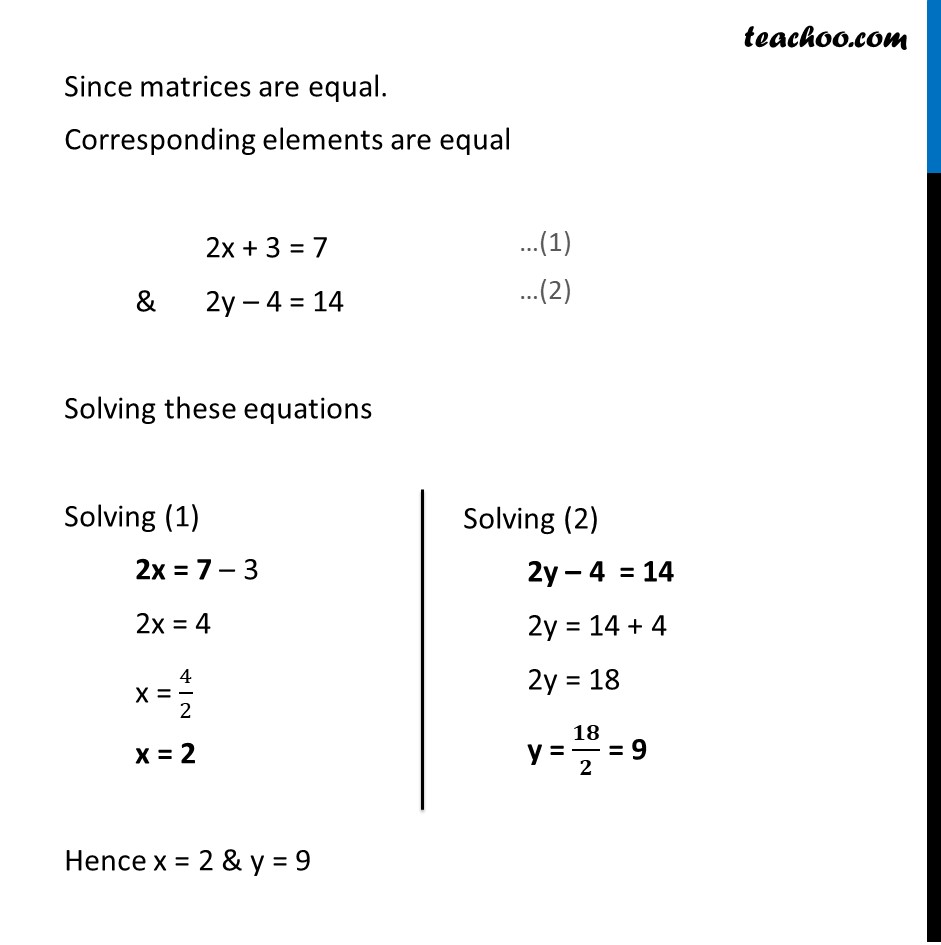
Example 10 Find the values of x and y from the following equation: 2[■8(𝑥&5@7&𝑦−3)] + [■8(3&−4@1&2)] = [■8(7&6@15&14)] 2[■8(𝑥&5@7&𝑦−3)] + [■8(3&−4@1&2)] = [■8(7&6@15&14)] [■8(𝑥 × 2&5 × 2@7 × 2&(𝑦−3)× 2)] + [■8(3&−4@1&2)] = [■8(7&6@15&14)] [■8(𝟐𝒙&𝟏𝟎@𝟏𝟒&𝟐𝒚 −𝟔)] + [■8(𝟑&−𝟒@𝟏&𝟐)] = [■8(𝟕&𝟔@𝟏𝟓&𝟏𝟒)] [■8(2𝑥+3&10 −4@14+1&2𝑦 −6+2)] = [■8(7&6@15&14)] [■8(𝟐𝒙+𝟑&𝟔@𝟏𝟓&𝟐𝒚 −𝟒)] = [■8(𝟕&𝟔@𝟏𝟓&𝟏𝟒)] Since matrices are equal. Corresponding elements are equal 2x + 3 = 7 & 2y – 4 = 14 Solving these equations Solving (1) 2x = 7 – 3 2x = 4 x = 4/2 x = 2 Hence x = 2 & y = 9 Solving (2) 2y – 4 = 14 2y = 14 + 4 2y = 18 y = 𝟏𝟖/𝟐 = 9
