You are here
Example 9 - Chapter 3 Class 12 Matrices
Last updated at Dec. 16, 2024 by Teachoo
Chapter 3 Class 12 Matrices
Concept wise
- Formation and order of matrix
- Types of matrices
- Equal matrices
-
Addition/ subtraction of matrices
- Statement questions - Addition/Subtraction of matrices
- Multiplication of matrices
- Statement questions - Multiplication of matrices
- Solving Equation
- Finding unknown - Element
- Finding unknown - Matrice
- Transpose of a matrix
- Symmetric and skew symmetric matrices
- Proof using property of transpose
- Inverse of matrix using elementary transformation
- Proof using mathematical induction
Transcript
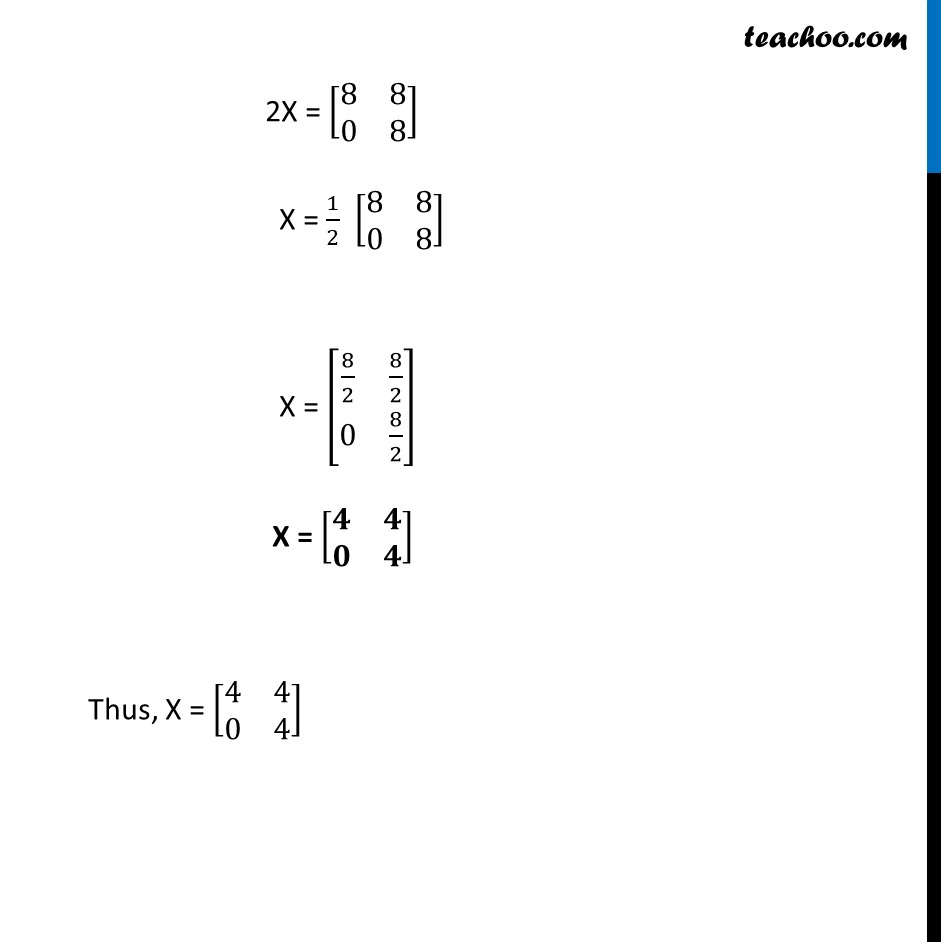
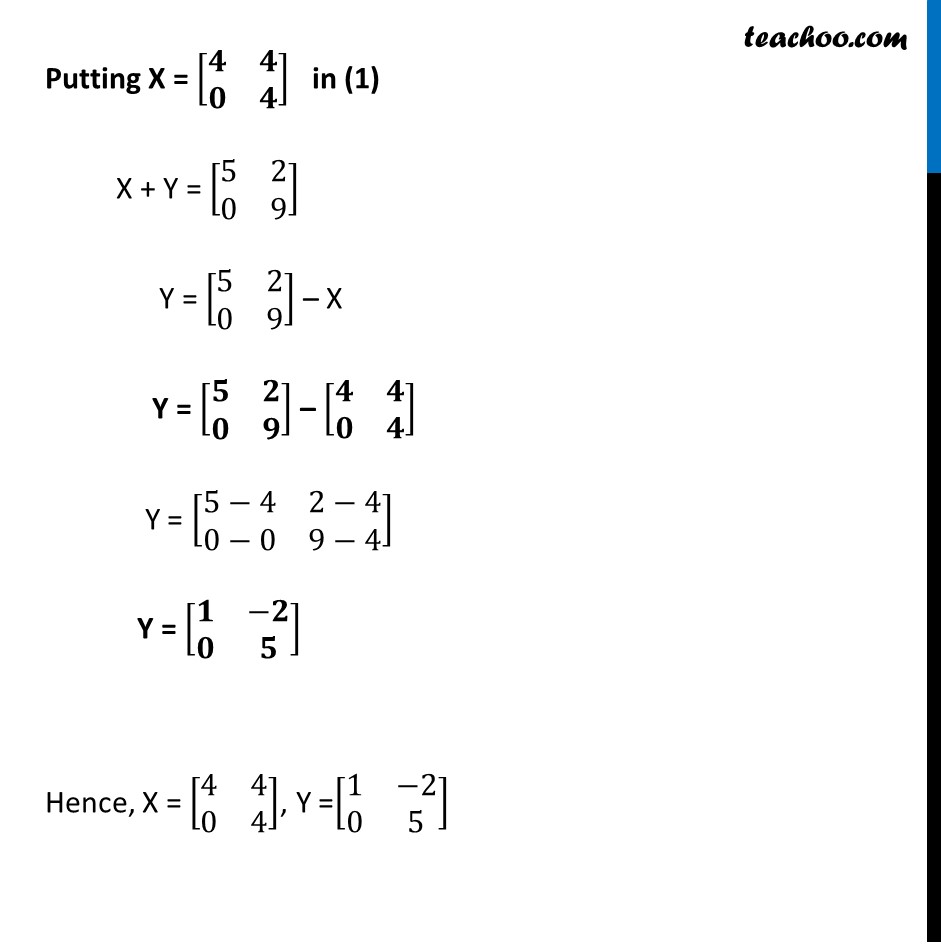
Example 9 Find X and Y , if X + Y = [■8(5&2@0&9)] and X – Y = [■8(3&6@0&−1)] It is given that X + Y = [■8(5&2@0&9)] X – Y = [■8(3& 6@0&−1)] Adding (1) and (2) (X + Y) + (X – Y) = [■8(5&2@0&9)] + [■8(3&6@0&−1)] X + X + Y – Y = [■8(5+3&2 +6@0+0&9+( −1))] 2X = [■8(8&8@0&8)] X = 1/2 [■8(8&8@0&8)] X = [■8(8/2&8/2@0&8/2)] X = [■8(𝟒&𝟒@𝟎&𝟒)] Thus, X = [■8(4&4@0&4)] Putting X = [■8(𝟒&𝟒@𝟎&𝟒)] in (1) X + Y = [■8(5&2@0&9)] Y = [■8(5&2@0&9)] – X Y = [■8(𝟓&𝟐@𝟎&𝟗)] – [■8(𝟒&𝟒@𝟎&𝟒)] Y = [■8(5−4&2−4@0−0&9−4)] Y = [■8(𝟏&−𝟐@𝟎&𝟓)] Hence, X = [■8(4&4@0&4)], Y =[■8(1&−2@0&5)]
