![Ex 3.4, 4 - Find inverse [2 3 5 7] - Class 12 CBSE - Inverse of matrix using elementary transformation](https://cdn.teachoo.com/40f96f7c-2827-48d6-8a1b-79f30822c27a/slide8.jpg)
Question 4 - Inverse of matrix using elementary transformation - Chapter 3 Class 12 Matrices
Last updated at Dec. 16, 2024 by Teachoo
Inverse of matrix using elementary transformation
Elementary operations on a matrix
You are here
You are here
Chapter 3 Class 12 Matrices
Concept wise
- Formation and order of matrix
- Types of matrices
- Equal matrices
- Addition/ subtraction of matrices
- Statement questions - Addition/Subtraction of matrices
- Multiplication of matrices
- Statement questions - Multiplication of matrices
- Solving Equation
- Finding unknown - Element
- Finding unknown - Matrice
- Transpose of a matrix
- Symmetric and skew symmetric matrices
- Proof using property of transpose
-
Inverse of matrix using elementary transformation
- Proof using mathematical induction
Transcript
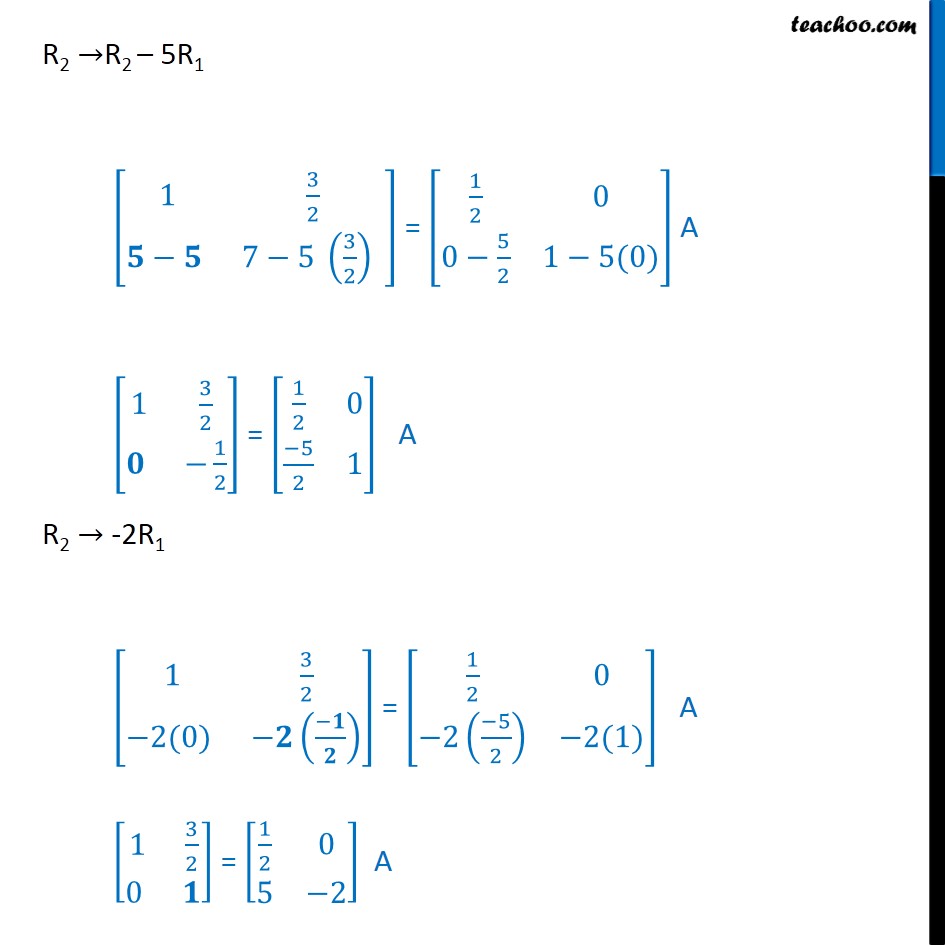
Ex3.4, 4 Find the inverse of each of the matrices, if it exists. [■8(2&3@5&7)] Let A = [■8(2&3@5&7)] We know that A = IA [■8(2&3@5&7)] = [■8(1&0@0&1)] A R1→1/2R1 [■8(𝟐/𝟐&3/2@5&7)] = [■8(1/2&0/2@0&1)] A [■8(𝟏&3/2@5" " &7" " )] = [■8(1/2&0@0&1)] A R2 →R2 – 5R1 [■8(1&3/2@𝟓−𝟓" " &7−5 (3/2)" " )] = [■8(1/2&0@0−5/2&1−5(0))] A [■8(1&3/2@𝟎" " &−1/2)] = [■8(1/2&0@(−5)/2&1)] A R2 → -2R1 [■8(1&3/2@−2(0)" " &−𝟐((−𝟏)/𝟐) )] = [■8(1/2&0@−2((−5)/2)&−2(1))] A [■8(1&3/2@0" " &𝟏)] = [■8(1/2&0@5&−2)] A R1 →R1 – 3/2 R2 [■8(1−0(3/2)&𝟑/𝟐−𝟑/𝟐(𝟏)@0" " &1)] = [■8(1/2−3/2(5)&0−3/2(−2)@5&−2)] A [■8(1&𝟎@0" " &1)] = [■8(−7&3@5&−2)] A This is similar to I = A-1A Thus, A-1 = [■8(−7&3@5&−2" " )]
