

Statement questions - Multiplication of matrices
Statement questions - Multiplication of matrices
Last updated at December 16, 2024 by Teachoo






Transcript
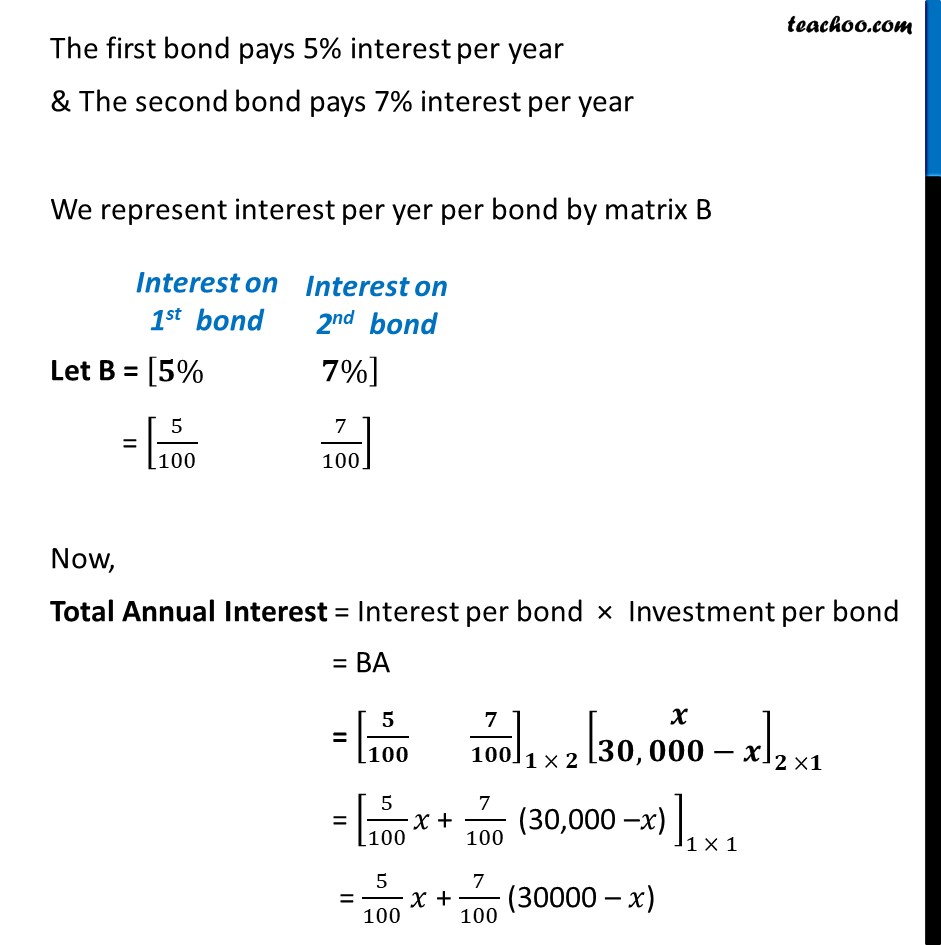
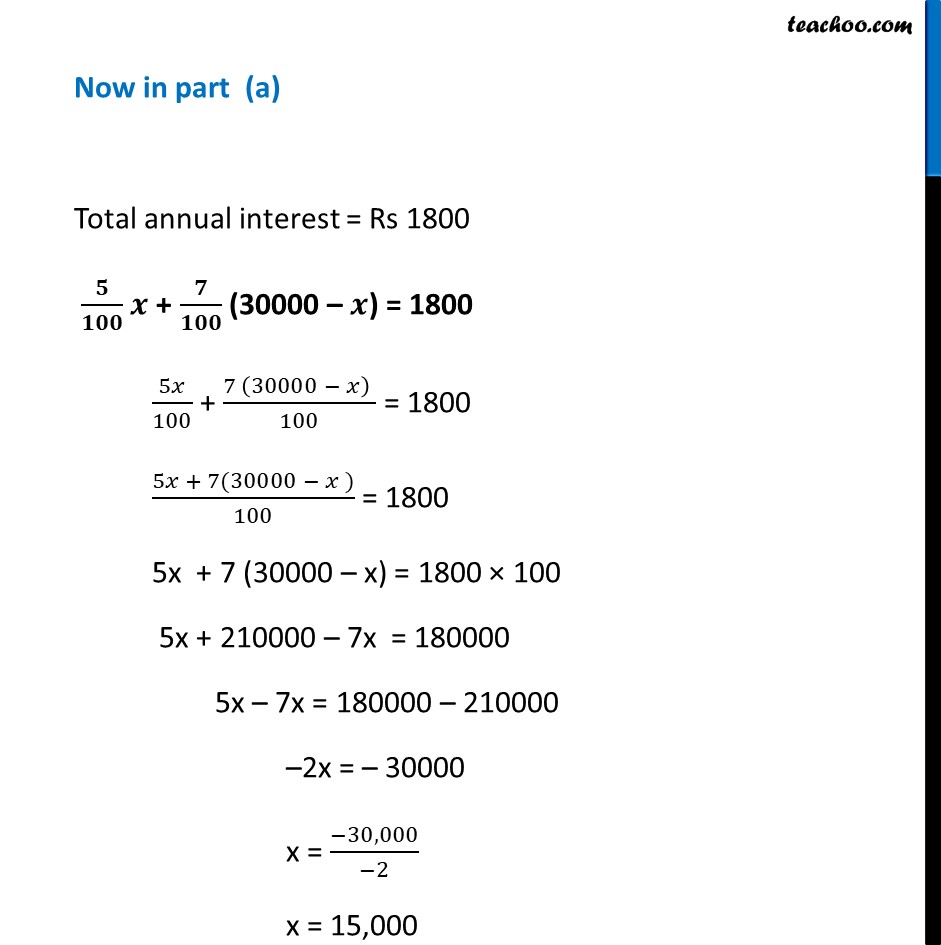
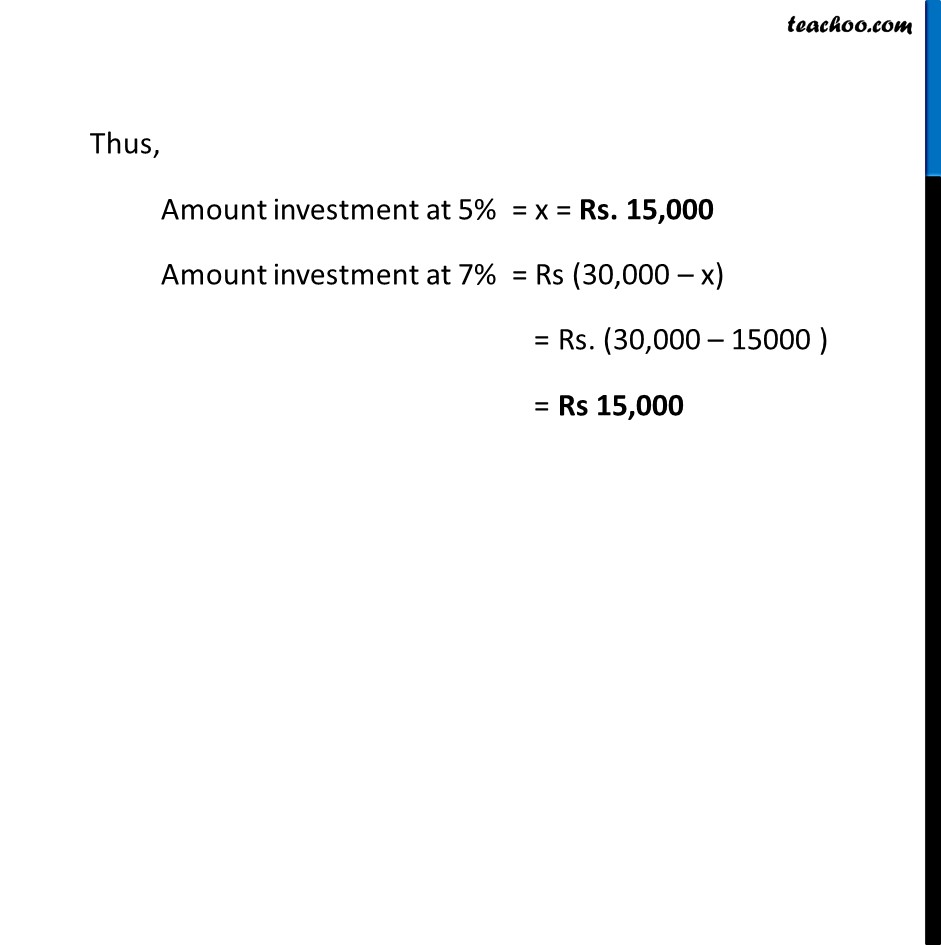
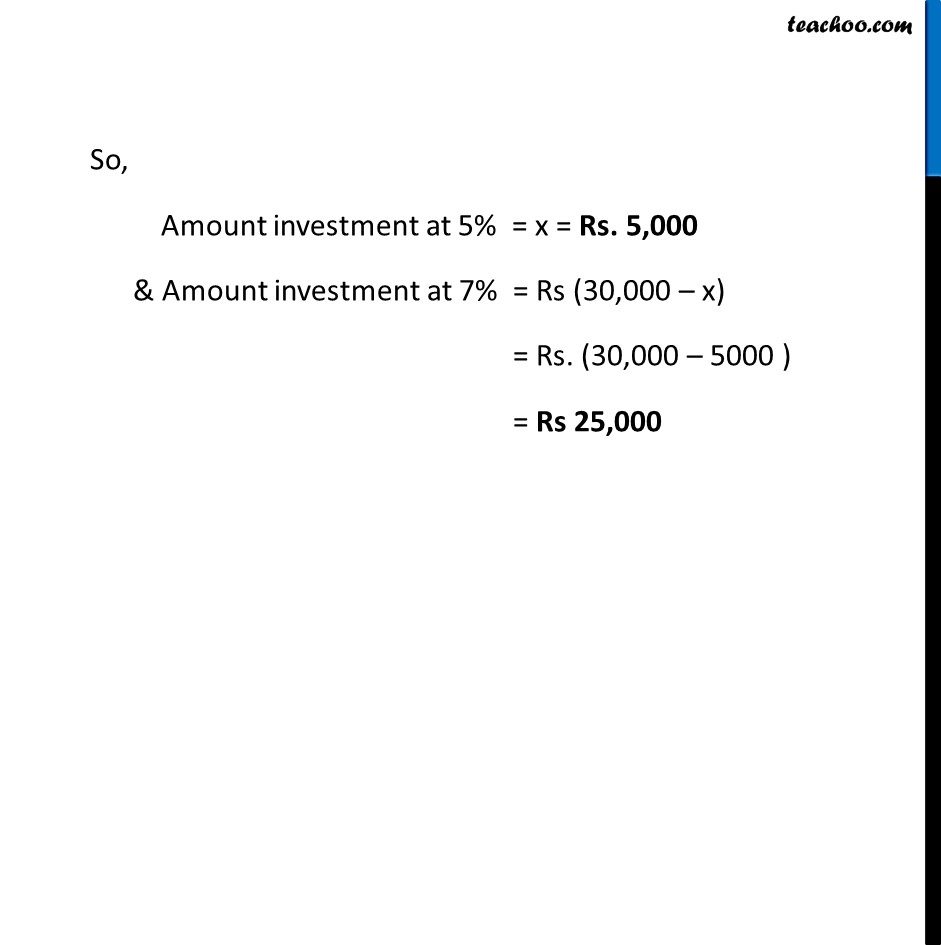
Ex 3.2, 19 A trust fund has Rs 30,000 that must be invested in two different types of bonds. The first bond pays 5% interest per year, and the second bond pays 7% interest per year. Using matrix multiplication, determine how to divide Rs 30,000 among the two types of bonds. If the trust fund must obtain an annual total interest of: Rs 1,800 (b) Rs 2000 Let Investment on 1st bond. = Rs x So, Investment on 2nd bond = Rs 30,000 – x We represent investment per bond by matrix A Let A = [■8(𝒙@𝟑𝟎,𝟎𝟎𝟎−𝒙)] The first bond pays 5% interest per year & The second bond pays 7% interest per year We represent interest per yer per bond by matrix B Let B = [■8(𝟓%& 𝟕%)] = [5/100 7/100] Now, Total Annual Interest = Interest per bond × Investment per bond = BA = [𝟓/𝟏𝟎𝟎 𝟕/𝟏𝟎𝟎]_(𝟏 × 𝟐) [■8(𝒙@𝟑𝟎,𝟎𝟎𝟎−𝒙)]_(𝟐 ×𝟏) = [5/100 𝑥" + " 7/100 " (30,000 –" 𝑥") " ]_(1 × 1) = 5/100 𝑥 + 7/100 (30000 – 𝑥) Now in part (a) Total annual interest = Rs 1800 𝟓/𝟏𝟎𝟎 𝒙 + 𝟕/𝟏𝟎𝟎 (30000 – 𝒙) = 1800 5𝑥/100 + (7 (30000 − 𝑥) )/100 = 1800 (5𝑥 + 7(30000 − 𝑥 ))/100 = 1800 5x + 7 (30000 – x) = 1800 × 100 5x + 210000 – 7x = 180000 5x – 7x = 180000 – 210000 –2x = – 30000 x = (−30,000)/(−2) x = 15,000 Thus, Amount investment at 5% = x = Rs. 15,000 Amount investment at 7% = Rs (30,000 – x) = Rs. (30,000 – 15000 ) = Rs 15,000 Now in part (b) Total annual interest = Rs 2000 𝟓/𝟏𝟎𝟎 𝒙 + 𝟕/𝟏𝟎𝟎 (30000 – 𝒙) = 2000 5𝑥/100 + (7 (30000 − 𝑥) )/100 = 2000 (5𝑥 + 7(30000 − 𝑥 ))/100 = 2000 5x + 7 (30000 – x) = 2000 × 100 5x + 210000 – 7x = 200000 5x – 7x = 200000 – 210000 –2x = – 10000 x = (−10,000)/(−2) x = 5,000 So, Amount investment at 5% = x = Rs. 5,000 & Amount investment at 7% = Rs (30,000 – x) = Rs. (30,000 – 5000 ) = Rs 25,000