Question 5 - Formulae based - Chapter 2 Class 12 Inverse Trigonometric Functions

Last updated at Dec. 16, 2024 by

Ex 2.2, 14 - If sin (sin-1 1/5 + cos-1 x) = 1, find x - CBSE

Ex 2.2, 14 - Chapter 2 Class 12 Inverse Trigonometric Functions - Part 2

Go Ad-free

Transcript

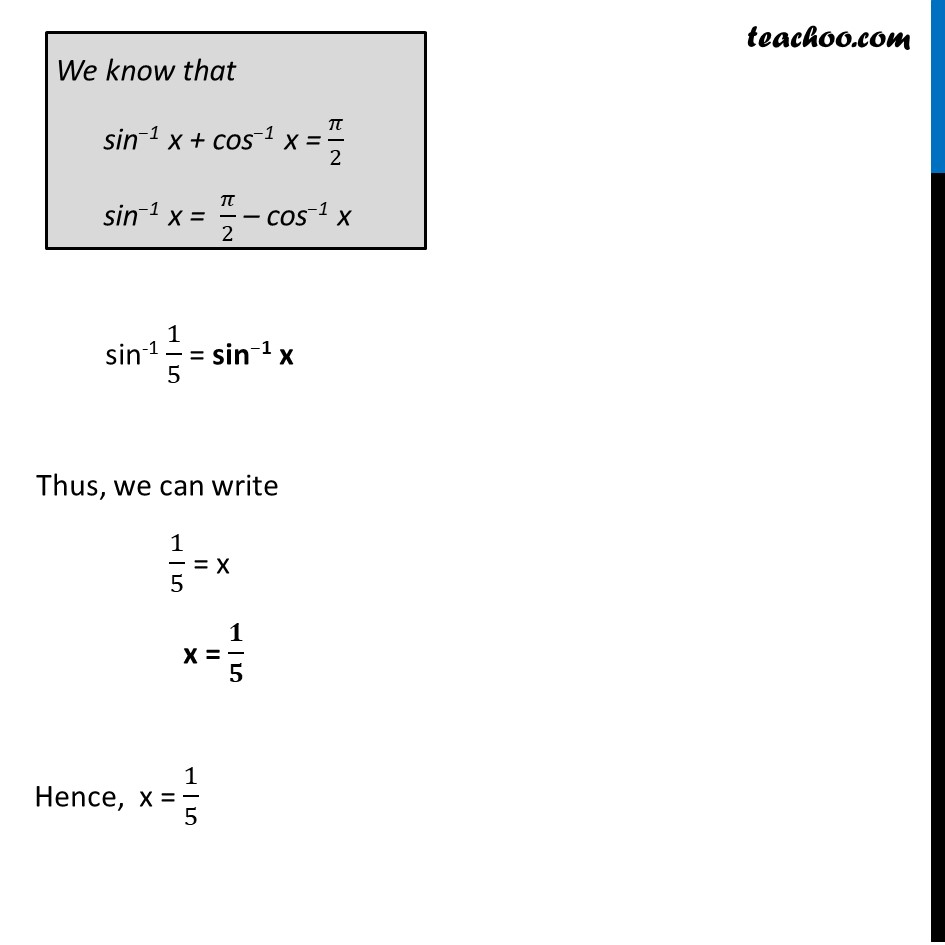
Question 5 If sin ("sin−1 " 1/5 " + cos−1 x" ) = 1 , then find the value of x. Given sin ("sin−1 " 1/5 " + cos−1 x" ) = 1 Putting sin 𝜋/2 = 1 sin ("sin−1 " 1/5 " + cos−1 x" ) = sin π/2 Comparing angles "sin−1 " 1/5 + "cos−1 x" = 𝜋/2 "sin−1 " 1/5 = 𝝅/𝟐 – "cos−1 x" We know that sin"−"1 x + cos"−"1 x = 𝜋/2 sin"−"1 x = 𝜋/2 – cos"−"1 x sin-1 1/5 = sin"−"1 x Thus, we can write 1/5 = x x = 𝟏/𝟓 Hence, x = 1/5

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo