Question 1 - Formulae based - Chapter 2 Class 12 Inverse Trigonometric Functions

Last updated at Dec. 16, 2024 by

Ex 2.2, 3 - Prove tan-1 2/11 + tan-1 7/24 = tan-1 1/2 - Ex 2.2

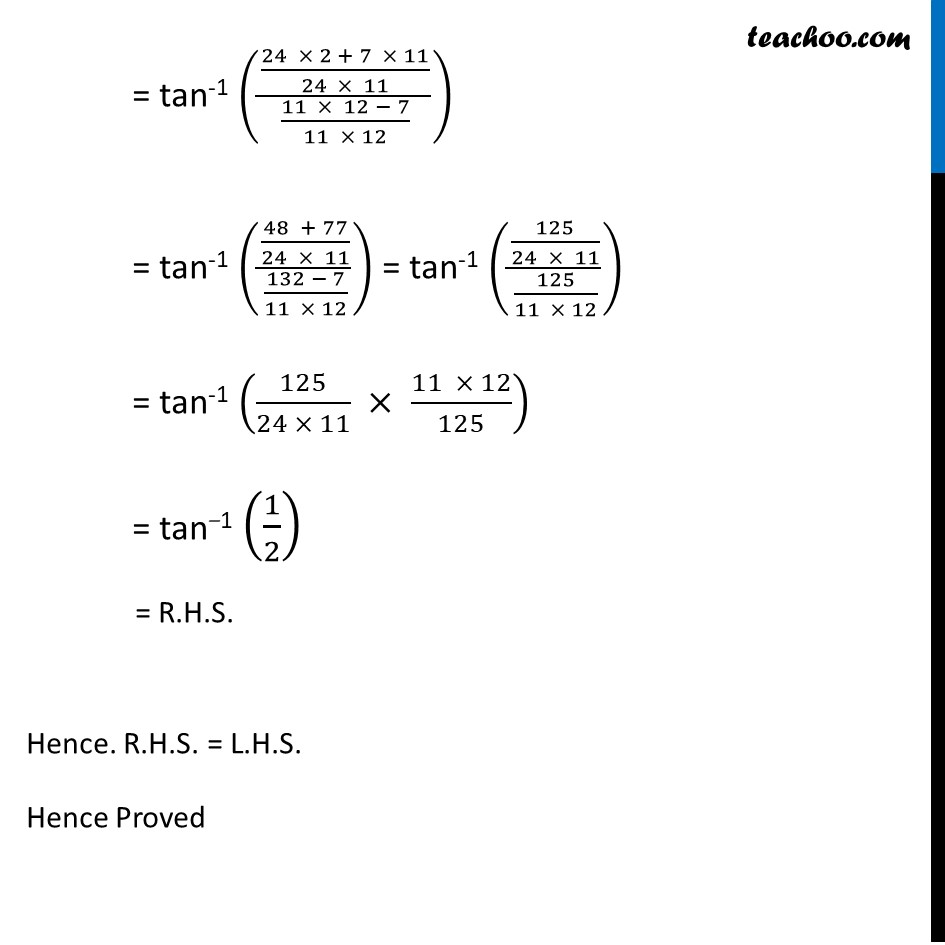
Ex 2.2, 3 - Chapter 2 Class 12 Inverse Trigonometric Functions - Part 2

Remove Ads

Transcript

Question 1 Prove tan−1 2/11 + tan−1 7/24 = tan−1 1/2 Solving L.H.S. tan-1 2/11 + tan−1 7/24 = "tan−1" ((2/11 + 7/24)/(1− 2/11 × 7/24)) We know that tan-1 x + tan−1 y = tan−1 ((𝒙+𝒚 )/(𝟏 −𝒙𝒚)) Replace x by 2/11 and y by 7/24= tan-1 (((24 × 2 + 7 × 11)/(24 × 11))/((11 × 12 − 7)/(11 × 12))) = tan-1 (((48 + 77)/(24 × 11))/((132 − 7)/(11 × 12))) = tan-1 ((125/(24 × 11))/(125/(11 × 12))) = tan-1 (125/(24 × 11) × (11 × 12)/125) = tan−1 (1/2) = R.H.S. Hence. R.H.S. = L.H.S. Hence Proved

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo