Misc 4 - Chapter 14 Class 11 Probability

Transcript

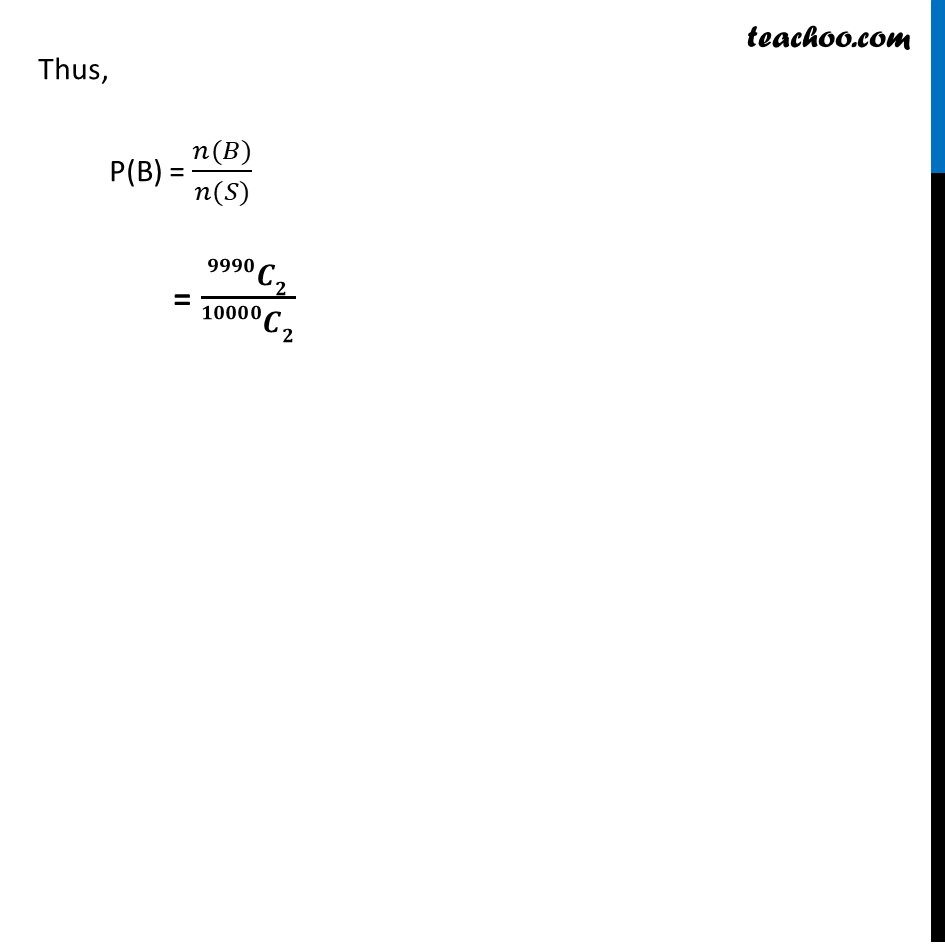
Misc 4 In a certain lottery 10,000 tickets are sold and ten equal prizes are awarded. What is the probability of not getting a prize if you buy (a) one ticket Since, 1 ticket is chosen out of 10000 tickets n(S) = 10000C1 = 10000!/1!9999! = (10000 × 9999!)/(1 × 9999!) = 10000 Now out of 10000 tickets only 10 have a prize Hence number of tickets not having prize = 10000 – 10 = 9990 Let A be the event that if we buy 1 ticket it does not have a prize Hence this 1 ticket will be out of 9990 ticket n(A) = 9990C1 = 9990!/1!(9990 − 1)! = 9990!/1!9989! = (9990 × 9989!)/(1 × 9989!) = 9990 Probability not getting a prize if we get one ticket = P(A) = (𝑛(𝐴))/(𝑛(𝑆)) = 9990/10000 = 𝟗𝟗𝟗/𝟏𝟎𝟎𝟎 Misc 4 In a certain lottery 10,000 tickets are sold and ten equal prizes are awarded. What is the probability of not getting a prize if you buy (B) Two tickets 2 ticket is chosen out of 10000 ticket Hence n(S) = 10000C2 Let B be the event that if we buy two ticket it does not have a prize Hence this 2 ticket will be out of 9990 ticket n(B) = 9990C2 Thus, P(B) = (𝑛(𝐵))/(𝑛(𝑆)) = 𝟗𝟗𝟗𝟎𝑪𝟐/𝟏𝟎𝟎𝟎𝟎𝑪𝟐 Misc 4 In a certain lottery 10,000 tickets are sold and ten equal prizes are awarded. What is the probability of not getting a prize if you buy (c) 10 ticket 10 ticket is chosen out of 10000 ticket Hence n(S) = 10000C10 Let C be the event that if we buy 10 tickets it does not have prize Hence these 10 ticket will be out of 9990 ticket So, n(C) = 9990C10 P(C) = (𝑛(𝐶))/(𝑛(𝑆)) = 𝟗𝟗𝟗𝟎𝑪𝟏𝟎/𝟏𝟎𝟎𝟎𝟎𝑪𝟏𝟎