




Miscellaneous
Last updated at December 16, 2024 by Teachoo






Transcript
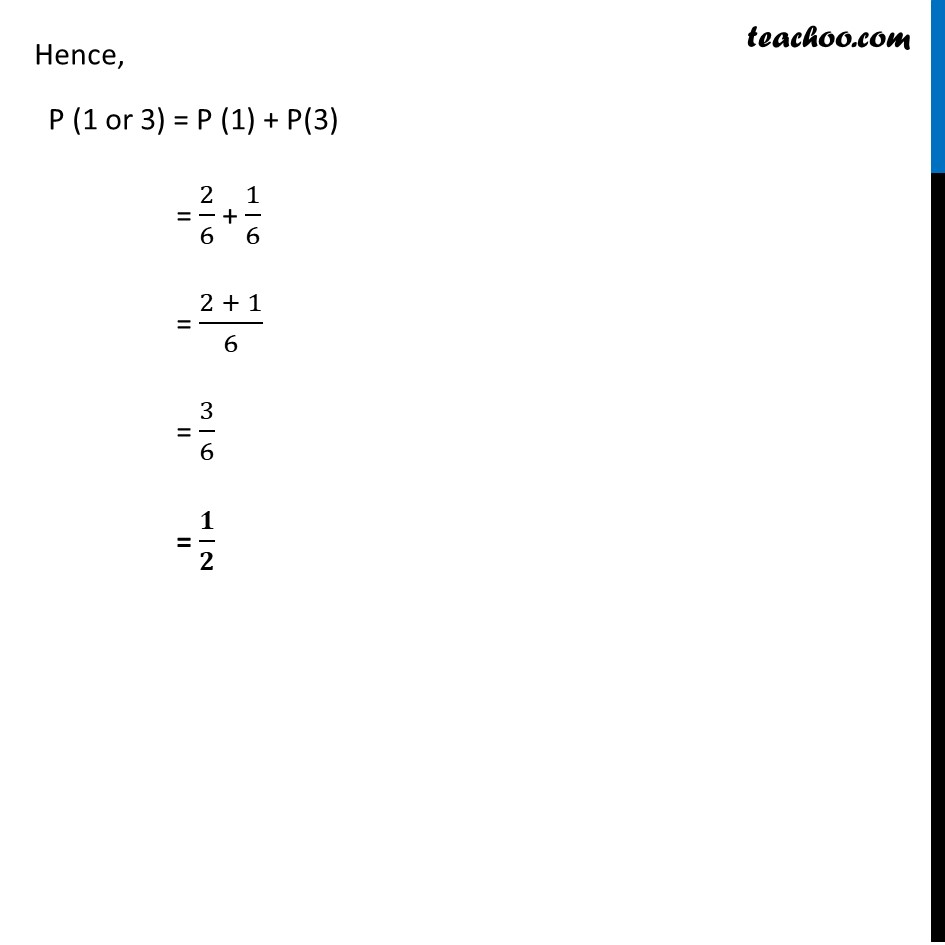
Misc 3 A die has two faces each with number ‘1’, three faces each with number ‘2’ and one face with number ‘3’. If die is rolled once, determine P(2) A normal die has 6 faces 1, 2, 3, 4, 5, 6 But in this question die has following 6 faces 1, 1, 2, 2, 2, 3 n(S) = 6 We need to find P(2) There are 3 three 2’s Hence, n(2) = 3 So, P(2) = 𝑛(2)/(𝑛(𝑆)) = 3/6 = 𝟏/𝟐 Misc 3 A die has two faces each with number ‘1’, three faces each with number ‘2’ and one face with number ‘3’. If die is rolled once, determine (ii) P(1 or 3) Die has faces 1, 1, 2, 2, 2, 3 Now ,P (1 or 3) = P (1) + P(3) P(1) There are two 1’s Hence , n(1) = 2 P(1) = (𝑛(1))/(𝑛(𝑆)) = 2/6 P(3) There are only one 3’s Hence , n(3) = 1 P(3) = (𝑛(3))/(𝑛(𝑆)) = 1/6 Hence, P (1 or 3) = P (1) + P(3) = 2/6 + 1/6 = (2 + 1)/6 = 3/6 = 𝟏/𝟐 Misc 3 A die has two faces each with number ‘1’, three faces each with number ‘2’ and one face with number ‘3’. If die is rolled once, determine (iii) P(not 3) P(not 3) = 1 – P(3) For P(3) There is only one 3’s Hence , n(3) = 1 P(3) = (𝑛(3))/(𝑛(𝑆)) = 1/6 Hence, P(not 3) = 1 – P(3) = 1 – 1/6 = (6 − 1)/6 = 𝟓/𝟔