Example 9 - Chapter 14 Class 11 Probability

Transcript

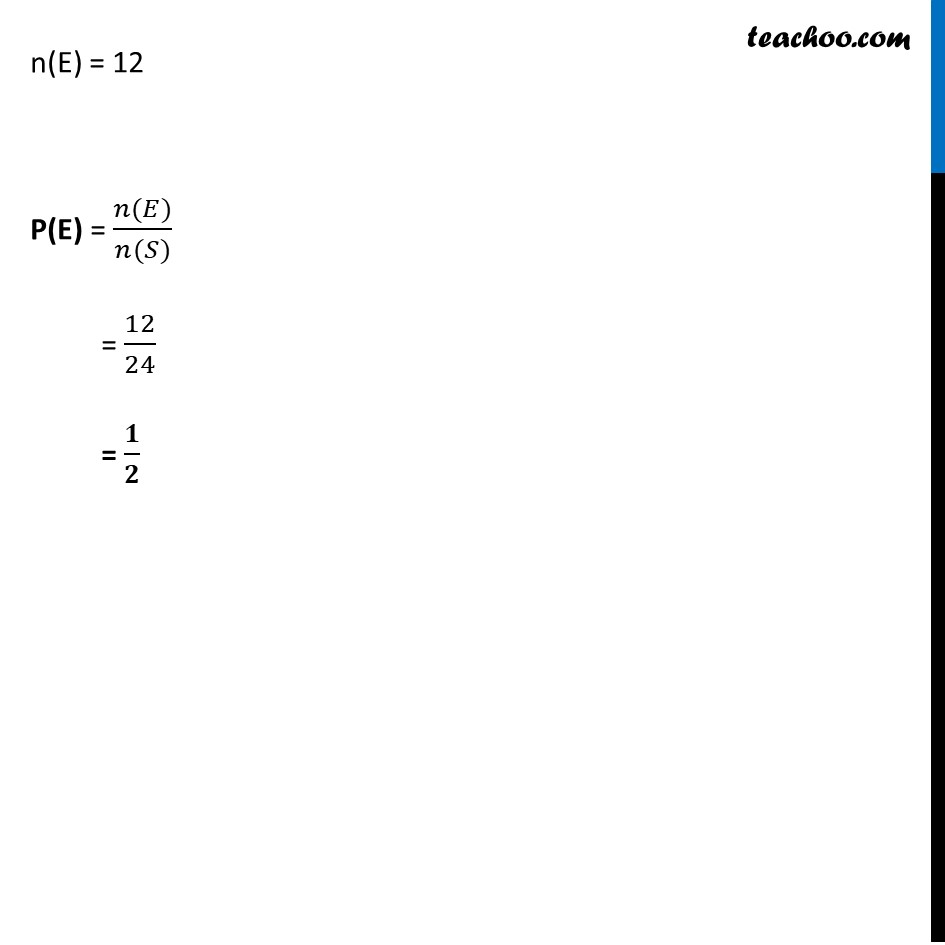
Example 9 On her vacations Veena visits four cities (A, B, C and D) in a random order. What is the probability that she visits A before B? 4 cities can be visited in any of following order S = {█("ABCD, ABDC, ACBD, ACDB, ADBC, ADCB," @" BACD, BADC, BDAC, BDCA, BCAD, BCDA," @" CABD, CADB, CBDA, CBAD, CDAB, CDBA," @" DABC, DACB, DBCA, DBAC, DCAB, DCBA" )} n(S) = 24 Let E be the event that “she visits A before B“ Hence , E = {█("ABCD, ABDC, ADBC, ACDB, ADBC, ADCB," @"CABD, CADB, CDAB, " @"DABC, DACB, DCAB," )} n(E) = 12 P(E) = (𝑛(𝐸))/(𝑛(𝑆)) = 12/24 = 𝟏/𝟐 Example 14 What is the probability that she visits (ii) A before B and B before C? S = {█("ABCD, ABDC, ACBD, ACDB, ADBC, ADCB," @" BACD, BADC, BDAC, BDCA, BCAD, BCDA," @" CABD, CADB, CBDA, CBAD, CDAB, CDBA," @" DABC, DACB, DBCA, DBAC, DCAB, DCBA" )} Let F be the event “she visits A before B and B before C “ F = {█("ABCD, ABDC, ADBC , DABC" )} So, n(F) = 4 P(F) = (𝑛(𝐹))/(𝑛(𝑆)) = 4/24 = 𝟏/𝟔 Example 14 What is the probability that she visits (iii) A first and B last? S = {█("ABCD, ABDC, ACBD, ACDB, ADBC, ADCB," @" BACD, BADC, BDAC, BDCA, BCAD, BCDA," @" CABD, CADB, CBDA, CBAD, CDAB, CDBA," @" DABC, DACB, DBCA, DBAC, DCAB, DCBA" )} Let G be the event “she visits A first and B last” G = {█("ACDB, ADCB" )} So, n(G) = 2 P(G) = (𝑛(𝐺))/(𝑛(𝑆)) = 2/24 = 𝟏/𝟏𝟐 Example 14 What is the probability that she visits (iv) A either first or second? S = {█("ABCD, ABDC, ACBD, ACDB, ADBC, ADCB," @" BACD, BADC, BDAC, BDCA, BCAD, BCDA," @" CABD, CADB, CBDA, CBAD, CDAB, CDBA," @" DABC, DACB, DBCA, DBAC, DCAB, DCBA" )} Let H be the event “she visits A either first or second” H = {█("ABCD, ABDC, ADBC, ACDB, ADBC, ADCB," @" BACD, BADC,CABD, CADB,DABC, DACB," )} So, n(H) = 12 P(H) = (𝑛(𝐻))/(𝑛(𝑆)) = 12/24 = 𝟏/𝟐 Example 14 What is the probability that she visits (v) A just before B? S = {█("ABCD, ABDC, ACBD, ACDB, ADBC, ADCB," @" BACD, BADC, BDAC, BDCA, BCAD, BCDA," @" CABD, CADB, CBDA, CBAD, CDAB, CDBA," @" DABC, DACB, DBCA, DBAC, DCAB, DCBA" )} Let I be the event “she visits A just before B” I = {█("ABCD, ABDC, CABD, CDAB, DABC, DCAB," )} So, n(I) = 6 P(I) = (𝑛(𝐼))/(𝑛(𝑆)) = 6/24 = 𝟏/𝟒