











Mutually Exculsive and Exhaustive Events
Mutually Exculsive and Exhaustive Events
Last updated at December 16, 2024 by Teachoo













Transcript
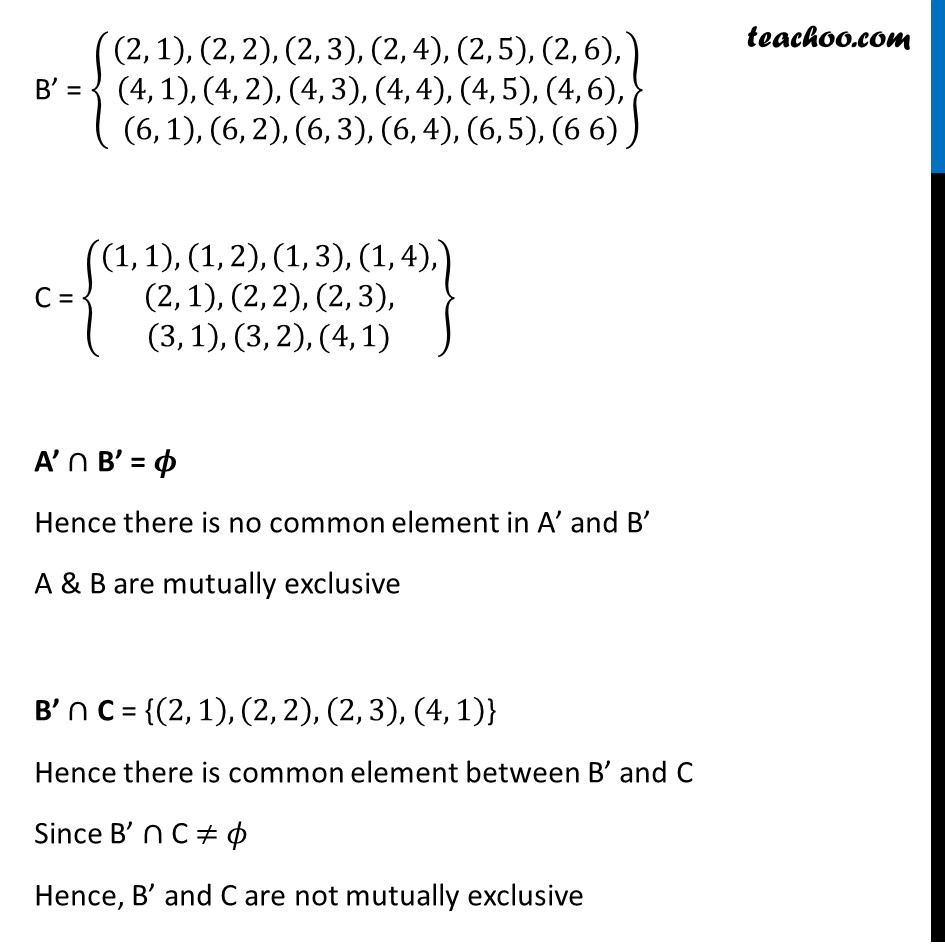
Ex 14.1, 7 Refer to question 6 above, State true or false: (give reason for your answer) A and B are mutually exclusive From 16.2 ,6 A = {█((2, 1),(2, 2),(2, 3),(2, 4),(2, 5),(2, 6)"," @" " (4, 1),(4, 2),(4, 3),(4, 4),(4, 5),(4, 6)"," @" " (6, 1),(6, 2),(6, 3),(6, 4),(6, 5),(6 6) )} B = {█((1, 1),(1, 2),(1, 3),(1, 4),(1, 5),(1, 6)"," @█((3, 1),(3, 2),(3, 3),(3, 4),(3, 5),(3, 6)@(5, 1),(5, 2),(5, 3),(5, 4),(5, 5),(5, 6) ))} A ∩ B = ϕ Since no common element in A & B So, A & B are mutually exclusive True. Ex 14.1, 7 Refer to question 6 above, State true or false: (give reason for your answer) (ii) A and B are mutually exclusive and exhaustive From 16.2 ,6 A = {█((2, 1),(2, 2),(2, 3),(2, 4),(2, 5),(2, 6)"," @" " (4, 1),(4, 2),(4, 3),(4, 4),(4, 5),(4, 6)"," @" " (6, 1),(6, 2),(6, 3),(6, 4),(6, 5),(6 6) )} B = {█((1, 1),(1, 2),(1, 3),(1, 4),(1, 5),(1, 6)"," @█((3, 1),(3, 2),(3, 3),(3, 4),(3, 5),(3, 6)@(5, 1),(5, 2),(5, 3),(5, 4),(5, 5),(5, 6) ))} A ∪ B = {█((1, 1),(1, 2),(1, 3),(1, 4),(1, 5),(1, 6)@(2, 1),(2, 2),(2, 3),(2, 4),(2, 5),(2, 6)@(3, 1),(3, 2),(3, 3),(3, 4),(3, 5),(3, 6)@(4, 1),(4, 2),(4, 3),(4, 4),(4, 5),(4, 6)@(5, 1),(5, 2),(5, 3),(5, 4),(5, 5),(5, 6)@(6, 1),(6, 2),(6, 3),(6, 4),(6, 5),(6 6),)}= S Since A ∪ B = S They are exhaustive events Also as per (i), they are mutually exclusive Hence they are mutually exclusive and exhaustive True Ex 14.1, 7 Refer to question 6 above, State true or false: (give reason for your answer) (iii) A = B’ A = getting even number on the first A = {█((2, 1),(2, 2),(2, 3),(2, 4),(2, 5),(2, 6)"," @" " (4, 1),(4, 2),(4, 3),(4, 4),(4, 5),(4, 6)"," @" " (6, 1),(6, 2),(6, 3),(6, 4),(6, 5),(6 6) )} B = getting odd no on the first die = {█((1, 1),(1, 2),(1, 3),(1, 4),(1, 5),(1, 6)"," @█((3, 1),(3, 2),(3, 3),(3, 4),(3, 5),(3, 6)@(5, 1),(5, 2),(5, 3),(5, 4),(5, 5),(5, 6) ))} B’ = getting even number on the first die = {█((2, 1),(2, 2),(2, 3),(2, 4),(2, 5),(2, 6)"," @" " (4, 1),(4, 2),(4, 3),(4, 4),(4, 5),(4, 6)"," @" " (6, 1),(6, 2),(6, 3),(6, 4),(6, 5),(6 6) )} = A Hence A = B’ True. Ex 14.1, 7 Refer to question 6 above, State true or false: (give reason for your answer) (iv) A and C are mutually exclusive A = {█((2, 1),(2, 2),(2, 3),(2, 4),(2, 5),(2, 6)"," @" " (4, 1),(4, 2),(4, 3),(4, 4),(4, 5),(4, 6)"," @" " (6, 1),(6, 2),(6, 3),(6, 4),(6, 5),(6 6) )} C = {█((1, 1), (1, 2), (1, 3), (1, 4),@(2, 1), (2, 2), (2, 3),@(3, 1), (3, 2), (4, 1))} A ∩ C = {(2, 1),(2, 2),(2, 3),(4, 1)} ≠ 𝜙 a Since there is common elements in A and C , So A & C are not mutually exclusive False. Ex 14.1, 7 Refer to question 6 above, State true or false: (give reason for your answer) (v) A and B’ are mutually exclusive A = {█((2, 1),(2, 2),(2, 3),(2, 4),(2, 5),(2, 6)"," @" " (4, 1),(4, 2),(4, 3),(4, 4),(4, 5),(4, 6)"," @" " (6, 1),(6, 2),(6, 3),(6, 4),(6, 5),(6 6) )} B’ = {█((2, 1),(2, 2),(2, 3),(2, 4),(2, 5),(2, 6)"," @" " (4, 1),(4, 2),(4, 3),(4, 4),(4, 5),(4, 6)"," @" " (6, 1),(6, 2),(6, 3),(6, 4),(6, 5),(6 6) )} A ∩ B’ = {█((2, 1),(2, 2),(2, 3),(2, 4),(2, 5),(2, 6)"," @" " (4, 1),(4, 2),(4, 3),(4, 4),(4, 5),(4, 6)"," @" " (6, 1),(6, 2),(6, 3),(6, 4),(6, 5),(6 6) )} = A Since A ∩ B’ = A ≠ ϕ Hence there is common element between A & B’ Hence A and B’ is not mutually exclusive Hence, false Ex 14.1, 7 Refer to question 6 above, State true or false: (give reason for your answer) (vi) A,’ B’, C are mutually exclusive and exhaustive. A = getting an even number on the first die A’ = getting an odd number on the first die A’ = {█((1, 1),(1, 2),(1, 3),(1, 4),(1, 5),(1, 6)"," @█((3, 1),(3, 2),(3, 3),(3, 4),(3, 5),(3, 6)@(5, 1),(5, 2),(5, 3),(5, 4),(5, 5),(5, 6) ))} B = getting an odd number on the first die B’ = getting an even number on the first die B’ = {█((2, 1),(2, 2),(2, 3),(2, 4),(2, 5),(2, 6)"," @" " (4, 1),(4, 2),(4, 3),(4, 4),(4, 5),(4, 6)"," @" " (6, 1),(6, 2),(6, 3),(6, 4),(6, 5),(6 6) )} C = {█((1, 1), (1, 2), (1, 3), (1, 4),@(2, 1), (2, 2), (2, 3),@(3, 1), (3, 2), (4, 1))} A’ ∩ B’ = 𝝓 Hence there is no common element in A’ and B’ A & B are mutually exclusive B’ ∩ C = {(2, 1),(2, 2),(2, 3), (4, 1)} Hence there is common element between B’ and C Since B’ ∩ C ≠ 𝜙 Hence, B’ and C are not mutually exclusive Since B’ & C are not mutually exclusive A,’ B’, C are not mutually exclusive and exhaustive ∴ A,’ B’, C are not mutually exclusive and exhaustive Hence, False