Misc 16 - Chapter 12 Class 11 Limits and Derivatives
Last updated at Dec. 16, 2024 by Teachoo
Miscellaneous
Misc 1 (i)
You are here
You are here
Transcript
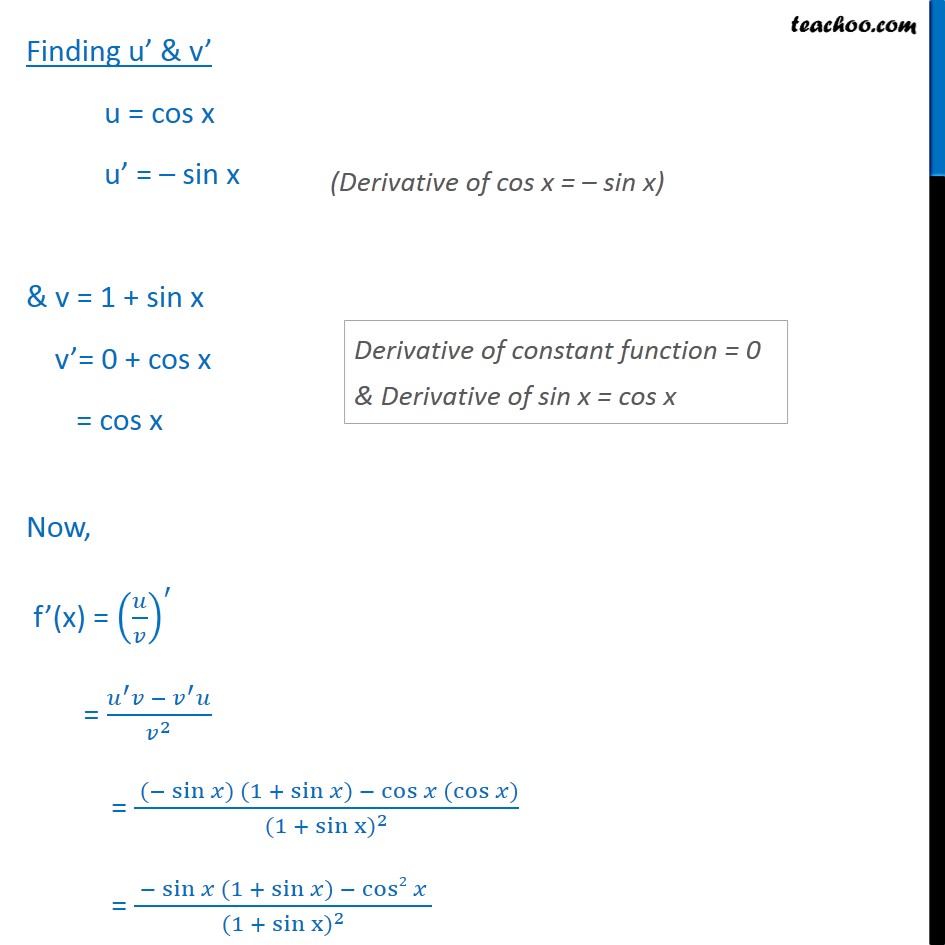
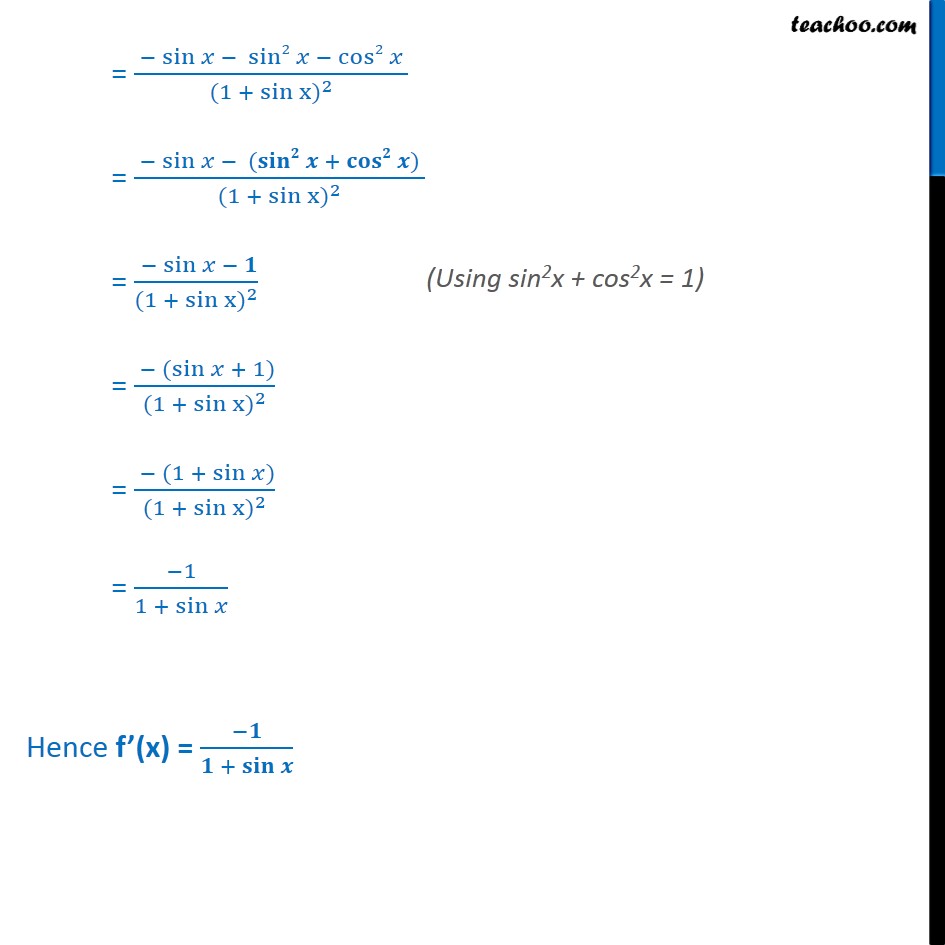
Misc 16 Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r and s are fixed non-zero constants and m and n are integers): cos x 1+ sin x Let f (x) = cos x 1 + sin x Let u = cos x & v = 1 + sin x So, f(x) = f (x) = Using quotient rule f (x) = 2 Finding u & v u = cos x u = sin x & v = 1 + sin x v = 0 + cos x = cos x Now, f (x) = = 2 = ( sin ) (1 + sin ) cos ( cos ) (1 + sin x ) 2 = sin (1 + sin ) cos2 (1 + sin x ) 2 = sin sin2 cos2 (1 + sin x ) 2 = sin ( + ) (1 + sin x ) 2 = sin (1 + sin x ) 2 = (sin + 1) (1 + sin x ) 2 = (1 + sin ) (1 + sin x ) 2 = 1 1 + sin Hence f (x) = +
