Example 22 (i) - Chapter 13 Class 11 Limits and Derivatives (Important Question)

Transcript

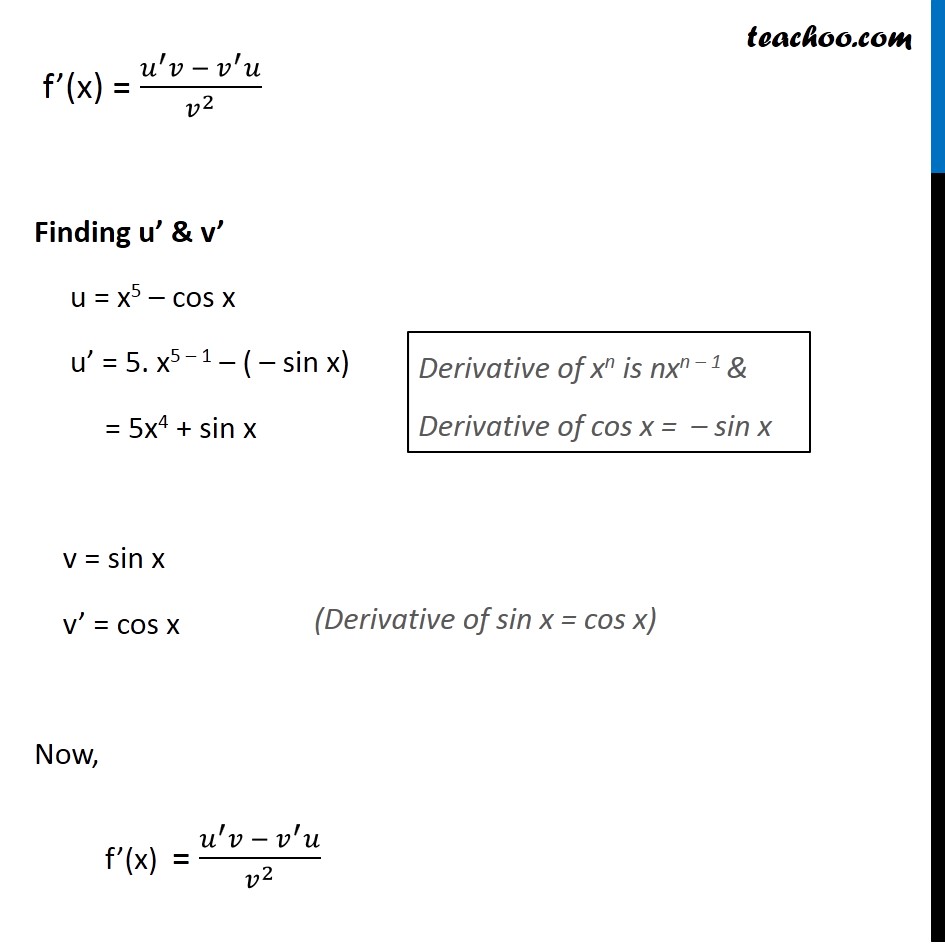
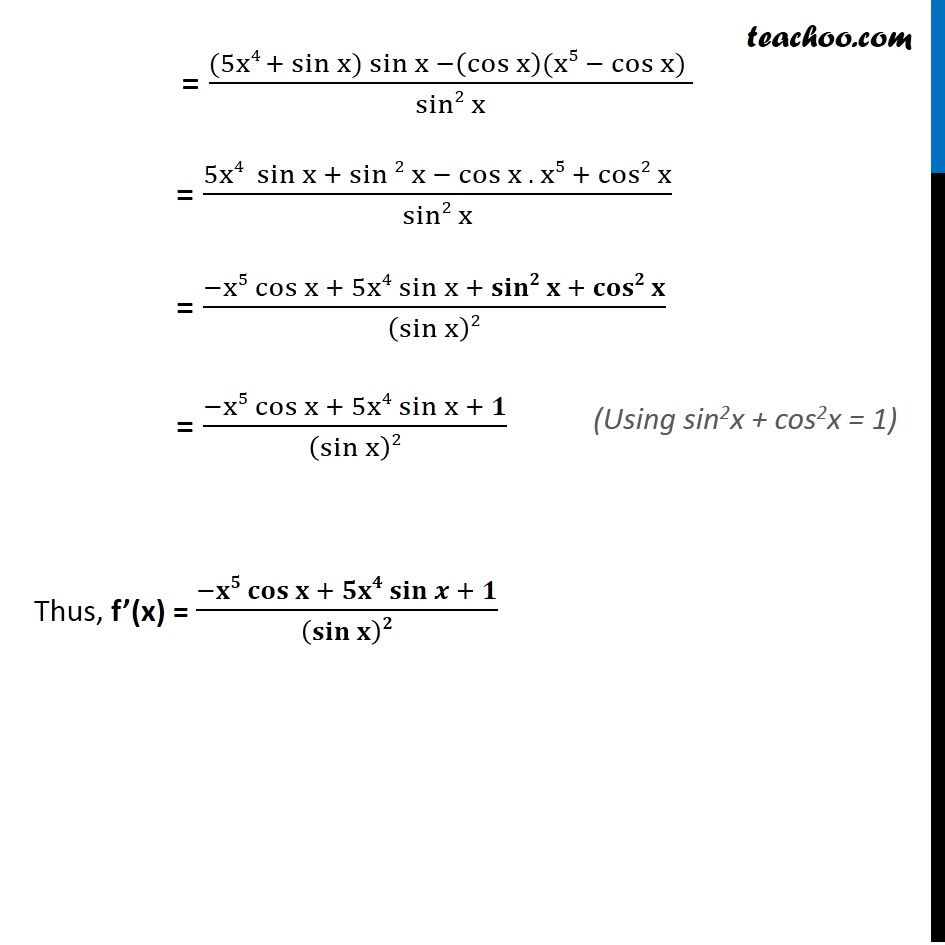
Example 22 Find the derivative of (i) (x^5 − cos⁡x)/sin⁡x Let f(x) = (x^5 − cos⁡x)/sin⁡x Let u = x5 – cos x & v = sin x So, f(x) = (𝑢/𝑣) ∴ f’(x) = (𝑢/𝑣)^′ Using quotient rule f’(x) = (𝑢^′ 𝑣 −〖 𝑣〗^′ 𝑢)/𝑣^2 Finding u’ & v’ u = x5 – cos x u’ = 5. x5 – 1 – ( – sin x) = 5x4 + sin x v = sin x v’ = cos x Now, f’(x) = (𝑢^′ 𝑣 −〖 𝑣〗^′ 𝑢)/𝑣^2 Derivative of xn is nxn – 1 & Derivative of cos x = – sin x (Derivative of sin x = cos x) = ((5x4 + sin⁡〖x) sin x −(cos x)(x5 − cos x) 〗)/sin2⁡x = (5x4 sin⁡〖x + sin 2⁡x − cos x . x5 + cos2 x〗)/(sin2 x) = (−x5 cos⁡〖x + 5x4 sin⁡x + 𝐬𝐢𝐧𝟐 𝐱 + 𝐜𝐨𝐬𝟐 𝐱〗)/(sin⁡x )2 = (−x5 cos⁡〖x + 5x4 sin⁡x + 𝟏〗)/(sin⁡x )2 Thus, f’(x) = (−𝐱𝟓 𝐜𝐨𝐬⁡〖𝐱 + 𝟓𝐱𝟒 𝐬𝐢𝐧⁡𝒙 + 𝟏〗)/(𝐬𝐢𝐧⁡𝐱 )𝟐 (Using sin2x + cos2x = 1)