

Derivatives by formula - x^n formula
Derivatives by formula - x^n formula
Last updated at December 16, 2024 by Teachoo



Transcript
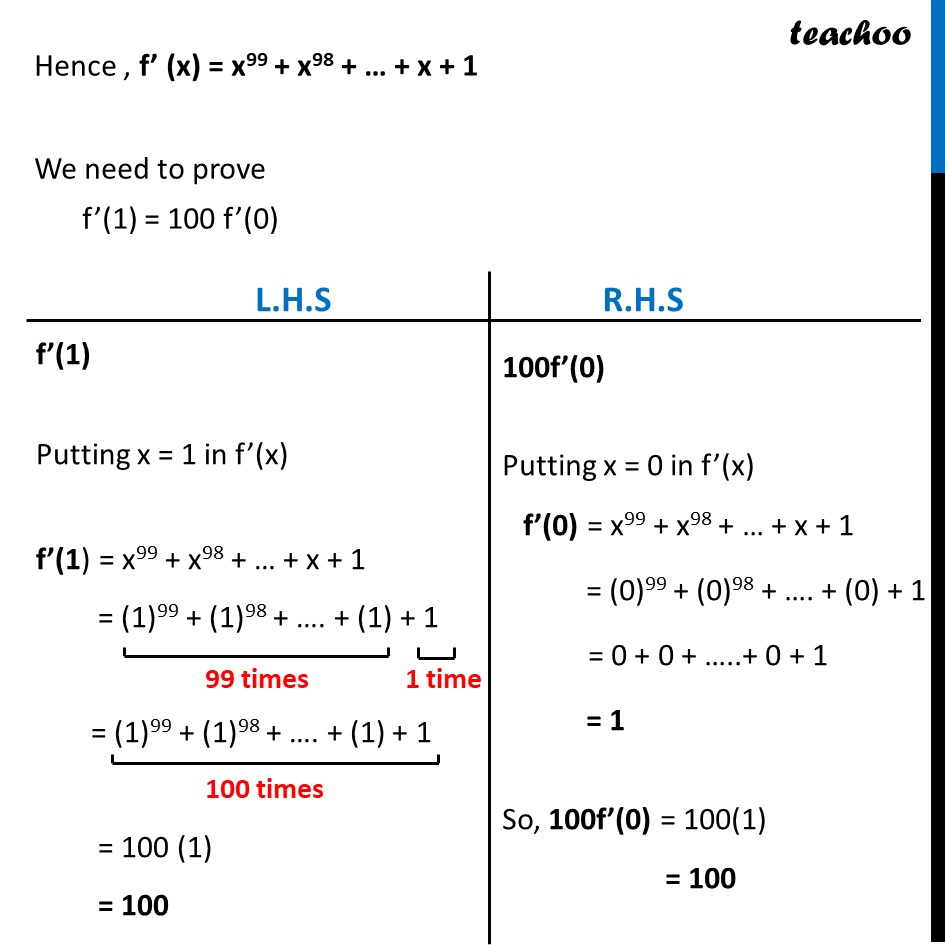
Ex 12.2, 5 For the function f(x) = x100100 + x99100 +….+ x22 + x + 1. Prove that f’(1) = 100 f’(0) We have f (x) = 𝑥100100 + 𝑥9999 + …… + 𝑥22 + x + 1 f’ (x) = 1100 x100 + 199 x99 + …… + 12 x2 + x1 + 1′ f’ (x) = 1100 × 100x100 – 1 + 199 × 99x99 – 1 + … + 12 × 2x2 – 1 + 1.x1-1 + 0 = 100100 x99 + 9999 x98 + …+ 22 x1 + x0 + 0 = x99 + x98 + …..+ x + 1 + 0 = x99 + x98 + … + x + 1 Hence , f’ (x) = x99 + x98 + … + x + 1 We need to prove f’(1) = 100 f’(0) Hence R.H.S = L.H.S Hence proved