You are here
Ex 12.1, 8 - Chapter 12 Class 11 Limits and Derivatives
Last updated at Dec. 16, 2024 by Teachoo
Chapter 12 Class 11 Limits and Derivatives
Concept wise
- Limits - Definition
-
Limits - 0/0 form
- Limits - x^n formula
- Limits - Of Trignometric functions
- Limits - Limit exists
- Derivatives by 1st principle - At a point
- Derivatives by 1st principle - At a general point
- Derivatives by formula - x^n formula
- Derivatives by formula - sin & cos
- Derivatives by formula - other trignometric
Transcript
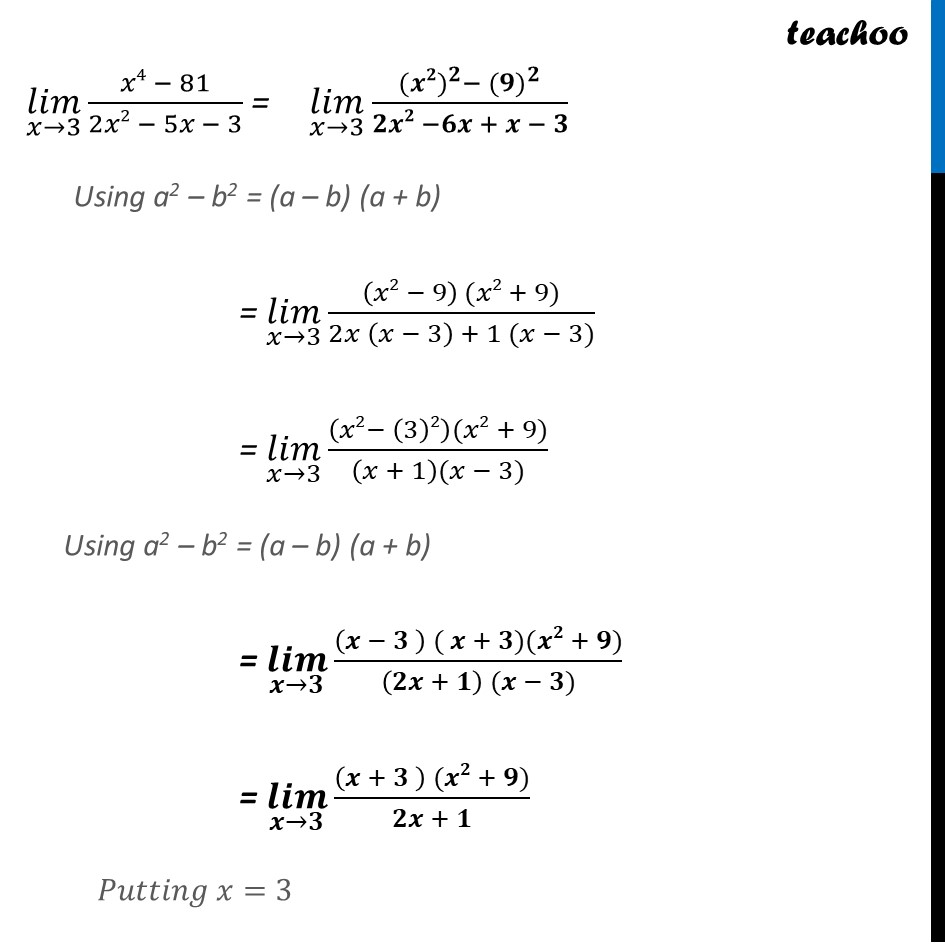
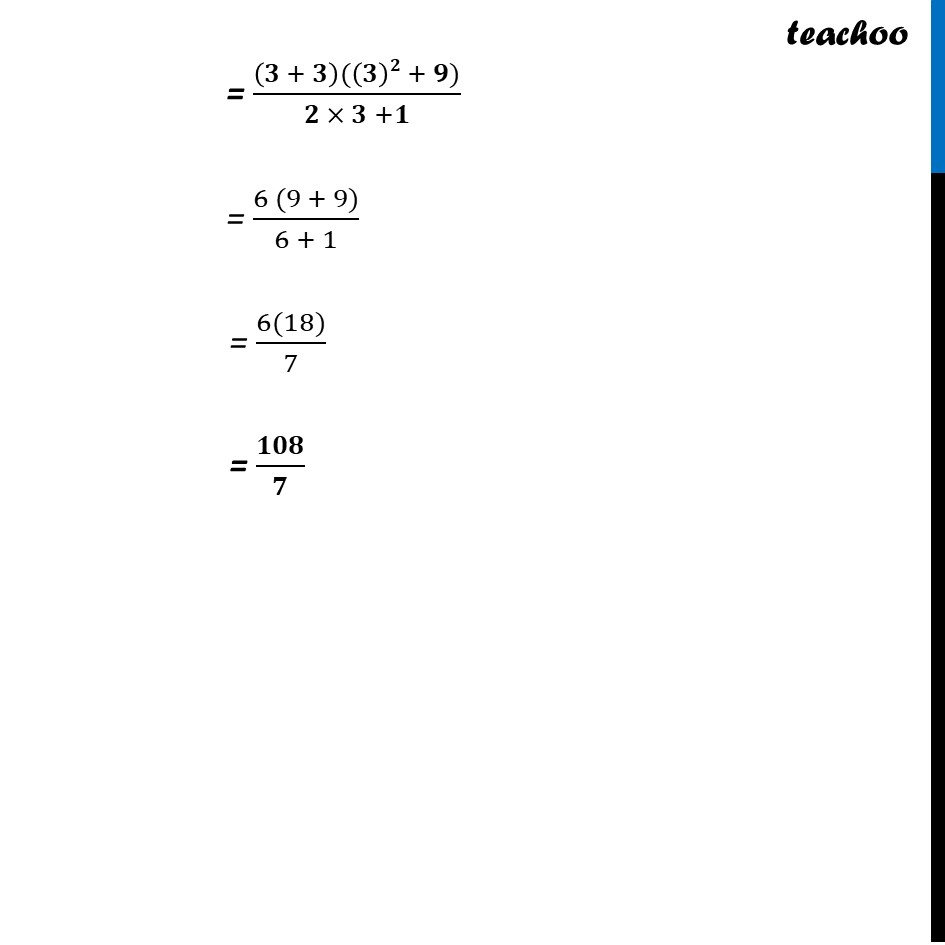
Ex 12.1, 8 Evaluate the Given limit: lim┬(x→3) (x4 −81)/(2x2 −5x−3) lim┬(x→3) (x4 − 81)/(2x2 − 5x − 3) Putting x = 3 = ((3)4 − 81)/(2 (3)2 − 5 (3) − 3) = (81 − 81)/(18 − 15 − 3) = 0/0 Since it is a 0/0 form we simplify as lim┬(x→3) (x4 − 81)/(2x2 − 5x − 3) = lim┬(x→3) (〖(𝑥2)〗^2 − 〖(9)〗^2)/(2x2 − 6x + x − 3) = lim┬(x→3) ((x2 − 9) (x2 + 9))/(2x (x − 3) + 1 (x − 3)) = lim┬(x→3) ((𝑥2− (3)2) (𝑥2 + 9))/((x + 1)(𝑥 − 3)) = lim┬(x→3) ((𝑥 − 3) (𝑥 + 3)(𝑥2 + 9))/((2𝑥 + 1) (𝑥 − 3)) = (𝐥𝐢𝐦)┬(𝐱→𝟑) ((𝒙 + 𝟑) (𝒙𝟐 + 𝟗))/(𝟐𝒙 + 𝟏) (Using a2 – b2 = (a – b) (a + b)) (Using a2 – b2 = (a – b) (a + b)) 𝑃𝑢𝑡𝑡𝑖𝑛𝑔 𝑥=3 = ((3 + 3)((3)2 + 9))/(2 ×3 +1) = (6 (9 + 9))/(6 + 1) = (6(18))/7 = 𝟏𝟎𝟖/𝟕
