Question 5 - Chapter 12 Class 11 Introduction to Three Dimensional Geometry (Important Question)

Transcript

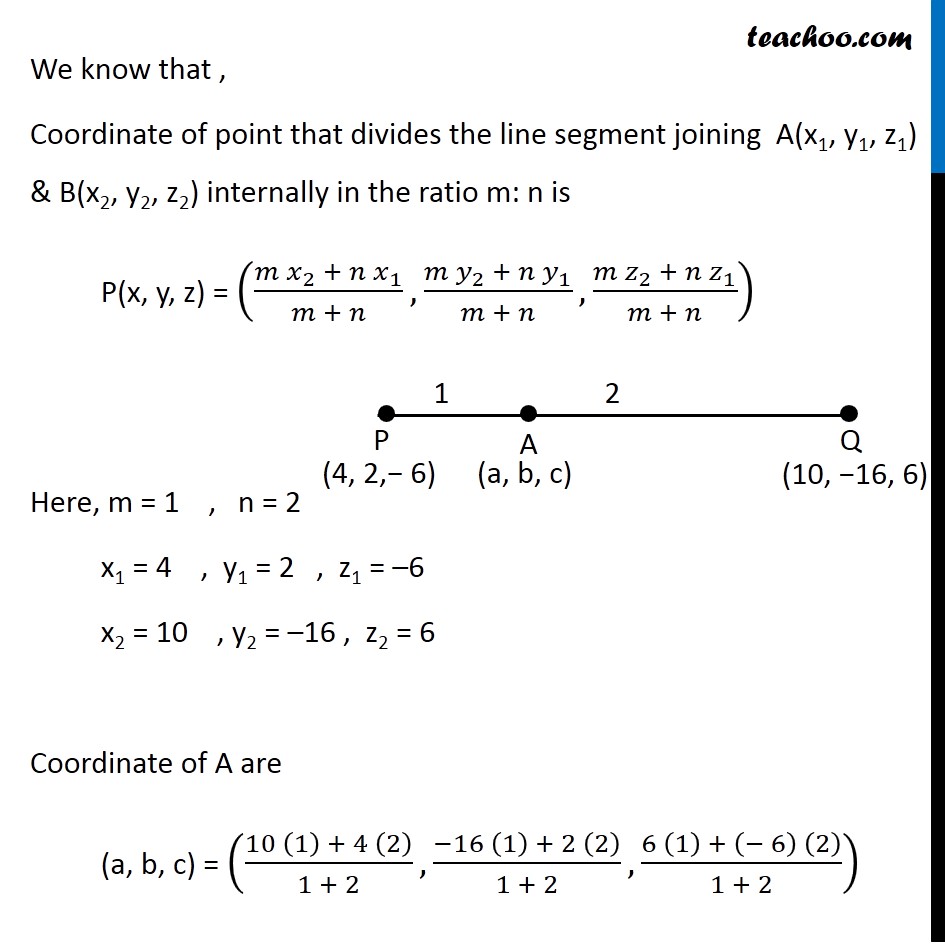
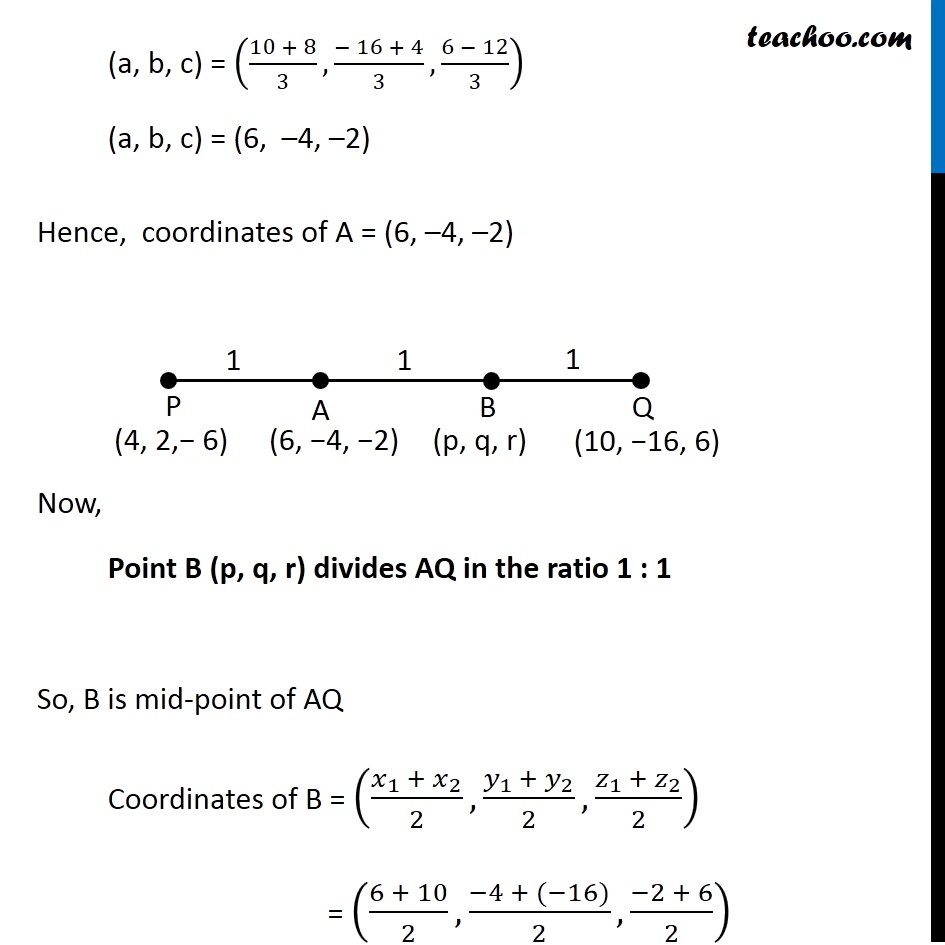
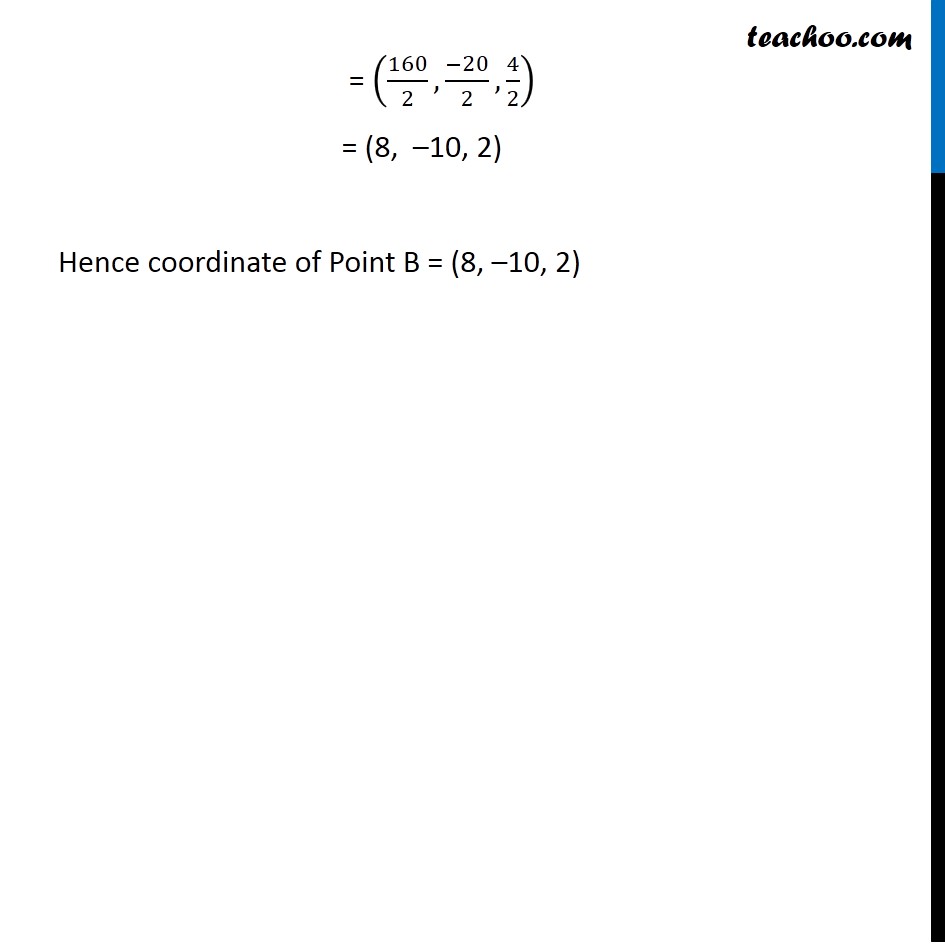
Question 5 Find the coordinates of the points which trisect the line segment joining the points P (4, 2, –6) and Q (10, –16, 6). Let Point A (a, b, c) & point B (p, q, r) trisect the line segment PQ i.e. PA = AB = BC Point A divides PQ in the ratio of 1 : 2 We know that , Coordinate of point that divides the line segment joining A(x1, y1, z1) & B(x2, y2, z2) internally in the ratio m: n is P(x, y, z) = ((〖𝑚 𝑥〗_2 +〖 𝑛 𝑥〗_1)/(𝑚 + 𝑛),(〖𝑚 𝑦〗_2 +〖 𝑛 𝑦〗_1)/(𝑚 + 𝑛),(〖𝑚 𝑧〗_2 +〖 𝑛 𝑧〗_1)/(𝑚 + 𝑛)) Here, m = 1 , n = 2 x1 = 4 , y1 = 2 , z1 = –6 x2 = 10 , y2 = –16 , z2 = 6 Coordinate of A are (a, b, c) = ((10 (1) + 4 (2))/(1 + 2),(−16 (1) + 2 (2))/(1 + 2),(6 (1) + (− 6) (2))/(1 + 2)) (a, b, c) = ((10 + 8)/3,(− 16 + 4)/3,(6 − 12)/3) (a, b, c) = (6, –4, –2) Hence, coordinates of A = (6, –4, –2) Now, Point B (p, q, r) divides AQ in the ratio 1 : 1 So, B is mid-point of AQ Coordinates of B = ((𝑥_(1 )+ 𝑥_2)/2,(𝑦_(1 )+ 𝑦_2)/2,(𝑧_(1 )+ 𝑧_2)/2) = ((6 + 10)/2,(−4 + (−16))/2,(−2 + 6)/2) = (160/2,(−20)/2,4/2) = (8, –10, 2) Hence coordinate of Point B = (8, –10, 2)