Example 10 - Chapter 11 Class 11 Conic Sections (Important Question)

Transcript

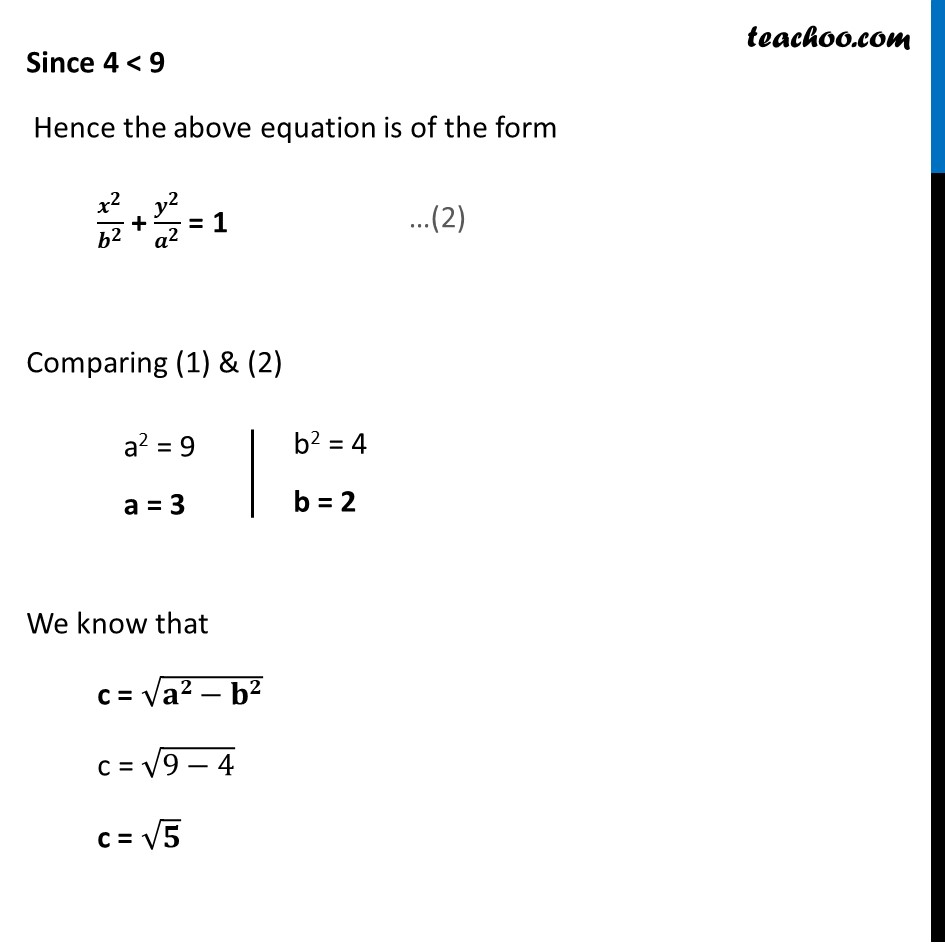
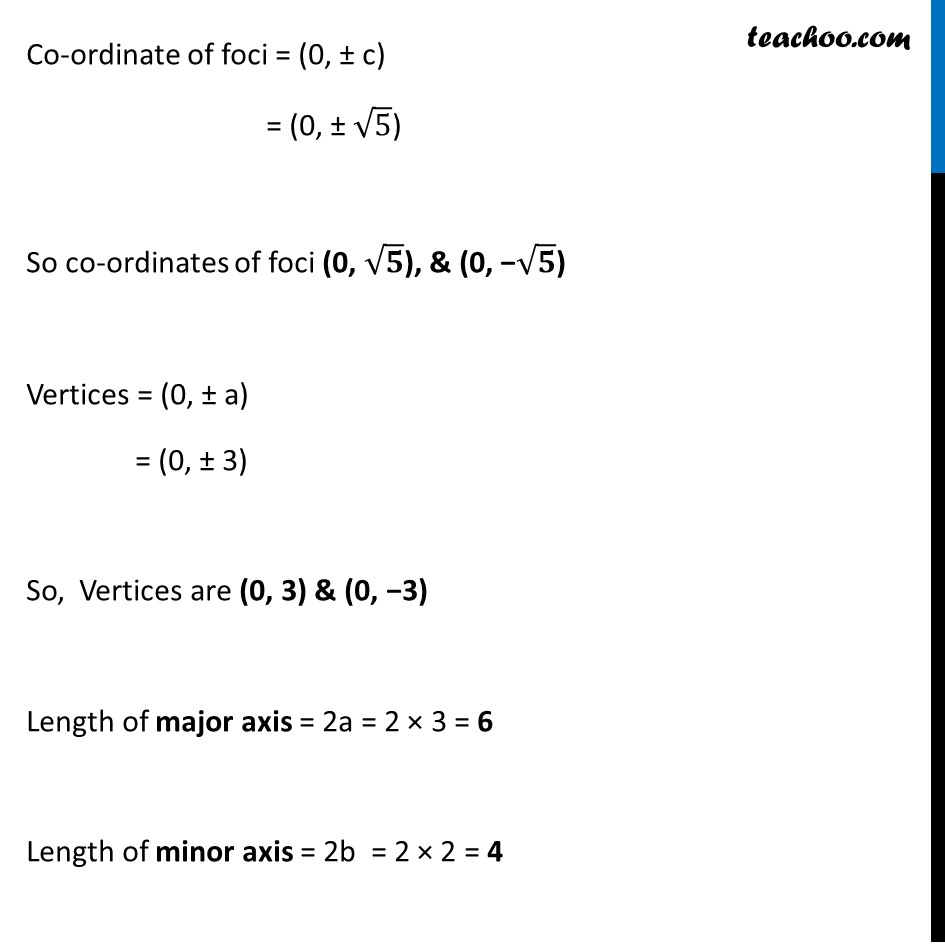
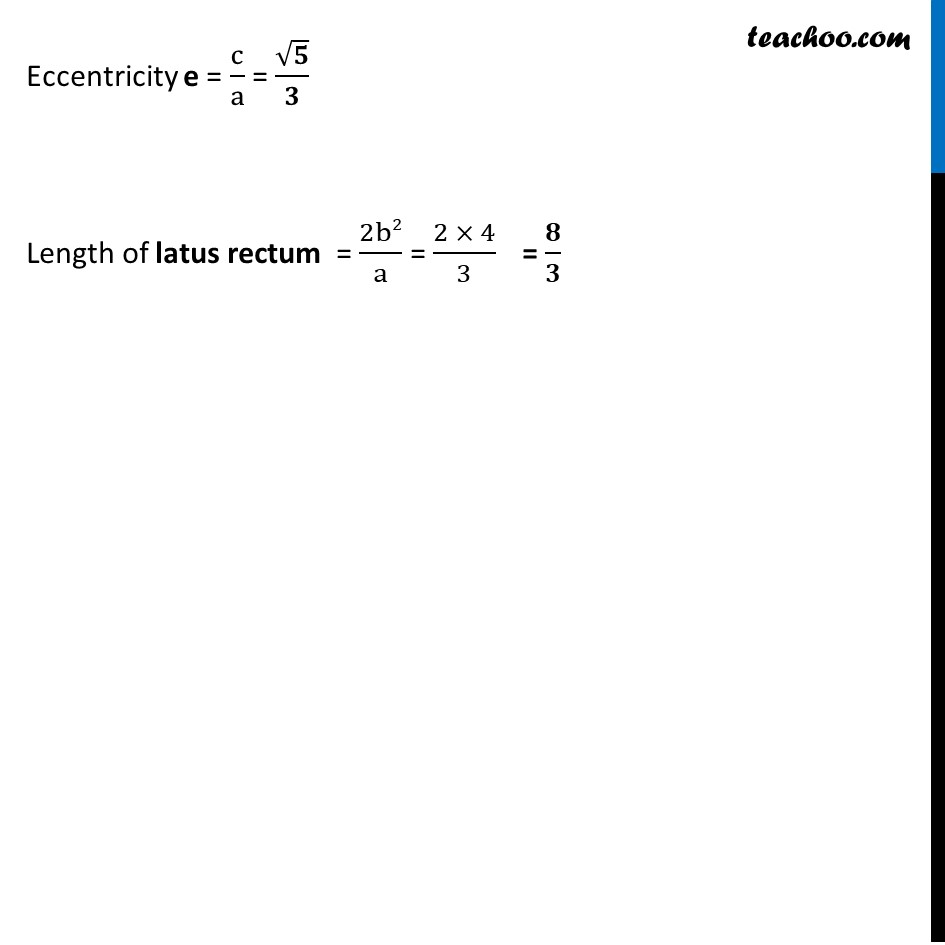
Example 10 Find the coordinates of the foci, the vertices, the lengths of major and minor axes and the eccentricity of the ellipse 9x2 + 4y2 = 36. Given 9x2 + 4y2 = 36 Dividing whole equation by 36 (9𝑥^2 + 4𝑦^2)/36 = 36/36 9/36 x2 + (4𝑦^2)/36 = 1 𝑥^2/4 + 𝑦^2/9 = 1 Since 4 < 9 Hence the above equation is of the form 𝑥^2/𝑏^2 + 𝑦^2/𝑎^2 = 1 Comparing (1) & (2) We know that c = √(a2−b2) c = √(9−4) c = √𝟓 Co-ordinate of foci = (0, ± c) = (0, ± √5) So co-ordinates of foci (0, √𝟓), & (0, −√𝟓) Vertices = (0, ± a) = (0, ± 3) So, Vertices are (0, 3) & (0, −3) Length of major axis = 2a = 2 × 3 = 6 Length of minor axis = 2b = 2 × 2 = 4 Eccentricity e = c/a = √𝟓/𝟑 Length of latus rectum = 2b2/a = (2 × 4)/3 = 𝟖/𝟑