Ex 10.3, 10 - Chapter 10 Class 11 Conic Sections

Last updated at Dec. 16, 2024 by

Slide28.JPG

Slide29.JPG
Slide30.JPG

Go Ad-free

Transcript

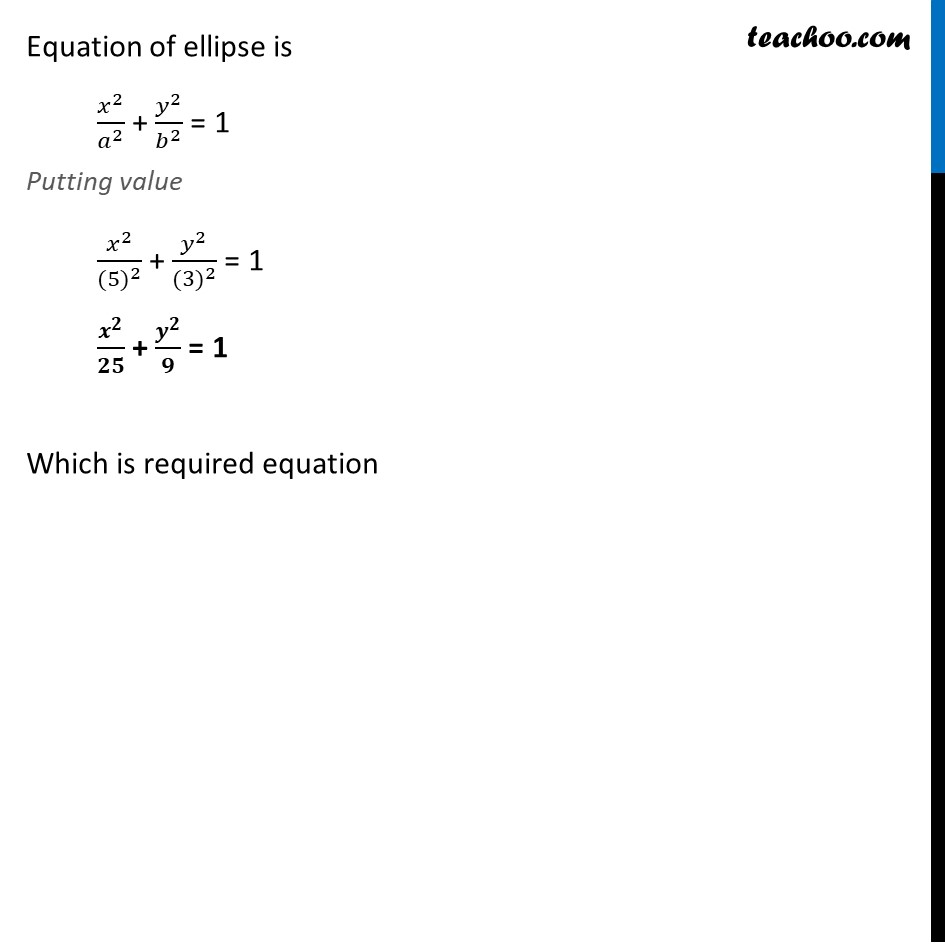
Ex 10.3, 10 Find the equation for the ellipse that satisfies the given conditions: Vertices (±5, 0), foci (±4, 0) Given Vertices (±5, 0) Since the vertices are of form (± a, 0) Hence, Major axis is along x-axis and equation of ellipse is ﷐﷐𝑥﷮2﷯﷮﷐𝑎﷮2﷯﷯ + ﷐﷐𝑦﷮2﷯﷮﷐𝑏﷮2﷯﷯ = 1 From (1) & (2) a = 5 Also given coordinate of foci = (±4, 0) We know that foci = (± c, 0) So c = 4 We know that c2 = a2 − b2 (4) 2 = (5) 2 − b2 b2 = (5) 2 − (4) 2 b2 = 25 − 16 b2 = 9 b = ﷐﷮9﷯ b = 3 Hence b = 3 Equation of ellipse is ﷐﷐𝑥﷮2﷯﷮﷐𝑎﷮2﷯﷯ + ﷐﷐𝑦﷮2﷯﷮﷐𝑏﷮2﷯﷯ = 1 Putting value ﷐﷐𝑥﷮2﷯﷮﷐(5)﷮2﷯﷯ + ﷐﷐𝑦﷮2﷯﷮﷐(3)﷮2﷯﷯ = 1 ﷐﷐𝑥﷮2﷯﷮25﷯ + ﷐﷐𝑦﷮2﷯﷮9﷯ = 1 Which is required equation

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo