
Ellipse - Defination
Last updated at December 16, 2024 by Teachoo



Transcript
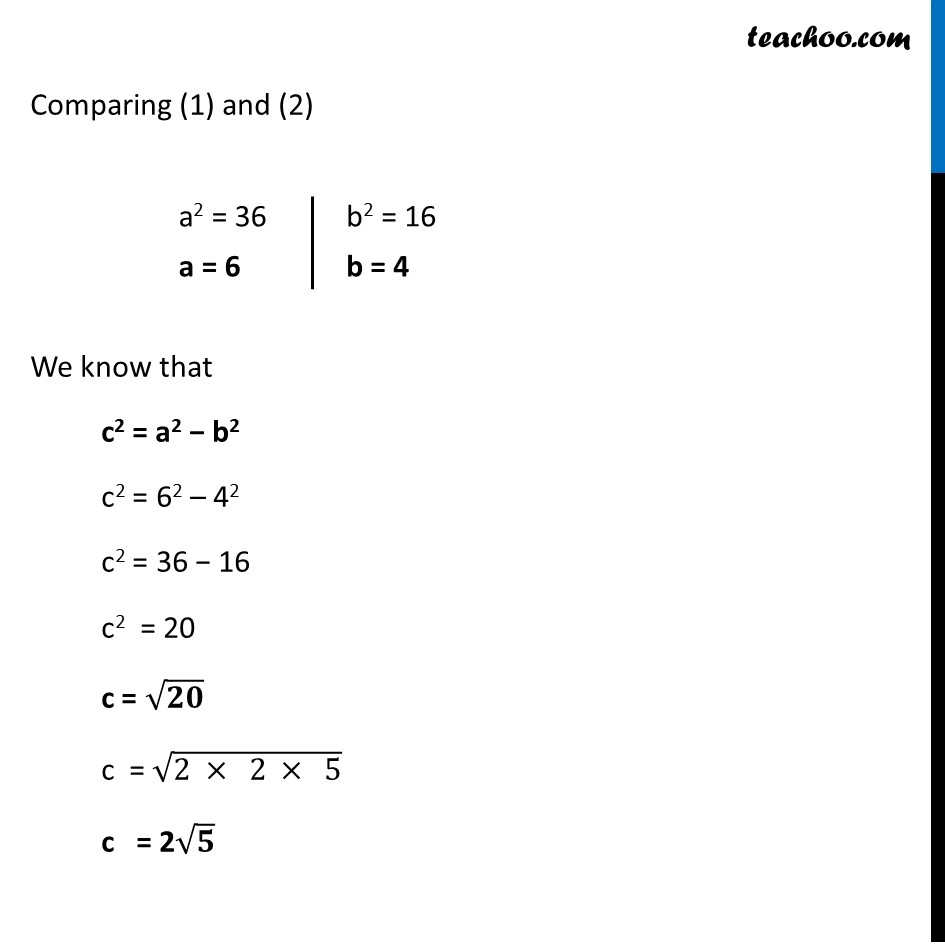
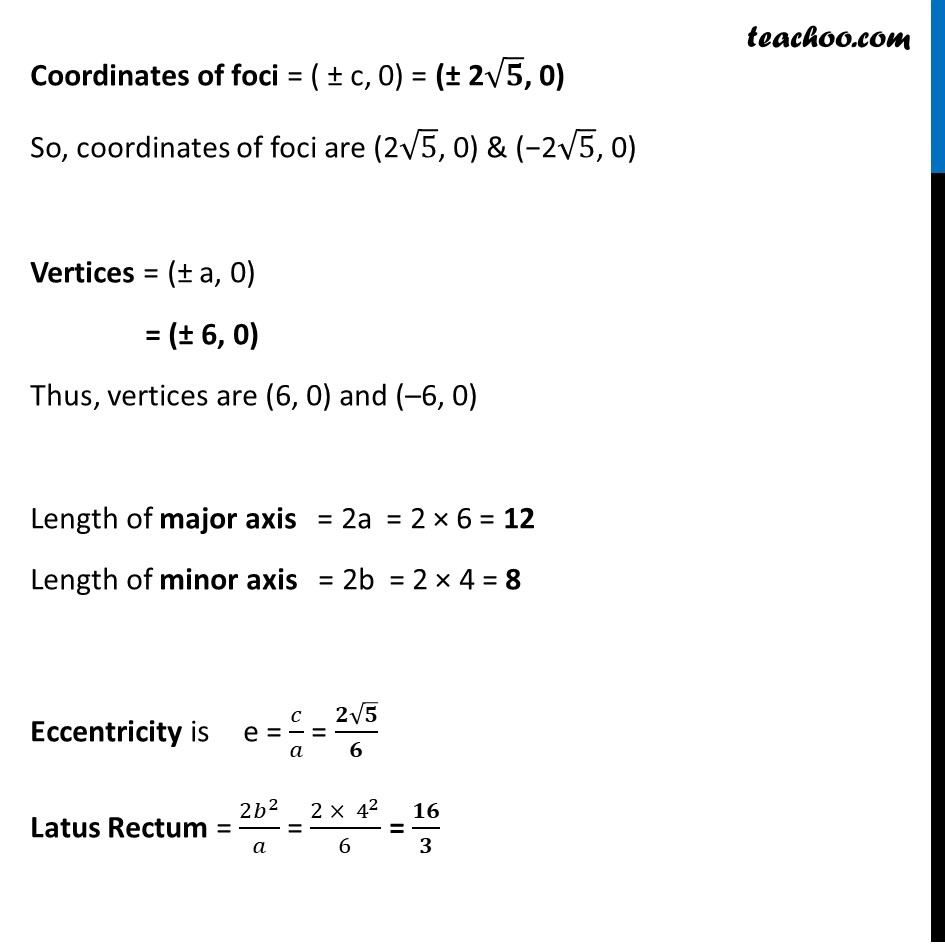
Ex 10.3, 1 Find the coordinates of the foci, the vertices, the length of major axis, the minor axis, the eccentricity and the length of the latus rectum of the ellipse x236 + y216 = 1 The given equation is 𝑥236 + 𝑦216 = 1 Since 36 > 16, The above equation is of the form 𝑥2𝑎2 + 𝑦2𝑏2 = 1 Comparing (1) and (2) We know that c2 = a2 − b2 c2 = 62 – 42 c2 = 36 − 16 c2 = 20 c = 20 c = 2 × 2 × 5 c = 2𝟓 Coordinates of foci = ( ± c, 0) = (± 25, 0) So, coordinates of foci are (25, 0) & (−25, 0) Vertices = (± a, 0) = (± 6, 0) Thus vertices are (6, 0) and (–6, 0) Length of major axis = 2a = 2 × 6 = 12 Length of minor axis = 2b = 2 × 4 = 8 Eccentricity is e = 𝑐𝑎 = 256 Latus Rectum = 2𝑏2𝑎 = 2 × 426 = 163