Misc 12 - Chapter 9 Class 11 Straight Lines

Transcript

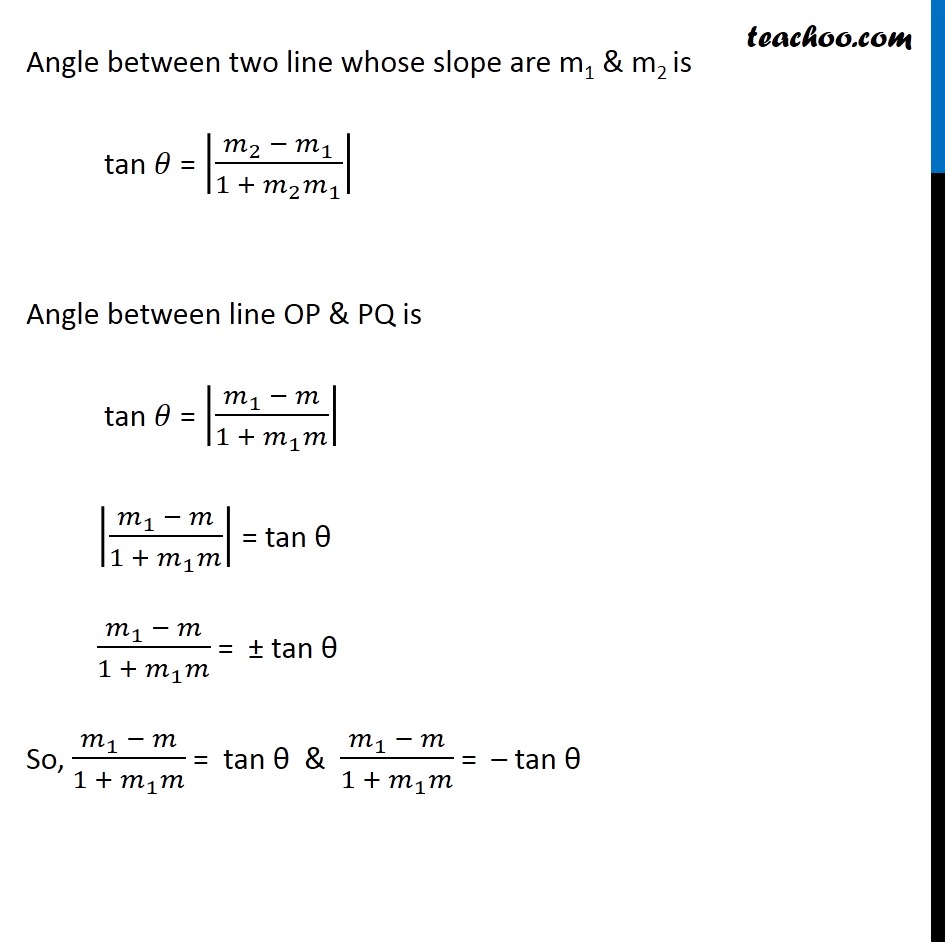
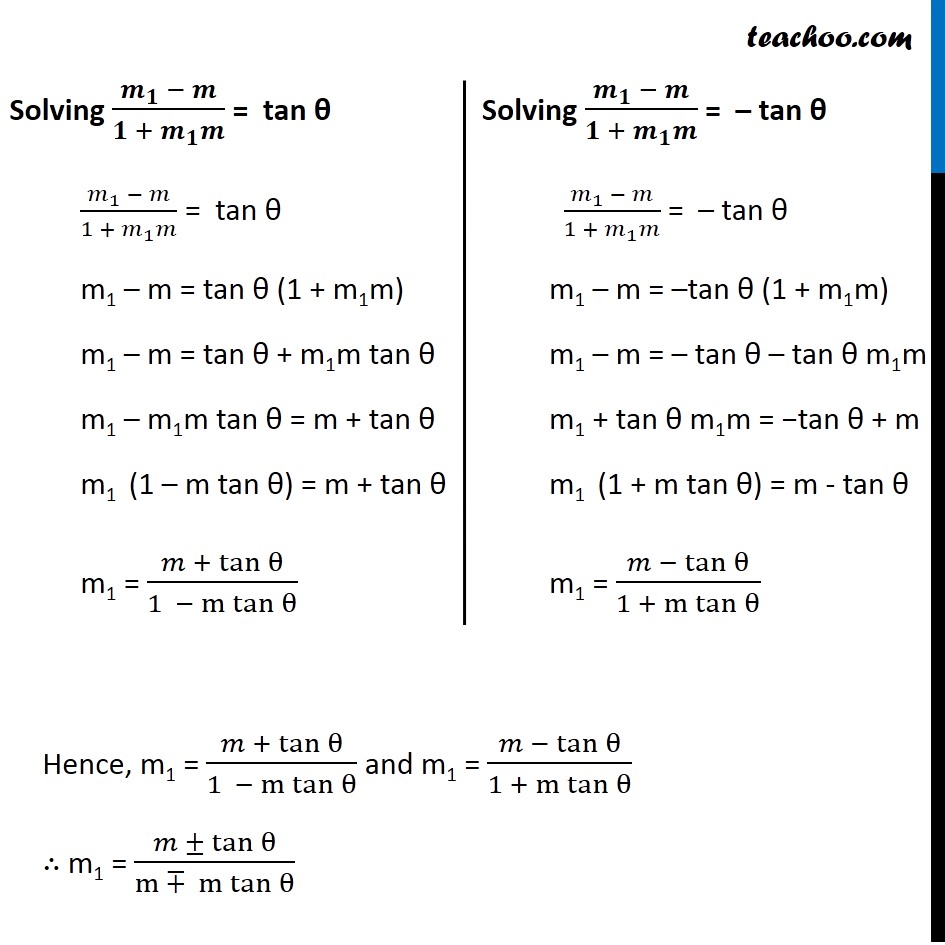
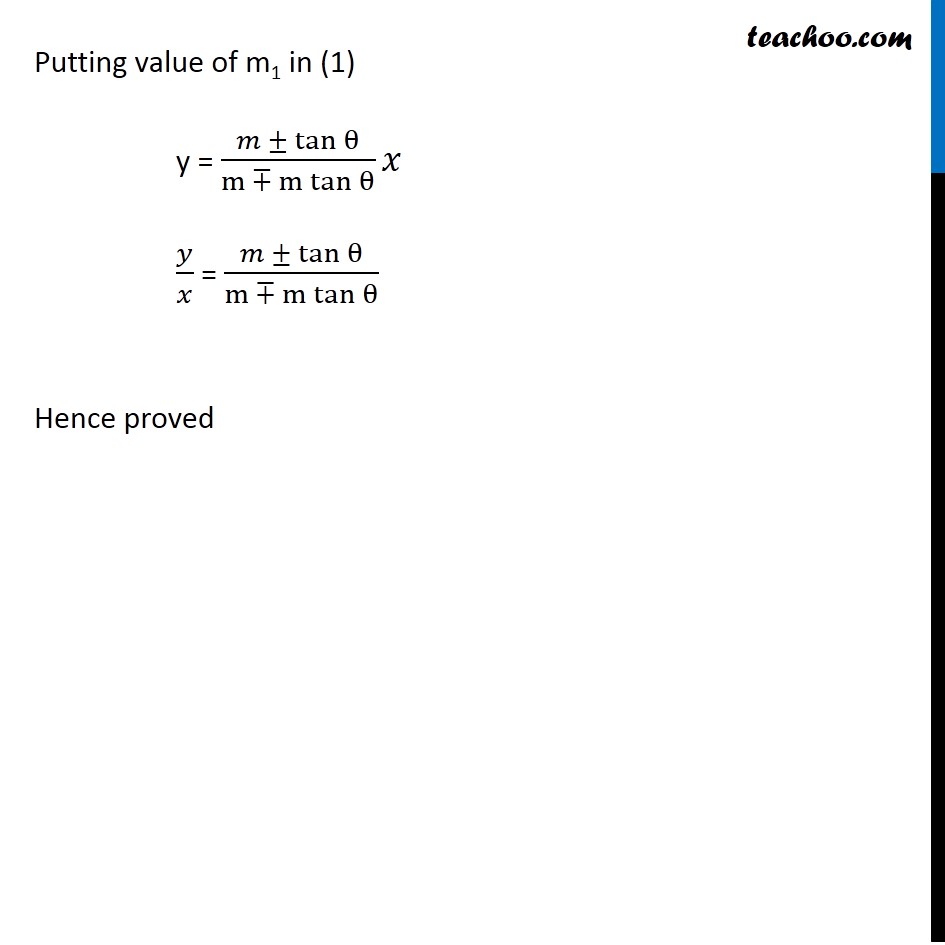
Misc 13 Show that the equation of the line passing through the origin and making an angle θ with the line y = mx + c is 𝑦/𝑥 = (𝑚 ± 𝑡𝑎𝑛𝜃)/(1 ∓ 𝑚 𝑡𝑎𝑛𝜃) . Let OP be the line passing through origin Let PQ be the line y = mx + c Whose slope is m and makes an angle θ with line OP We need to show equation line OP is 𝑦/𝑥 = (𝑚 ± 𝑡𝑎𝑛𝜃)/(1 ∓ 𝑚 𝑡𝑎𝑛𝜃) We know that equation of line passing through point (x1, y1) & having slope m is (y – y1) = m(x – x1) Let m1 be the slope of line OP Equation of a line OP passing through origin (0, 0) with slope m1 (y – 0) = m1(x – 0) y = m1x Now we need to find slope (i.e. m1) of line OP It is given that line OP makes an angle θ with line PQ y = mx + c We know that equation of line passing through point (x1, y1) & having slope m is (y – y1) = m(x – x1) Let m1 be the slope of line OP Equation of a line OP passing through origin (0, 0) with slope m1 (y – 0) = m1(x – 0) y = m1x Now we need to find slope (i.e. m1) of line OP It is given that line OP makes an angle θ with line PQ y = mx + c Angle between two line whose slope are m1 & m2 is tan 𝜃 = |(𝑚_2 − 𝑚_1)/(1 + 𝑚_2 𝑚_1 )| Angle between line OP & PQ is tan 𝜃 = |(𝑚_1 − 𝑚)/(1 + 𝑚_1 𝑚)| |(𝑚_1 − 𝑚)/(1 + 𝑚_1 𝑚)| = tan θ (𝑚_1 − 𝑚)/(1 + 𝑚_1 𝑚) = ± tan θ So, (𝑚_1 − 𝑚)/(1 + 𝑚_1 𝑚) = tan θ & (𝑚_1 − 𝑚)/(1 + 𝑚_1 𝑚) = – tan θ Solving (𝒎_𝟏 − 𝒎)/(𝟏 + 𝒎_𝟏 𝒎) = tan θ (𝑚_1 − 𝑚)/(1 + 𝑚_1 𝑚) = tan θ m1 – m = tan θ (1 + m1m) m1 – m = tan θ + m1m tan θ m1 – m1m tan θ = m + tan θ m1 (1 – m tan θ) = m + tan θ m1 = (𝑚 + tan⁡θ)/(1 − m tan⁡θ ) Solving (𝒎_𝟏 − 𝒎)/(𝟏 + 𝒎_𝟏 𝒎) = – tan θ (𝑚_1 − 𝑚)/(1 + 𝑚_1 𝑚) = – tan θ m1 – m = –tan θ (1 + m1m) m1 – m = – tan θ – tan θ m1m m1 + tan θ m1m = −tan θ + m m1 (1 + m tan θ) = m - tan θ m1 = (𝑚 − tan⁡θ)/(1 + m tan⁡θ ) Putting value of m1 in (1) y = (𝑚 ± tan⁡θ)/(m ∓ m tan⁡θ ) 𝑥 𝑦/𝑥 = (𝑚 ± tan⁡θ)/(m ∓ m tan⁡θ ) Hence proved