You are here
Misc 11 - Chapter 9 Class 11 Straight Lines
Last updated at Dec. 16, 2024 by Teachoo
Chapter 9 Class 11 Straight Lines
Concept wise
- Cordinate geometry questions
- Slope - Finding slope
- Slope - Prependicularity
- Angle between two lines by Slope
- Collinearity of 3 points by sliope
- Point Slope form
-
Slope-Intercept form
- Intercept form
- Normal form
- Given Statement
- Two lines // or/and prependicular
- Angle between two lines
- Distance - Between two parallel lines
- Distance of a point from a line
- Distance of a point from a line along a line
- Other Type of questions - Area of Triangle formed
- Other Type of questions - 3 lines Concurrent
- Other Type of questions - Image
- Other Type of questions - Mix
Transcript
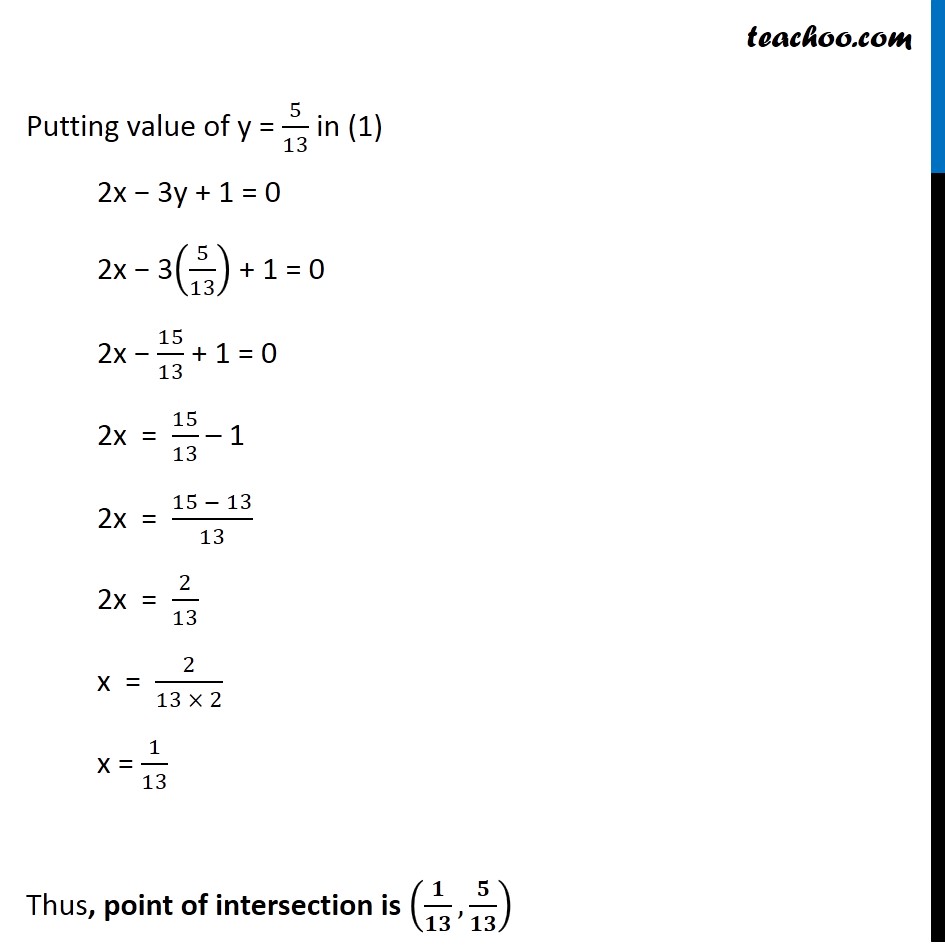
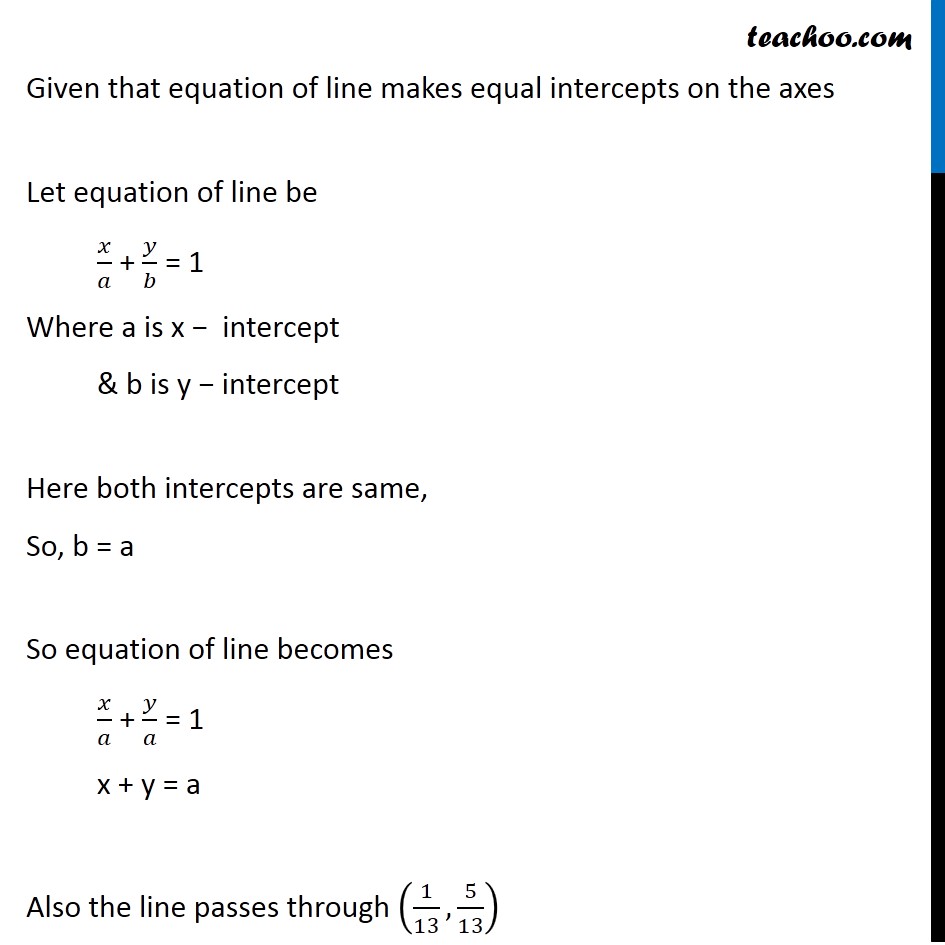
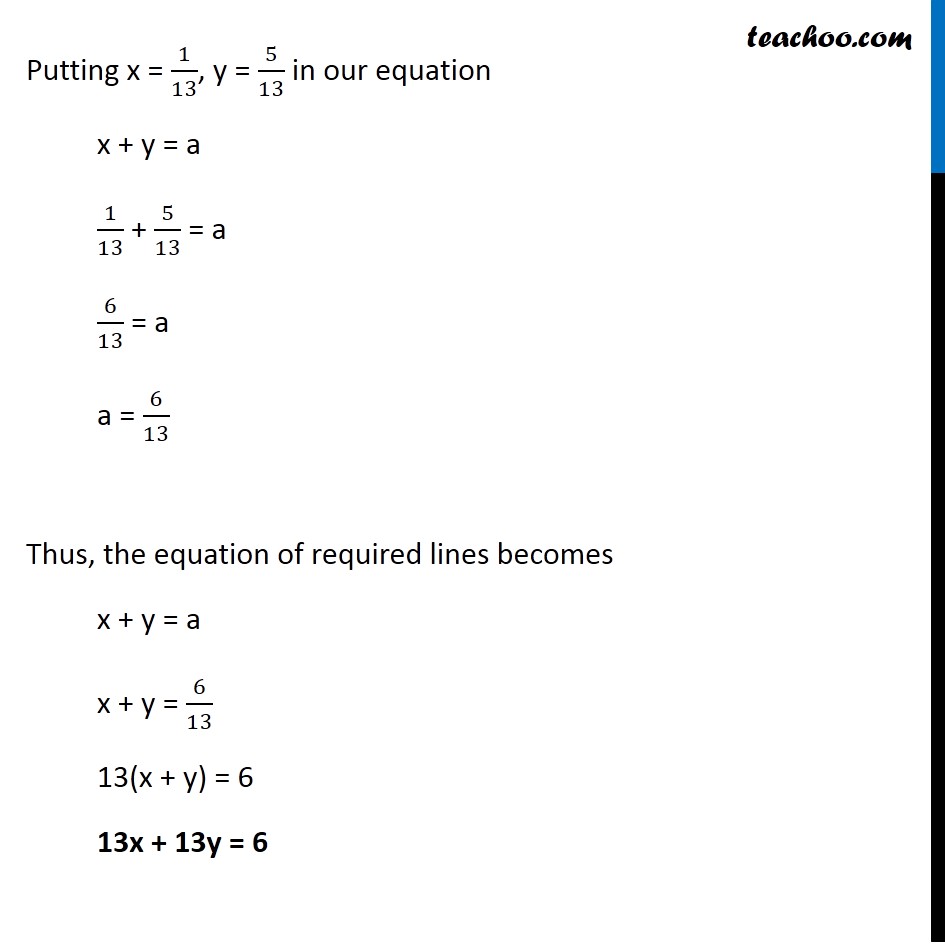
Misc 11 Find the equation of the line passing through the point of intersection of the lines 4x + 7y – 3 = 0 and 2x – 3y + 1 = 0 that has equal intercepts on the axes. Given lines are 4x + 7y − 3 = 0 2x – 3y + 1 = 0 We need to calculate Equation of line that passes through point of intersection of lines (1) & (2) & make equal intercepts on the axes Calculating point of intersection of lines (1) & (2) Misc 11 Find the equation of the line passing through the point of intersection of the lines 4x + 7y – 3 = 0 and 2x – 3y + 1 = 0 that has equal intercepts on the axes. Given lines are 4x + 7y − 3 = 0 2x – 3y + 1 = 0 We need to calculate Equation of line that passes through point of intersection of lines (1) & (2) & make equal intercepts on the axes Calculating point of intersection of lines (1) & (2) Misc 11 Find the equation of the line passing through the point of intersection of the lines 4x + 7y – 3 = 0 and 2x – 3y + 1 = 0 that has equal intercepts on the axes. Given lines are 4x + 7y − 3 = 0 2x – 3y + 1 = 0 We need to calculate Equation of line that passes through point of intersection of lines (1) & (2) & make equal intercepts on the axes Calculating point of intersection of lines (1) & (2) Misc 11 Find the equation of the line passing through the point of intersection of the lines 4x + 7y – 3 = 0 and 2x – 3y + 1 = 0 that has equal intercepts on the axes. Given lines are 4x + 7y − 3 = 0 2x – 3y + 1 = 0 We need to calculate Equation of line that passes through point of intersection of lines (1) & (2) & make equal intercepts on the axes Calculating point of intersection of lines (1) & (2) Misc 11 Find the equation of the line passing through the point of intersection of the lines 4x + 7y – 3 = 0 and 2x – 3y + 1 = 0 that has equal intercepts on the axes. Given lines are 4x + 7y − 3 = 0 2x – 3y + 1 = 0 We need to calculate Equation of line that passes through point of intersection of lines (1) & (2) & make equal intercepts on the axes Calculating point of intersection of lines (1) & (2) From (2) 2x − 3y + 1 = 0 2x = 3y − 1 x = (3𝑦 − 1)/2 Putting value of x in (1) 4x + 7y − 3 = 0 4((3𝑦 − 1)/2) + 7y − 3 = 0 2(3y − 1) + 7y − 3 = 0 6y − 2 + 7y − 3 = 0 13y − 5 = 0 13y = 5 y = 5/13
