You are here
Misc 2 - Chapter 9 Class 11 Straight Lines
Last updated at Dec. 16, 2024 by Teachoo
Chapter 9 Class 11 Straight Lines
Concept wise
- Cordinate geometry questions
- Slope - Finding slope
- Slope - Prependicularity
- Angle between two lines by Slope
- Collinearity of 3 points by sliope
- Point Slope form
- Slope-Intercept form
-
Intercept form
- Normal form
- Given Statement
- Two lines // or/and prependicular
- Angle between two lines
- Distance - Between two parallel lines
- Distance of a point from a line
- Distance of a point from a line along a line
- Other Type of questions - Area of Triangle formed
- Other Type of questions - 3 lines Concurrent
- Other Type of questions - Image
- Other Type of questions - Mix
Transcript
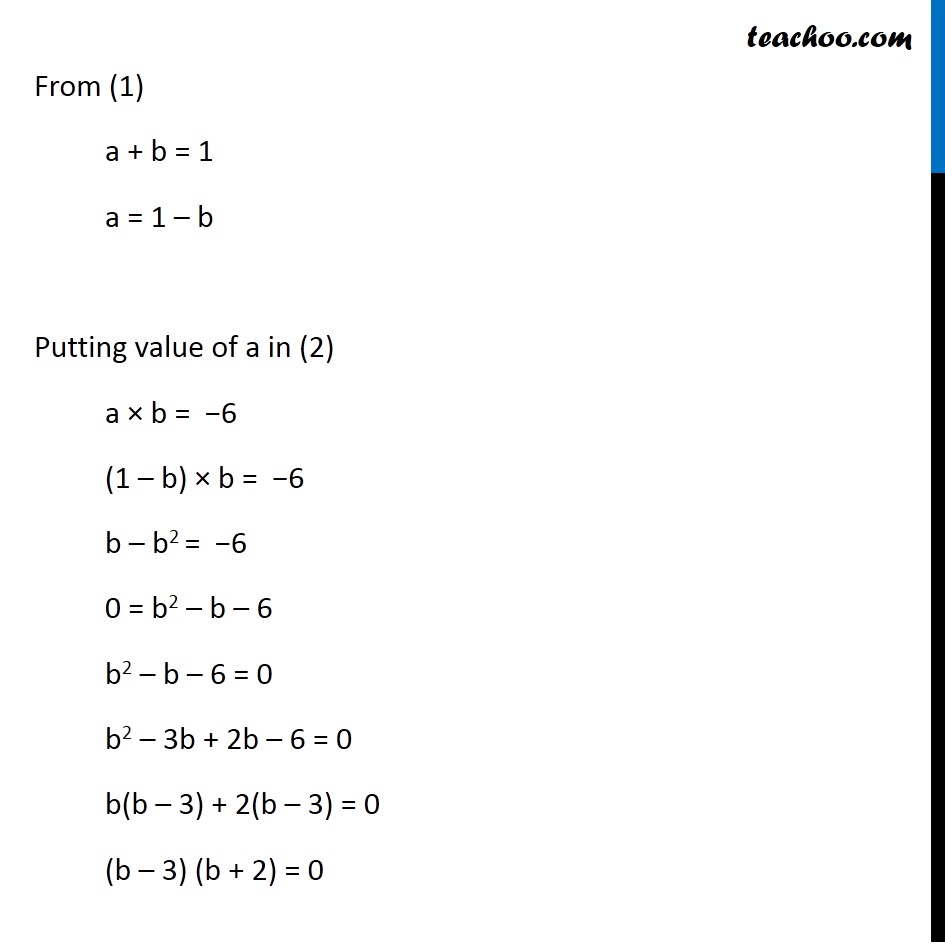
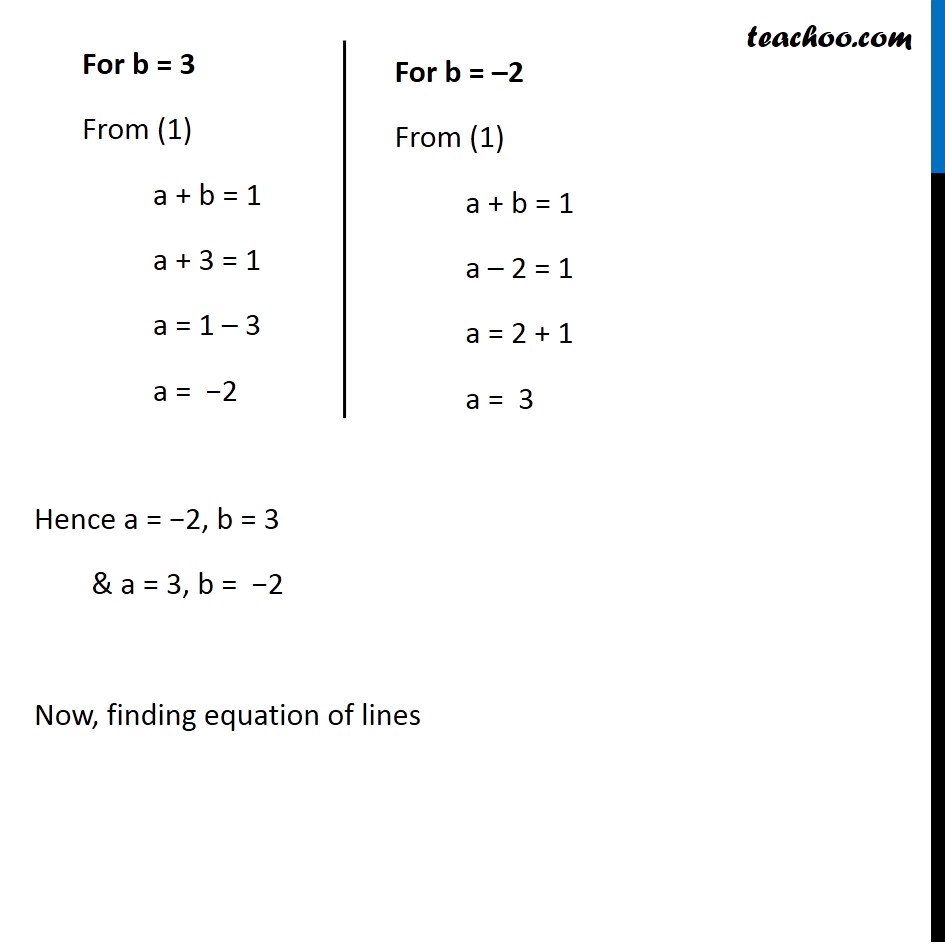
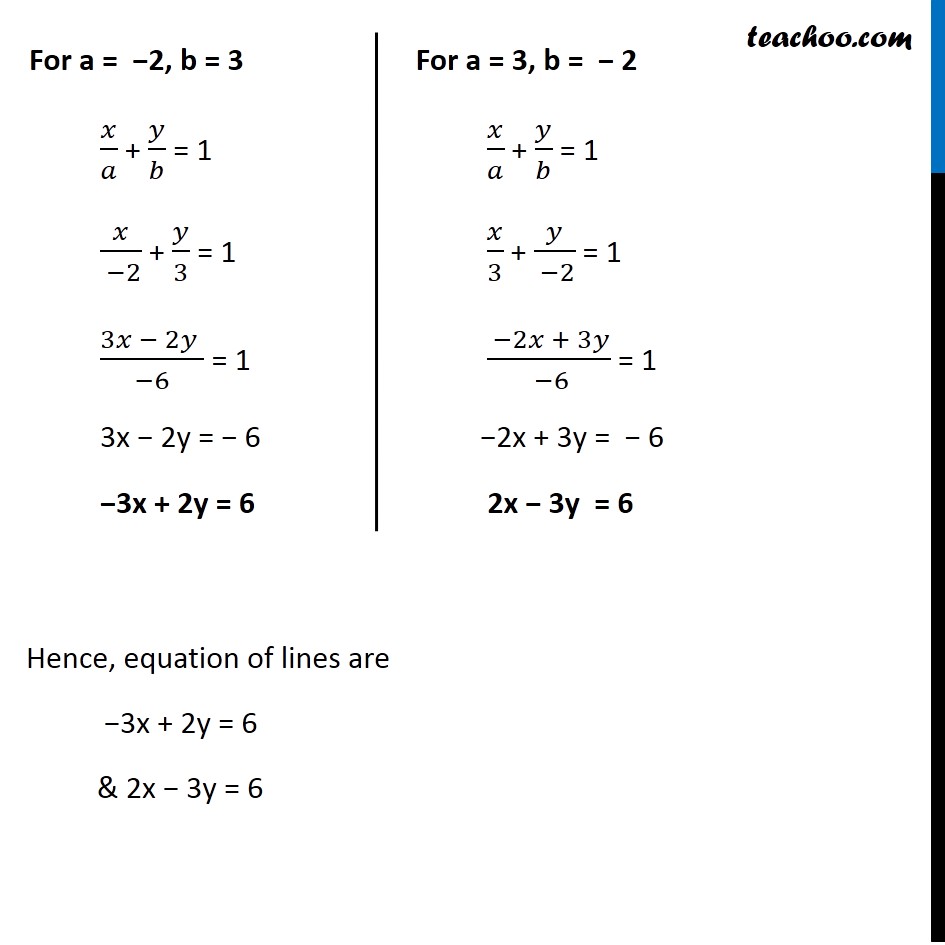
Misc 2 Find the equations of the lines, which cut-off intercepts on the axes whose sum and product are 1 and –6, respectively. Equation of a line by intercept form is 𝑥/𝑎 + 𝑦/𝑏 = 1 where a is x – intercept & b is y – intercept Given that sum of intercept is 1 i.e. a + b = 1 Product of intercept is − 6 i.e. a × b = −6 From (1) a + b = 1 a = 1 – b Putting value of a in (2) a × b = −6 (1 – b) × b = −6 b – b2 = −6 0 = b2 – b – 6 b2 – b – 6 = 0 b2 – 3b + 2b – 6 = 0 b(b – 3) + 2(b – 3) = 0 (b – 3) (b + 2) = 0 So, b = 3, & b = – 2 For b = 3 From (1) a + b = 1 a + 3 = 1 a = 1 – 3 a = −2 For b = –2 From (1) a + b = 1 a – 2 = 1 a = 2 + 1 a = 3 Hence a = −2, b = 3 & a = 3, b = −2 Now, finding equation of lines For a = −2, b = 3 𝑥/𝑎 + 𝑦/𝑏 = 1 𝑥/( −2) + 𝑦/3 = 1 (3𝑥 − 2𝑦 )/( −6 ) = 1 3x − 2y = − 6 −3x + 2y = 6 For a = −2, b = 3 𝑥/𝑎 + 𝑦/𝑏 = 1 𝑥/( −2) + 𝑦/3 = 1 (3𝑥 − 2𝑦 )/( −6 ) = 1 3x − 2y = − 6 −3x + 2y = 6 For a = −2, b = 3 𝑥/𝑎 + 𝑦/𝑏 = 1 𝑥/( −2) + 𝑦/3 = 1 (3𝑥 − 2𝑦 )/( −6 ) = 1 3x − 2y = − 6 −3x + 2y = 6 Hence, equation of lines are −3x + 2y = 6 & 2x − 3y = 6
