Question 8 - Two lines // or/and prependicular - Chapter 9 Class 11 Straight Lines

Transcript

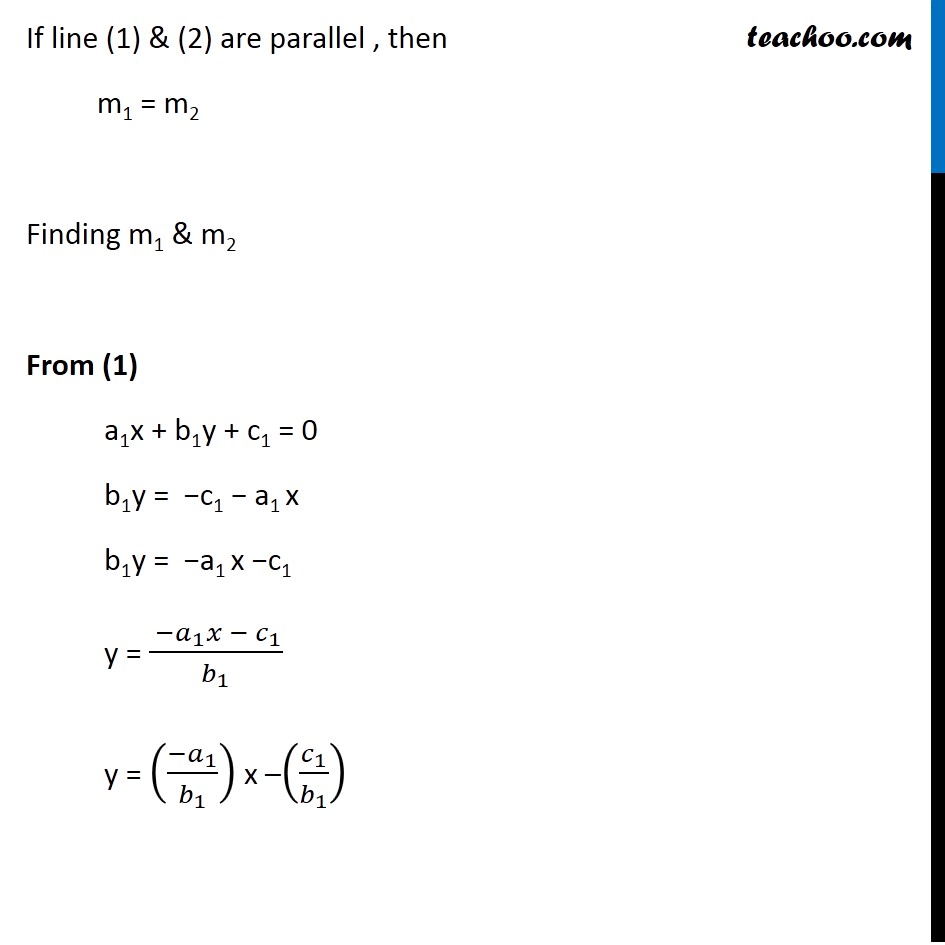
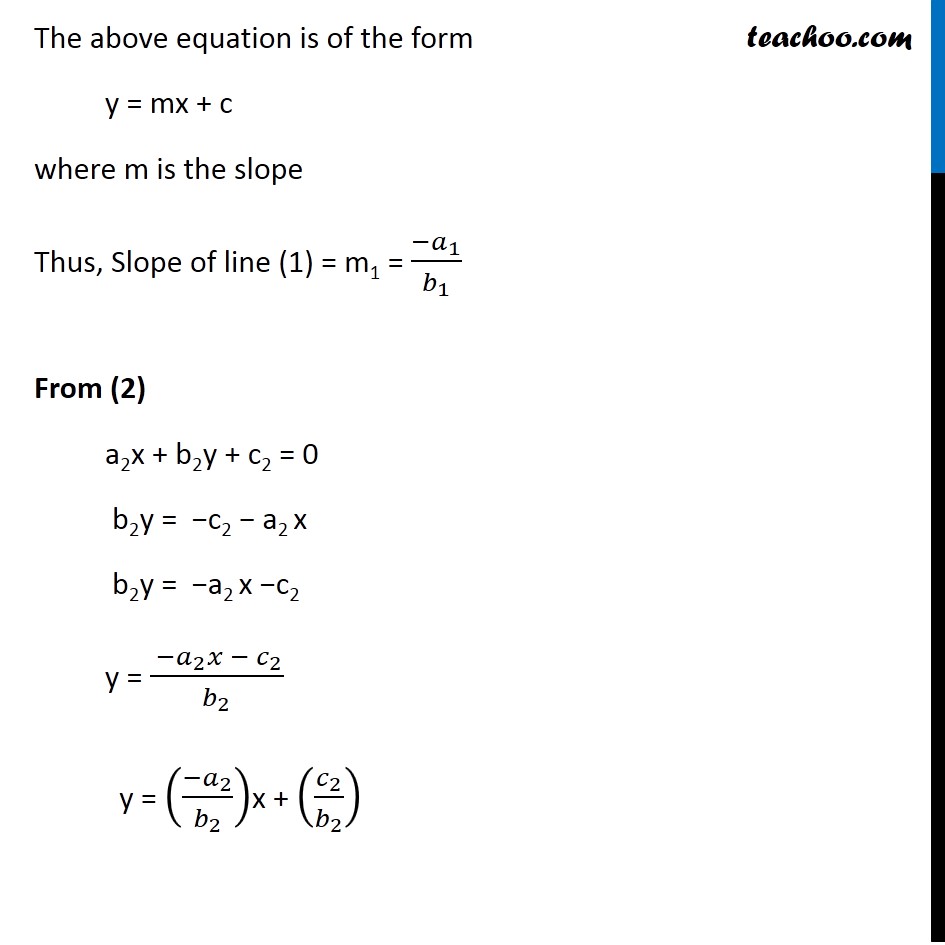
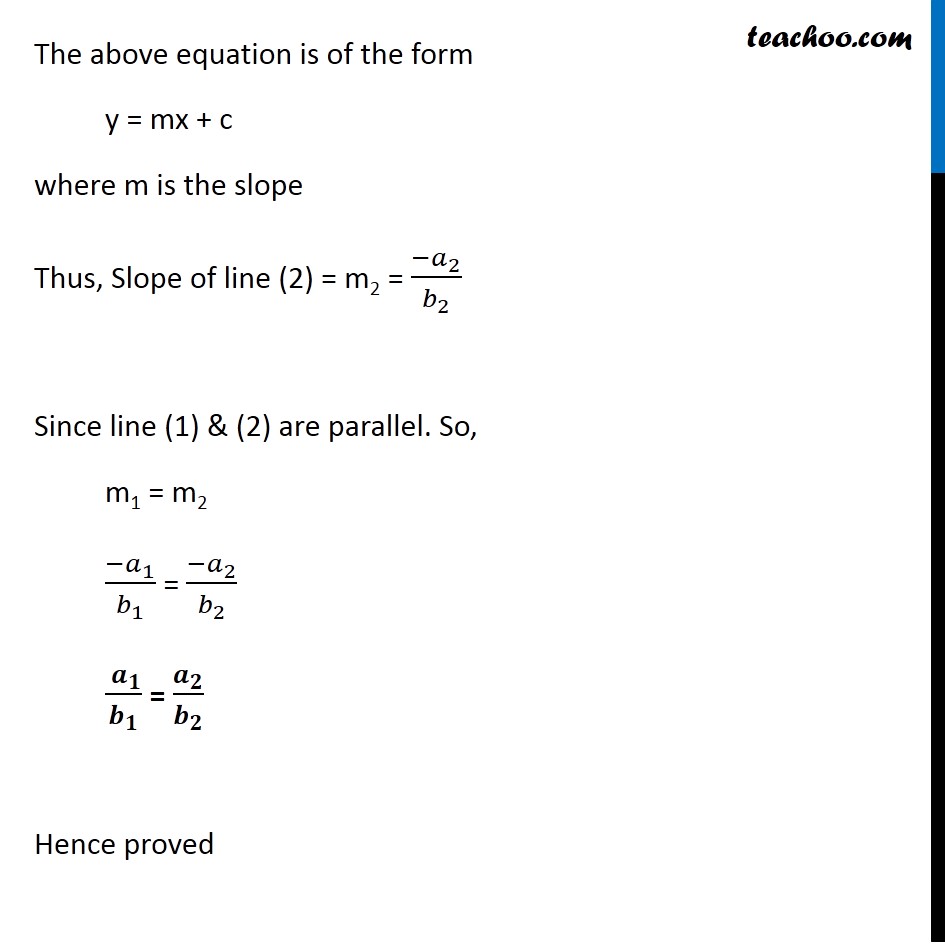
Question 8 Show that two lines a1x + b1y + c1 = 0 and a2 x + b2 y + c2 = 0 , where b1, b2 ≠ 0 are: (i) Parallel if 𝑎_1/𝑏_1 = 𝑎2/𝑏2 The given lines are a1x + b1y + c1 = 0 & a2 x + b2 y + c2 = 0 Let slope of line (1) be m1 & slope of line (2) be m2 If two lines are parallel, then their slopes are equal If line (1) & (2) are parallel , then m1 = m2 Finding m1 & m2 From (1) a1x + b1y + c1 = 0 b1y = −c1 − a1 x b1y = −a1 x −c1 y = ( −𝑎_1 𝑥 − 𝑐_1)/𝑏_1 y = ((−𝑎_1)/𝑏_1 ) x –(𝑐_1/𝑏_1 ) The above equation is of the form y = mx + c where m is the slope Thus, Slope of line (1) = m1 = (−𝑎_1)/𝑏_1 From (2) a2x + b2y + c2 = 0 b2y = −c2 − a2 x b2y = −a2 x −c2 y = ( −𝑎_2 𝑥 − 𝑐_2)/𝑏_2 y = ((−𝑎_2)/𝑏_2 )x + (𝑐_2/𝑏_2 ) The above equation is of the form y = mx + c where m is the slope Thus, Slope of line (2) = m2 = (−𝑎_2)/𝑏_2 Since line (1) & (2) are parallel. So, m1 = m2 (−𝑎_1)/𝑏_1 = (−𝑎_2)/𝑏_2 ( 𝒂_𝟏)/𝒃_𝟏 = 𝒂_𝟐/𝒃_𝟐 Hence proved Question 8 Show that two lines a1x + b1y + c1 = 0 and a2 x + b2 y + c2 = 0 , where b1, b2 ≠ 0 are: (ii) Perpendicular if a1a2 + b1b2 = 0 . If two lines are perpendicular, then product of their slope is equal to −1 Since line (1) & (2) are perpendicular ⇒ (Slope of line 1) × (Slope of line 2) = −1 m1 × m2 = − 1 ( −𝑎_1)/𝑏_1 × ( −𝑎_2)/𝑏_2 = −1 ( 𝑎_1)/𝑏_1 × 𝑎_2/𝑏_2 = −1 a1a2 = −b1b2 a1a2 + b1b2 = 0 Hence proved