Example 3 - Chapter 10 Class 11 Straight Lines (Term 1)
Last updated at Feb. 3, 2020 by Teachoo
Examples
Example 1 (a)
Example 1 (b)
Example 1 (c) Important
Example 1 (d)
Example 2 Important
Example 3 Important You are here
Example 4
Example 5
Example 6 Important
Example 7
Example 8
Example 9 (i)
Example 9 (ii) Important
Example 10
Example 11
Example 12
Example 13 Important
Example 14 Important
Example 15 Important
Example 16
Example 17
Example 18 Important
Example 19
Example 20
Example 21 Important
Example 22 Important
Example 23
Example 24 Important
Example 25 Important
Chapter 10 Class 11 Straight Lines (Term 1)
Serial order wise
- Ex 10.1
- Ex 10.2
- Ex 10.3
-
Examples
- Miscellaneous
Transcript
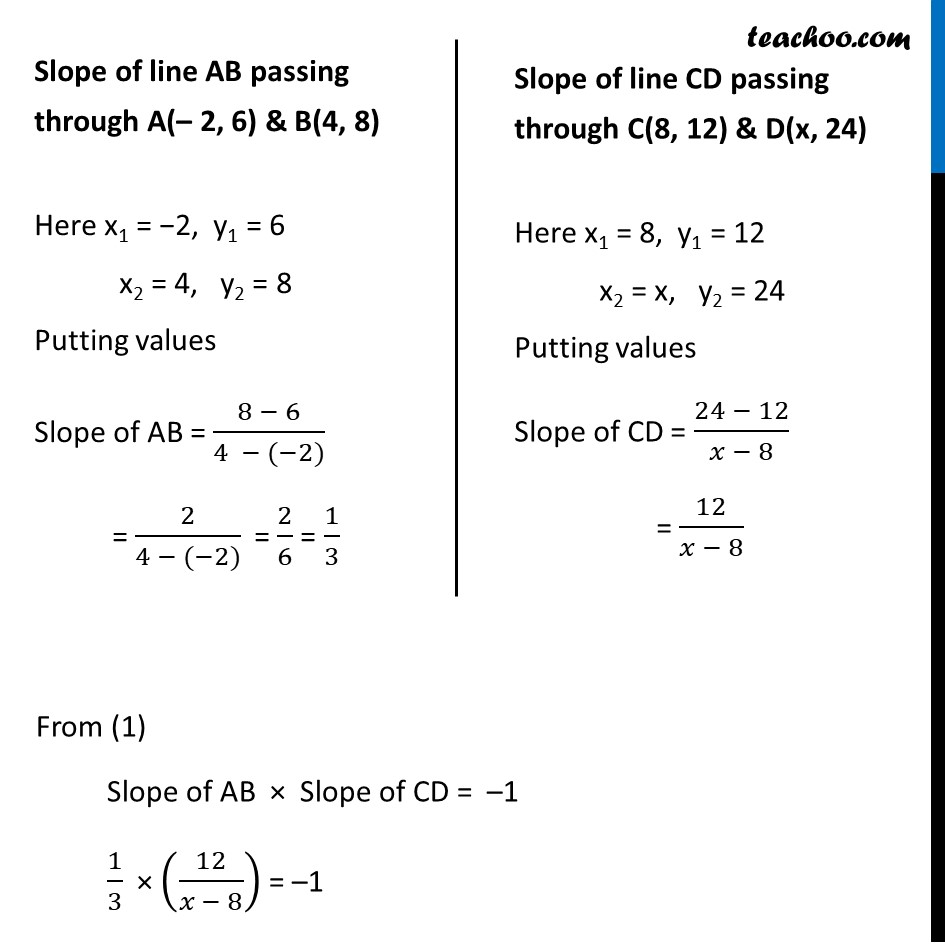
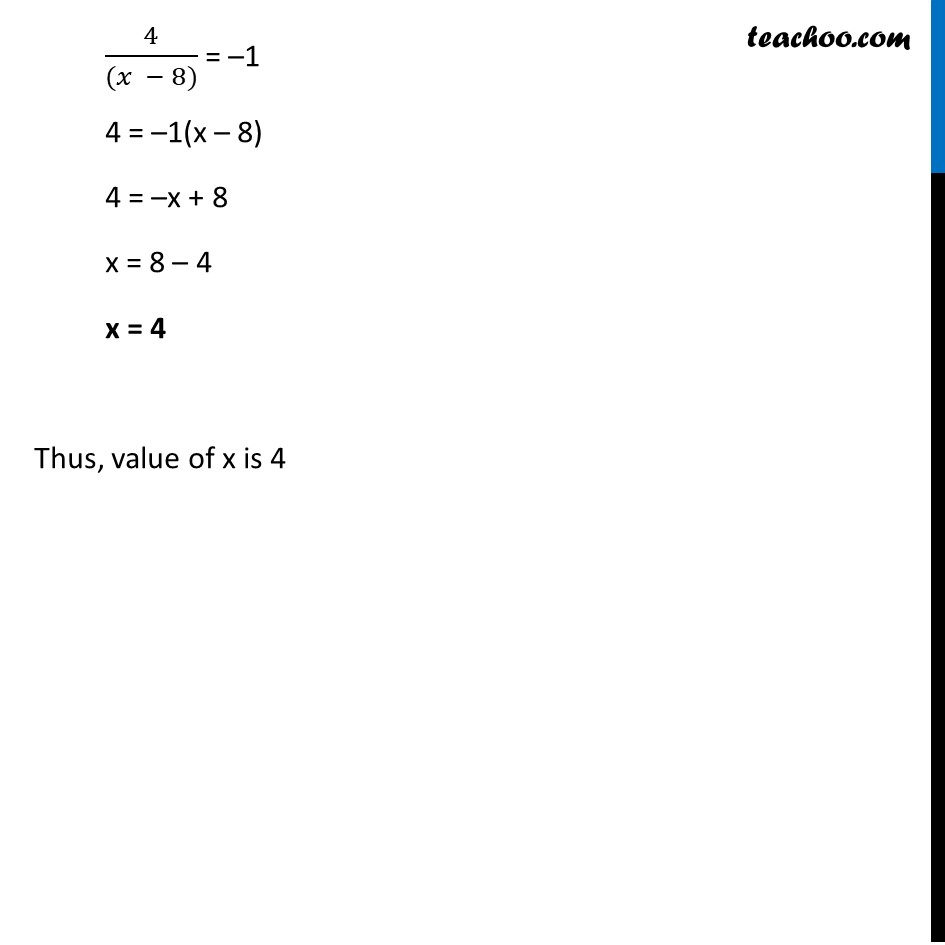
Example 3 Line through the points (–2, 6) and (4, 8) is perpendicular to the line through the points (8, 12) and (x, 24). Find the value of x. Let points be A(–2, 6), B(4, 8) , C(8, 12) and D(x, 24) If two lines are perpendicular , then product of their slope is –1 So, Slope of AB × Slope of CD = –1 We know that slope of a line through the points (x1, y1) , (x2, y2)is m = (𝑦_2 − 𝑦_1)/(𝑥_2 − 𝑥_1 ) Slope of line AB passing through A(– 2, 6) & B(4, 8) Here x1 = −2, y1 = 6 x2 = 4, y2 = 8 Putting avalues Slope of AB = (8 − 6)/(4 − (−2)) = 2/(4 − (−2)) = 2/6 = 1/3 Slope of line CD passing through C(8, 12) & D(x, 24) Here x1 = 8, y1 = 12 x2 = x, y2 = 24 Putting values Slope of CD = (24 − 12)/(𝑥 − 8) = 12/(𝑥 − 8) From (1) Slope of AB × Slope of CD = –1 1/3 × (12/(𝑥 − 8)) = –1 4/((𝑥 − 8)) = –1 4 = –1(x – 8) 4 = –x + 8 x = 8 – 4 x = 4 Thus, value of x is 4
