Ex 9.3, 11 - Chapter 9 Class 11 Straight Lines

Transcript

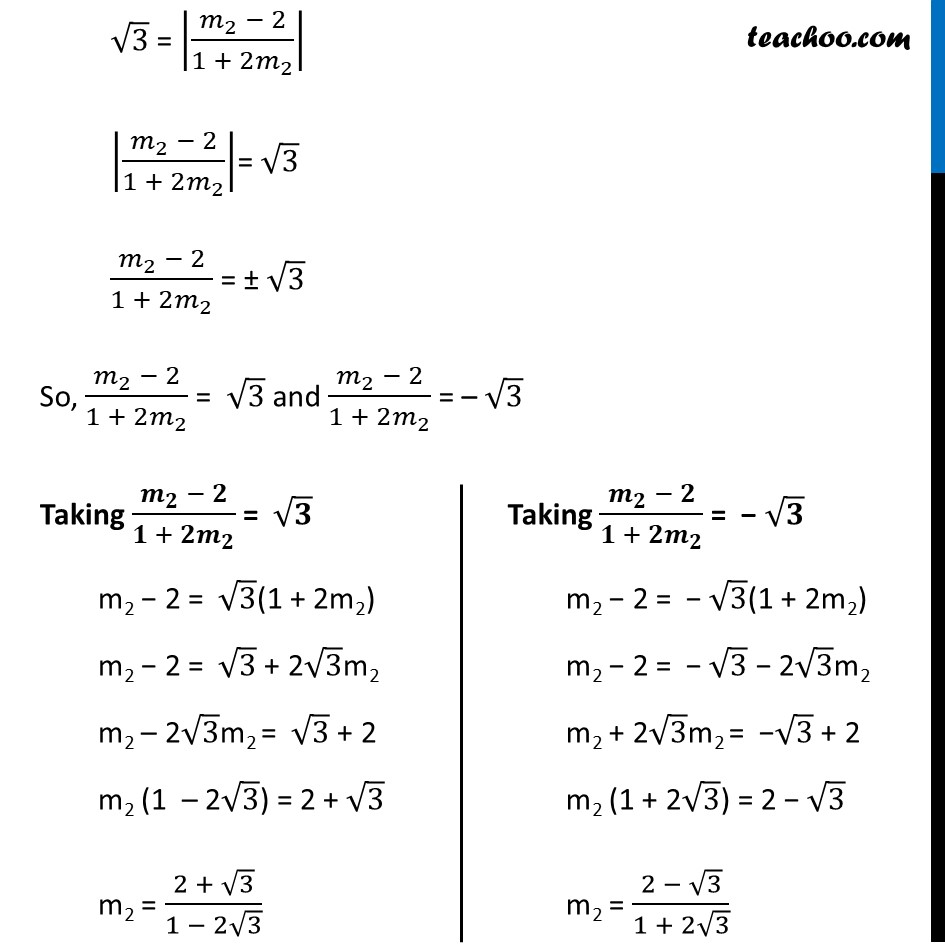
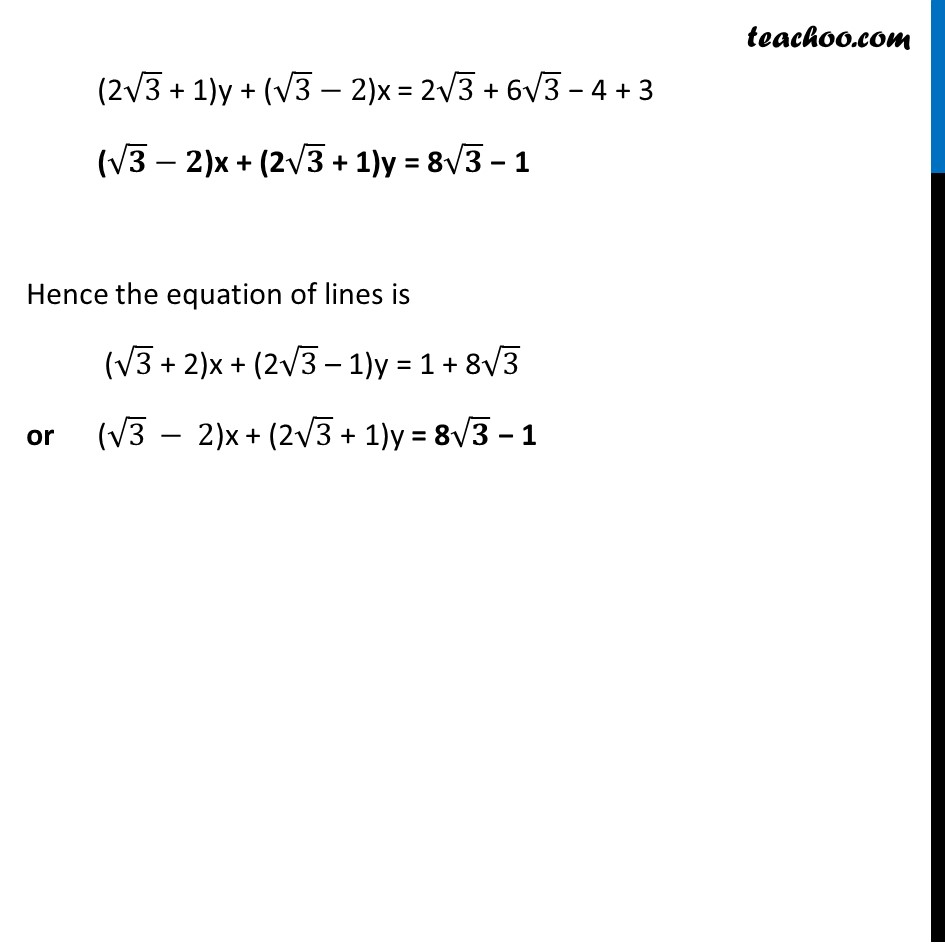
Ex 9.3, 11 Two lines passing through the point (2, 3) intersects each other at an angle of 60°. If slope of one line is 2, find equation of the other line. We know that Angle between 2 lines be tan θ =|(𝑚_2 − 𝑚_1)/(1 + 𝑚_2 𝑚_1 )| Here m1 = Slope of one line = 2 θ = 60° (given) We need to find m2 Putting the values tan 60° = |(𝑚_2 − 2)/(1 + 2 × 𝑚_2 )| √3 = |(𝑚_2 − 2)/(1 + 2𝑚_2 )| |(𝑚_2 − 2)/(1 + 2𝑚_2 )|= √3 (𝑚_2 − 2)/(1 + 2𝑚_2 ) = ± √3 So, (𝑚_2 − 2)/(1 + 2𝑚_2 ) = √3 and (𝑚_2 − 2)/(1 + 2𝑚_2 ) = – √3 Taking (𝒎_𝟐 − 𝟐)/(𝟏 + 𝟐𝒎_𝟐 ) = √𝟑 m2 − 2 = √3(1 + 2m2) m2 − 2 = √3 + 2√3m2 m2 – 2√3m2 = √3 + 2 m2 (1 – 2√3) = 2 + √3 m2 = (2 + √3)/(1 − 2√3) Taking (𝒎_𝟐 − 𝟐)/(𝟏 + 𝟐𝒎_𝟐 ) = − √𝟑 m2 − 2 = − √3(1 + 2m2) m2 − 2 = − √3 − 2√3m2 m2 + 2√3m2 = −√3 + 2 m2 (1 + 2√3) = 2 − √3 m2 = (2 − √3)/(1 + 2√3) We know that equation of a line passing through (x1, y1) & having slope m is (y − y1) = m(x − x1) Equation of a line passing through (2, 3) & having slope (𝟐 + √𝟑)/(𝟏 − 𝟐√𝟑) is (y − 3) = ( (2 + √3))/(1 − 2√3) (x − 2) (1 − 2√3)(y − 3) = (2 + √3)(x − 2) 1(y − 3) − 2√3(y − 3) = 2(x – 2) + √3(x − 2) y – 3 − 2√3y + 6√3 = 2x – 4 + √3x − 2√3 y − 2√3y − 4x − √3x = – 6√3 – 2√3 – 4 + 3 y (1 − 2√3) − x(√3 + 2) = – 1 – 8√3 1 + 8√3 = x(√3 + 2) + y (2√3 – 1) (√𝟑 + 2)x + (2√𝟑 – 1)y = 1 + 8√𝟑 Equation of a line passing through (2, 3) & having slope (𝟐 − √𝟑)/(𝟏 + 𝟐√𝟑) is (y − 3) = (2 − √3)/(2√3 + 1)(x − 2) (2√3 + 1) (y − 3) = (2 − √3) (x − 2) 2√3 (y − 3) + 1 (y − 3) = 2(x − 2) − √3(x − 2) 2√3 (y − 3) + 1 (y − 3) = 2(x − 2) − √3(x − 2) 2√3y − 6√3 + y − 3 = 2x − 4 − √3x + 2√3 2√3y + y − 6√3 − 3 = 2x − √3x − 4 + 2√3 (2√3 + 1)y − 6√3 − 3 = (2 − √3)x − 4 + 2√3 (2√3 + 1)y = − (√3 − 2)x − 4 + 2√3 + 6√3 + 3 (2√3 + 1)y + (√3−2)x = 2√3 + 6√3 − 4 + 3 (√𝟑−𝟐)x + (2√𝟑 + 1)y = 8√𝟑 − 1 Hence the equation of lines is (√3 + 2)x + (2√3 – 1)y = 1 + 8√3 or (√3 − 2)x + (2√3 + 1)y = 8√3 − 1