You are here
Question 1 (i) - Normal form - Chapter 9 Class 11 Straight Lines
Last updated at Dec. 16, 2024 by Teachoo
Chapter 9 Class 11 Straight Lines
Concept wise
- Cordinate geometry questions
- Slope - Finding slope
- Slope - Prependicularity
- Angle between two lines by Slope
- Collinearity of 3 points by sliope
- Point Slope form
- Slope-Intercept form
- Intercept form
-
Normal form
- Given Statement
- Two lines // or/and prependicular
- Angle between two lines
- Distance - Between two parallel lines
- Distance of a point from a line
- Distance of a point from a line along a line
- Other Type of questions - Area of Triangle formed
- Other Type of questions - 3 lines Concurrent
- Other Type of questions - Image
- Other Type of questions - Mix
Transcript
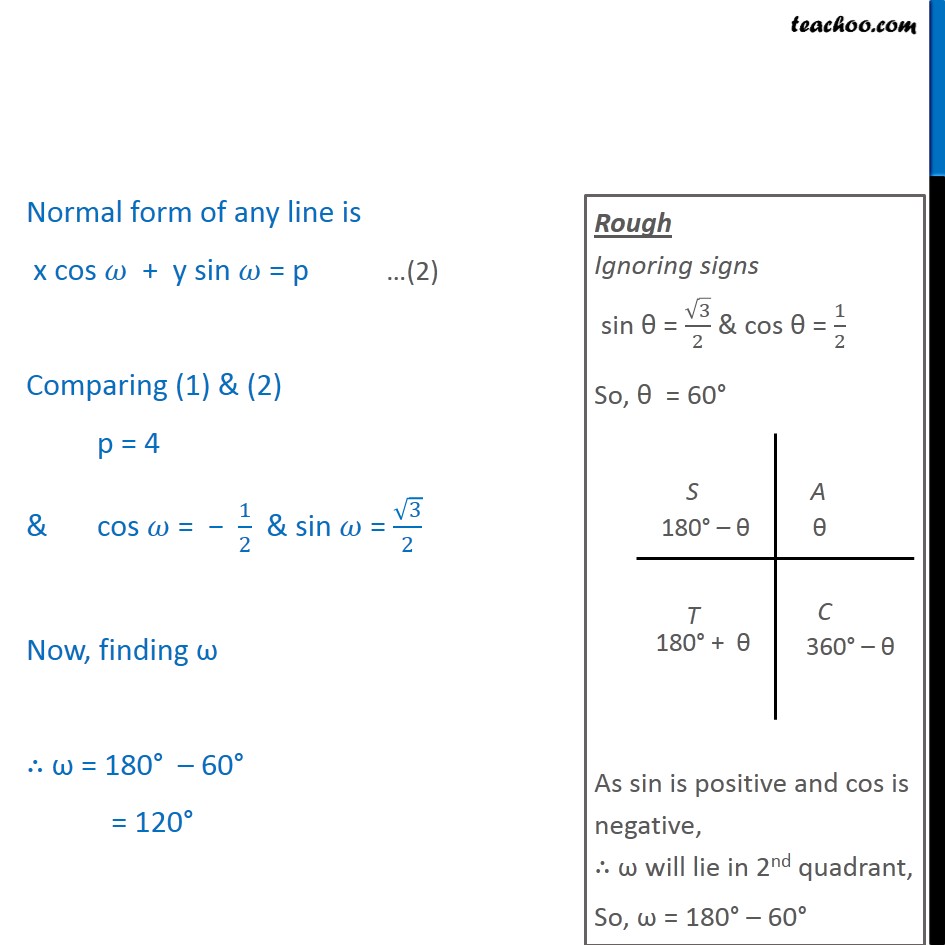
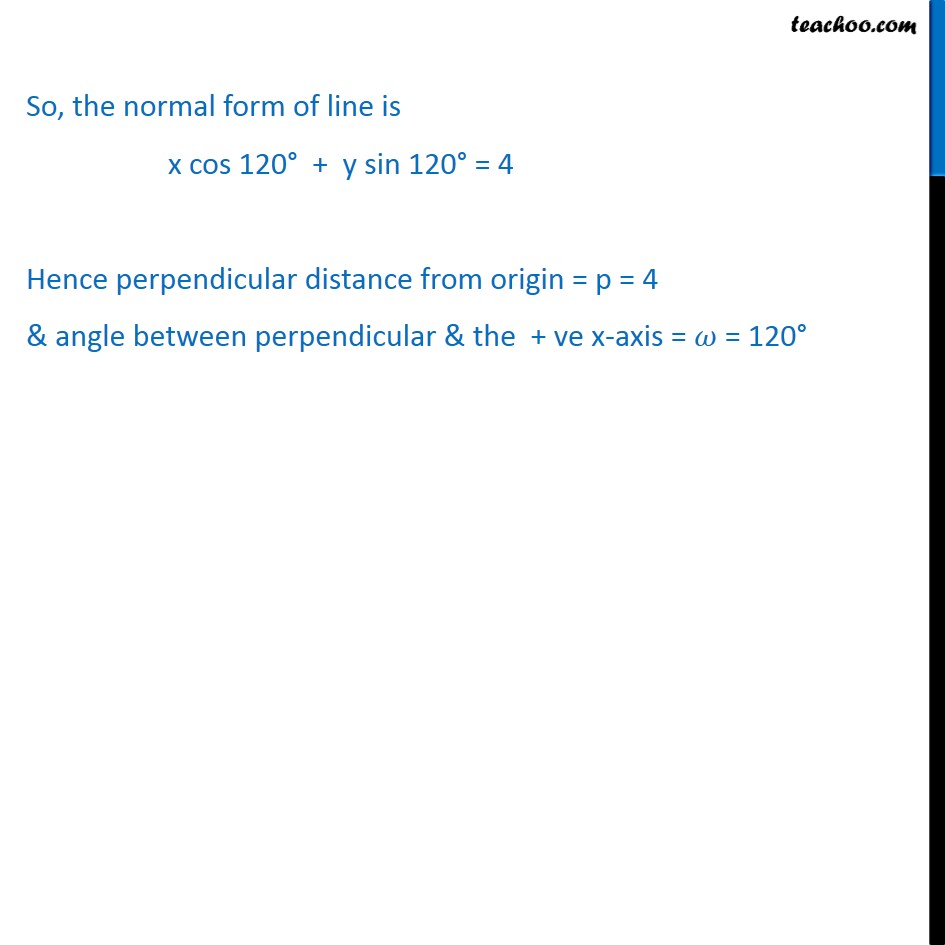
Ex10.3, 3 Reduce the following equations into normal form. Find their perpendicular distances from the origin and angle between perpendicular and the positive x-axis. x 3 y + 8 = 0 x 3 y + 8 = 0 8 = x + 3 x + 3 = 8 Divide equation by (( 1)2 + ( 3)^2 ) = (1 + 3)= 4 = 2 ( + 3 )/2 = 8/2 ( )/2 + 3/2y = 4 x(( 1)/2) + y ( 3/2) = 4 Normal form of any line is x cos + y sin = p Comparing (1) & (2) p = 4 & cos = 1/2 & sin = 3/2 Now, finding = 180 60 = 120 So, the normal form of line is x cos 120 + y sin 120 = 4 Hence perpendicular distance from origin = p = 4 & angle between perpendicular & the + ve x-axis = = 120
