You are here
Ex 9.1, 9 - Chapter 9 Class 11 Straight Lines
Last updated at Dec. 16, 2024 by Teachoo
Chapter 9 Class 11 Straight Lines
Concept wise
- Cordinate geometry questions
-
Slope - Finding slope
- Slope - Prependicularity
- Angle between two lines by Slope
- Collinearity of 3 points by sliope
- Point Slope form
- Slope-Intercept form
- Intercept form
- Normal form
- Given Statement
- Two lines // or/and prependicular
- Angle between two lines
- Distance - Between two parallel lines
- Distance of a point from a line
- Distance of a point from a line along a line
- Other Type of questions - Area of Triangle formed
- Other Type of questions - 3 lines Concurrent
- Other Type of questions - Image
- Other Type of questions - Mix
Transcript
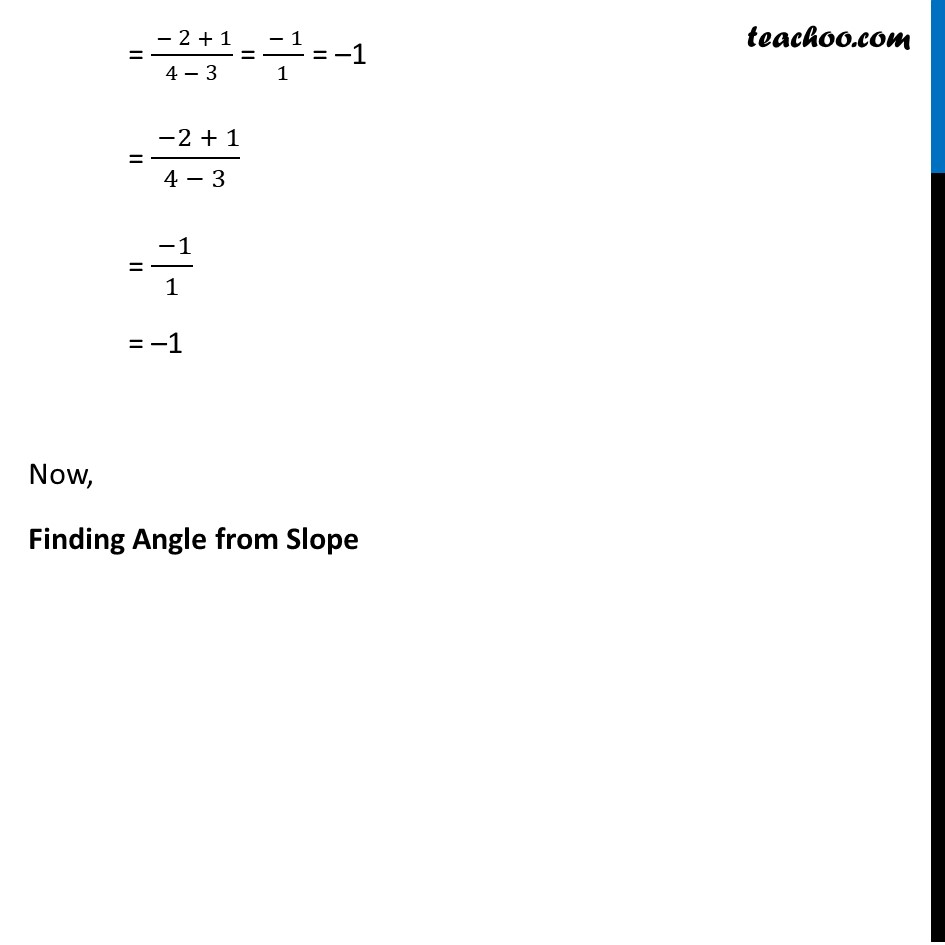
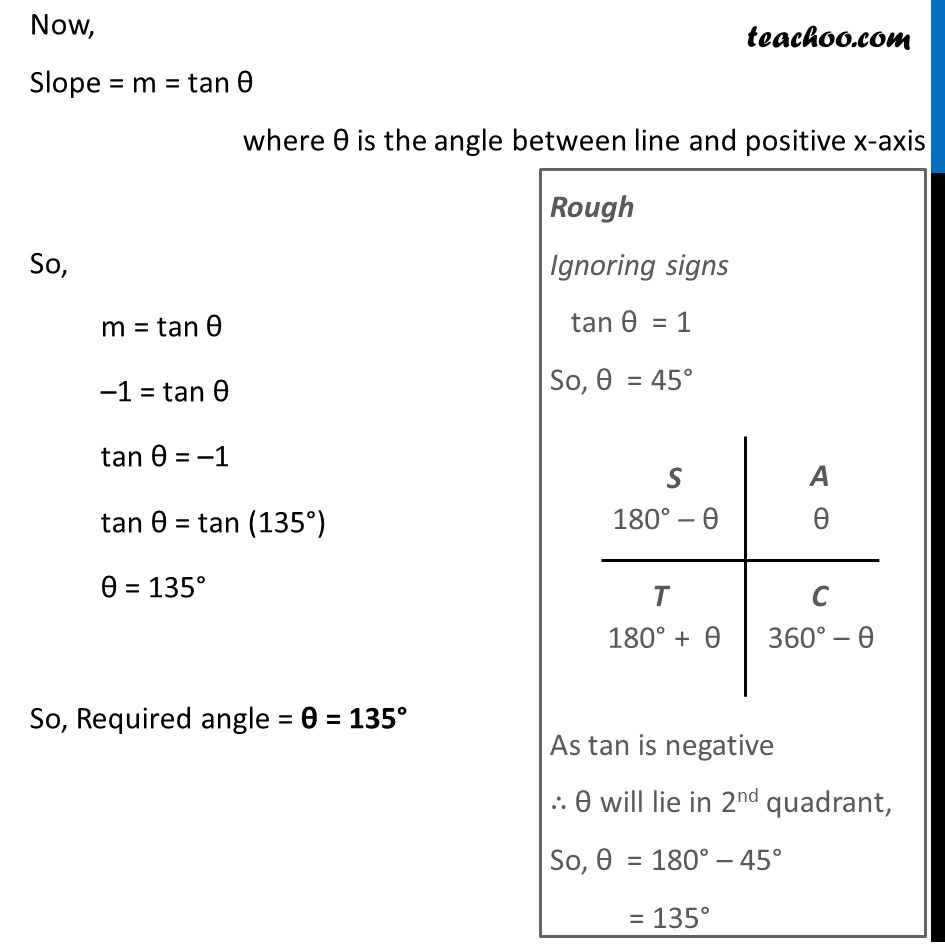
Ex 9.1, 9 Find the angle between the x-axis and the line joining the points (3, –1) and (4, –2). First we find slope of line joining the points (3, –1) and (4, –2). We know that slope of line passing through (x1, y1) and (x2, y2) is m = (𝑦_2 − 𝑦_1)/(𝑥_2 − 𝑥_1 ) Here x1 = 3, y1 = –1 & x2 = 4, y2 = –2 Slope of line joining (3, –1) and (4, –2) is m = ( −2 − (−1))/(4 − 3) = ( − 2 + 1)/(4 − 3) = ( − 1)/1 = –1 Ex 9.1, 9 Find the angle between the x-axis and the line joining the points (3, –1) and (4, –2). First we find slope of line joining the points (3, –1) and (4, –2). We know that slope of line passing through (x1, y1) and (x2, y2) is m = (𝑦_2 − 𝑦_1)/(𝑥_2 − 𝑥_1 ) Here x1 = 3, y1 = –1 & x2 = 4, y2 = –2 Slope of line joining (3, –1) and (4, –2) is m = ( −2 − (−1))/(4 − 3) = ( − 2 + 1)/(4 − 3) = ( − 1)/1 = –1 = ( −2 + 1)/(4 − 3) = ( −1)/1 = –1 Now, Finding Angle from Slope Now, Slope = m = tan θ where θ is the angle between line and positive x-axis So, m = tan θ –1 = tan θ tan θ = –1 tan θ = tan (135°) θ = 135° So, Required angle = θ = 135° Rough Ignoring signs tan θ = 1 So, θ = 45° As tan is negative ∴ θ will lie in 2nd quadrant, So, θ = 180° – 45° = 135°
