Example 13 - Chapter 9 Class 11 Sequences and Series
Last updated at Dec. 8, 2016 by Teachoo
Transcript
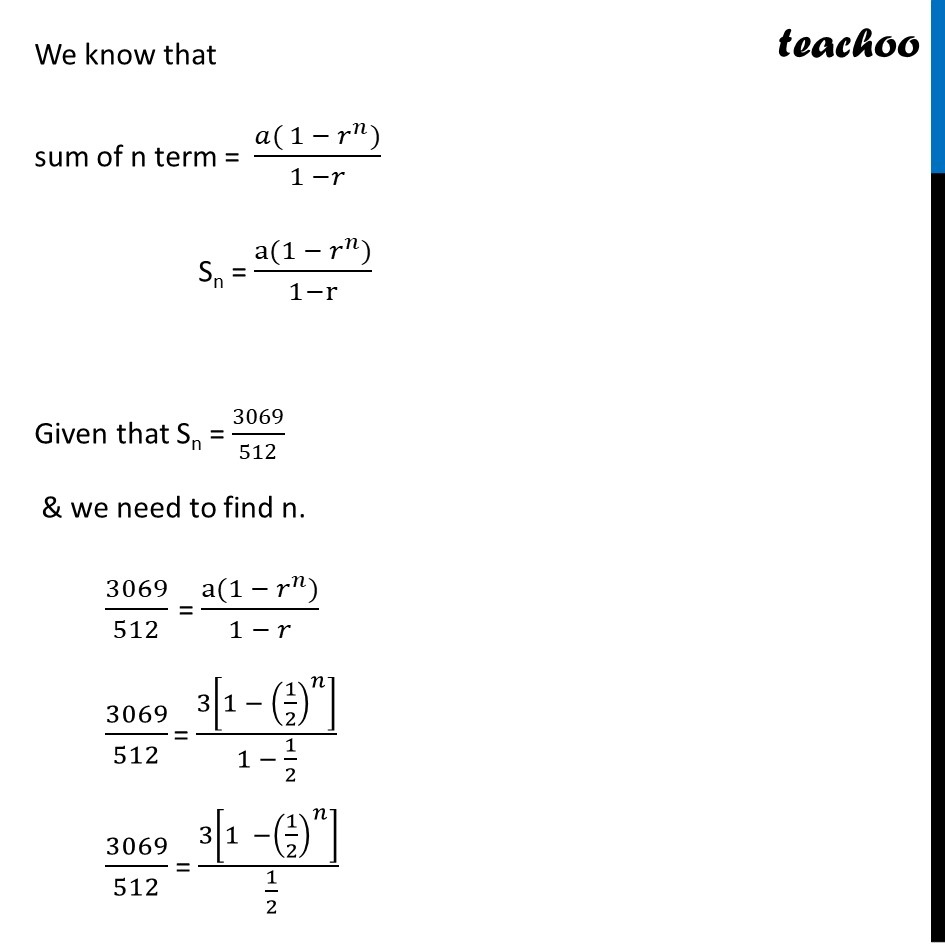
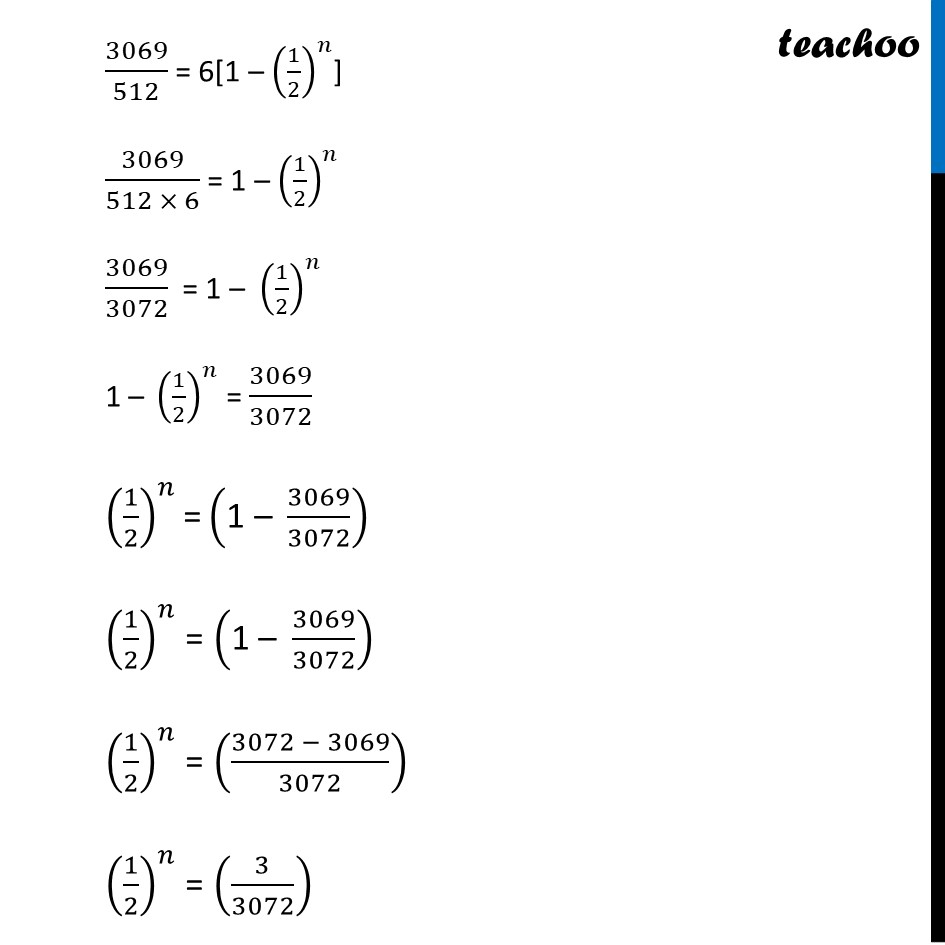
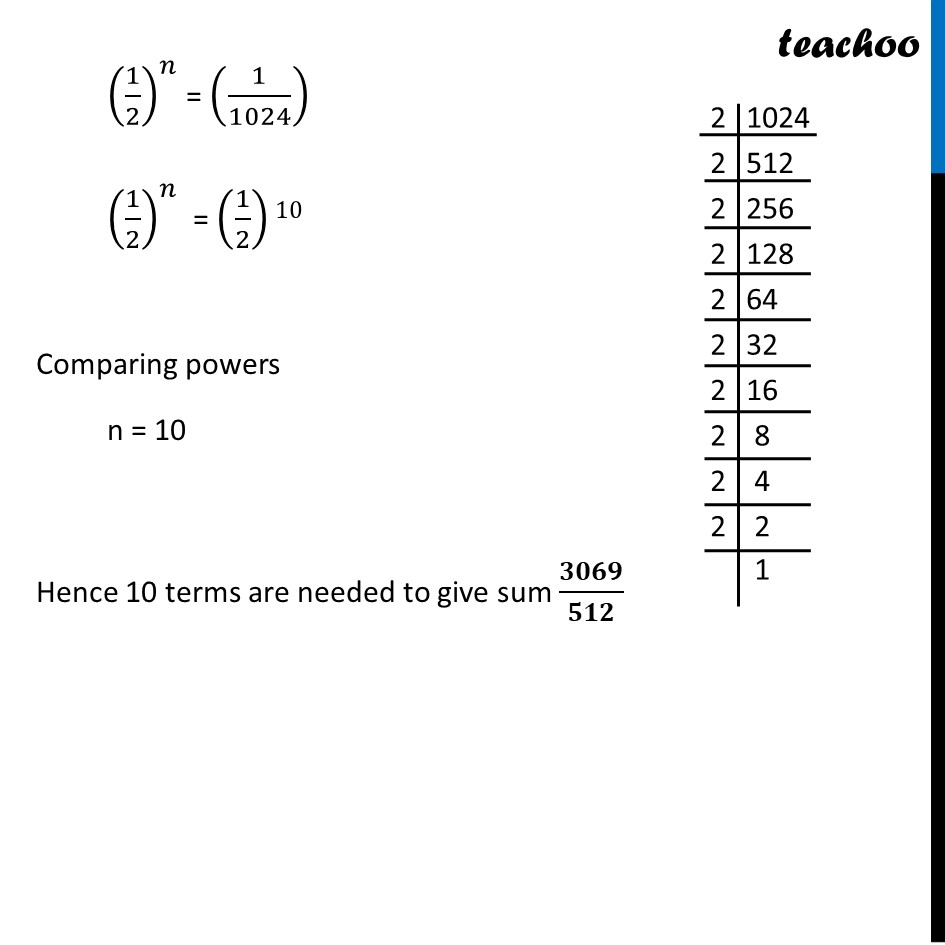
Example 13, How many terms of the G.P. 3, 3/2, 3/4 , , ,... are needed to give the sum 3069/512 ? Here First term = a = 3, Common ratio r = (3/2)/3 = 3/(2 3) = 1/2 We know that sum of n term = ( ( 1 ^ ))/(1 ) Sn = (a(1 ^ ))/(1 r) Given that Sn = 3069/512 & we need to find n. 3069/512 = (a(1 ^ ))/(1 ) 3069/512 = (3[1 (1/2)^ ])/(1 1/2) 3069/512 =(3[1 (1/2)^ ])/( 1/2) 3069/512 = 6[1 (1/2)^ ] 3069/(512 6) = 1 (1/2)^ 3069/3072 = 1 (1/2)^ 1 (1/2)^ = 3069/3072 " " (1/2)^ " = " ("1 " 3069/3072) (1/2)^ = ((3072 3069)/3072) (1/2)^ = (3/3072) (1/2)^ = (1/1024) (1/2)^ = (1/2)10 Comparing powers n = 10 Hence 10 terms are needed to give sum 3069/512
Examples
Example 1
Example 2
Example 3
Example 4
Example 5
Example 6 Important
Example 7
Example 8
Example 9
Example 10
Example 11
Example 12
Example 13 You are here
Example 14 Important
Example 15 Important
Example 16
Example 17 Important
Example 18 Important
Example 19 Important
Example 20
Example 21 Important
Example 22
Example 23 Important
Example 24 Important
Chapter 9 Class 11 Sequences and Series
Serial order wise
- Ex 9.1
- Ex 9.2
- Ex 9.3
- Ex 9.4
-
Examples
- Miscellaneous
About the Author
Davneet Singh
Davneet Singh is a graduate from Indian Institute of Technology, Kanpur. He has been teaching from the past 10 years. He provides courses for Maths and Science at Teachoo.