Example 12 - Chapter 9 Class 11 Sequences and Series
Last updated at May 29, 2018 by Teachoo
Transcript
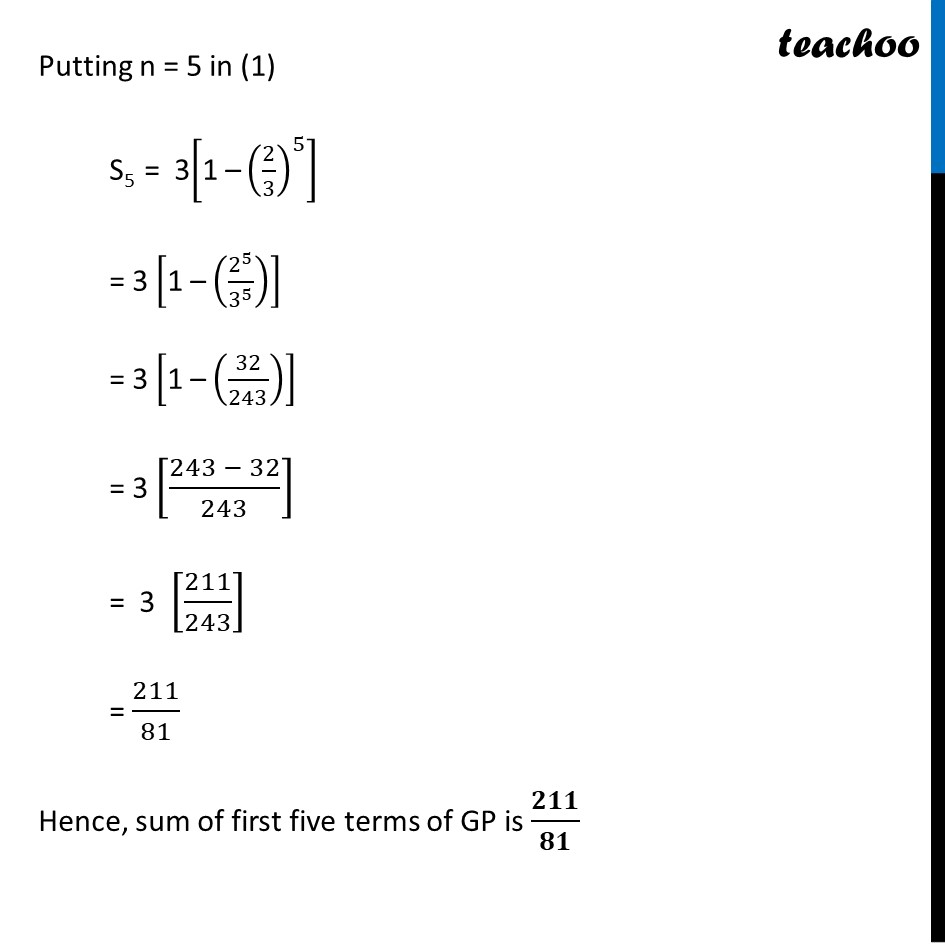
Example 12 Find the sum of first n terms and the sum of first 5 terms of the geometric series 1 + 2/3 "+" 4/9 + ……… 1 + 2/3 "+" 4/9 + ……… We know that Sn = (a(1 − 𝑟^𝑛))/(1 − r) where Sn = sum of n terms of GP n is the number of terms a is the first term r is the common ratio Here, First term = a = 1 and common ratio = r = (2/3)/1 Now, we need to calculate sum of n terms of GP We use the formula, Sn = (a(1 − 𝑟^𝑛))/(1 − r) Putting a = 1 , r = 2/3 = 1(1 − ( 2/3)^𝑛 )/(1 − 2/3) = (1 − ( 2/3)^𝑛)/((3 − 2)/3) = (1 − ( 2/3)^𝑛)/(1/3) = 3/1 ["1 –" (2/3)^𝑛 ] = 3["1 –" (2/3)^𝑛 ] Thus, Sum of n terms = Sn = 3["1 –" (2/3)^𝑛 ] Now, we need to find sum of first 5 terms of GP Putting n = 5 in Sn S5 = 3["1 –" (2/3)^5 ] = 3 ["1 –" (2^5/3^5 )] = 3 ["1 –" (32/243)] = 3 [(243 − 32)/243] = 3 [211/243] = 211/81 Hence, sum of first five terms of GP is 211/81
Examples
Example 1
Example 2
Example 3
Example 4
Example 5
Example 6 Important
Example 7
Example 8
Example 9
Example 10
Example 11
Example 12 You are here
Example 13
Example 14 Important
Example 15 Important
Example 16
Example 17 Important
Example 18 Important
Example 19 Important
Example 20
Example 21 Important
Example 22
Example 23 Important
Example 24 Important
Chapter 9 Class 11 Sequences and Series
Serial order wise
About the Author
Davneet Singh
Davneet Singh is a graduate from Indian Institute of Technology, Kanpur. He has been teaching from the past 8 years. He provides courses for Maths and Science at Teachoo. You can check his NCERT Solutions from Class 6 to 12.