Question 10 - Chapter 9 Class 11 Sequences and Series (Important Question)

Last updated at Dec. 16, 2024 by

Ex 9.4, 10 - Find sum of series, nth terms is (2n - 1)2 - Ex 9.4

Ex 9.4, 10 - Chapter 9 Class 11 Sequences and Series - Part 2
Ex 9.4, 10 - Chapter 9 Class 11 Sequences and Series - Part 3

Go Ad-free

Transcript

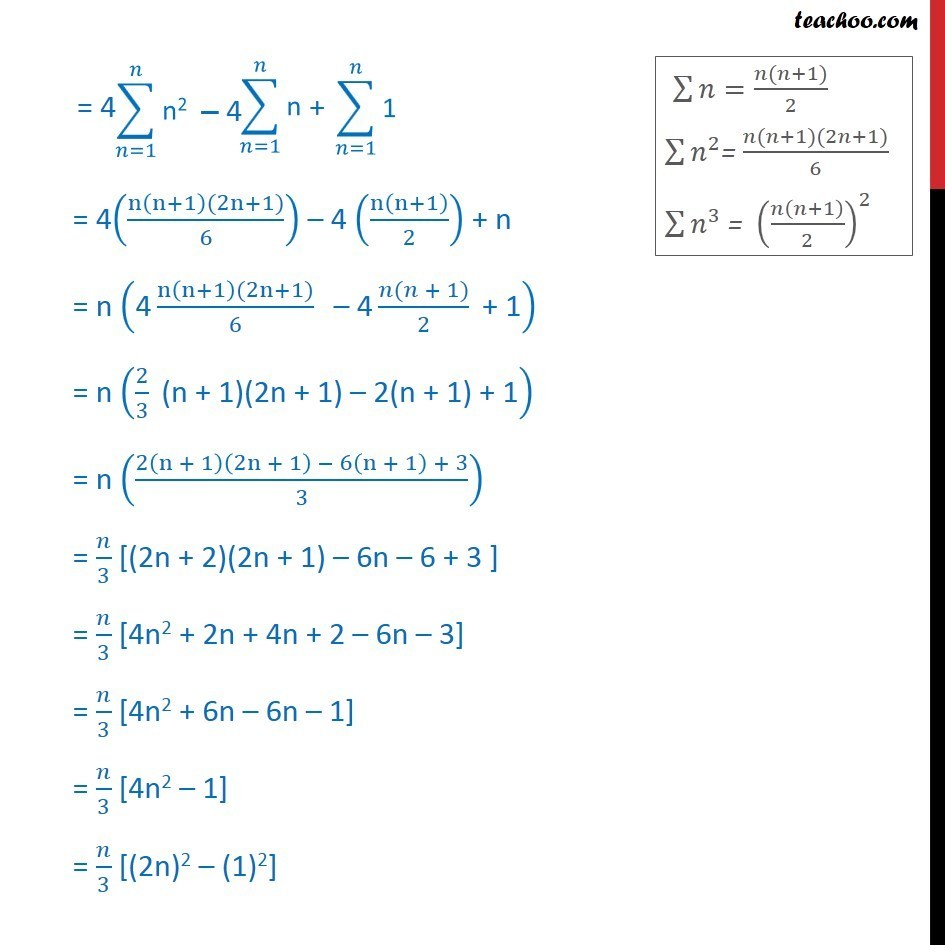
Question 10 Find the sum to n terms of the series whose nth terms is given by (2n 1)2 Given an = (2n 1)2 =(2n)2 + (1)2 2(2n)(1) = 4n2 + 1 4n = 4n2 4n + 1 Sum of n terms is = 4((n(n+1)(2n+1))/6) 4 (n(n+1)/2) + n = n ("4" (n(n+1)(2n+1))/6 " 4" ( ( + 1))/2 " + 1" ) = n (2/3 " (n + 1)(2n + 1) 2(n + 1) + 1" ) = n ((2(n + 1)(2n + 1) 6(n + 1) + 3)/3) = /3 [(2n + 2)(2n + 1) 6n 6 + 3 ] = /3 [4n2 + 2n + 4n + 2 6n 3] = /3 [4n2 + 6n 6n 1] = /3 [4n2 1] = /3 [(2n)2 (1)2] = /3 [(2n 1) (2n + 1)] Hence, the required sum is /3 [(2n 1 ) (2n + 1)]

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo