Ex 9.4.4 - Chapter 9 Class 11 Sequences and Series (Important Question)

Last updated at Dec. 16, 2024 by

Ex 9.4. 4 - Find sum of series 1/ 1 x 2 + 1/ 2 x 3 + 1/ 3 x 4 - Finding sum from nth number

Ex 9.4.4 - Chapter 9 Class 11 Sequences and Series - Part 2
Ex 9.4.4 - Chapter 9 Class 11 Sequences and Series - Part 3

Go Ad-free

Transcript

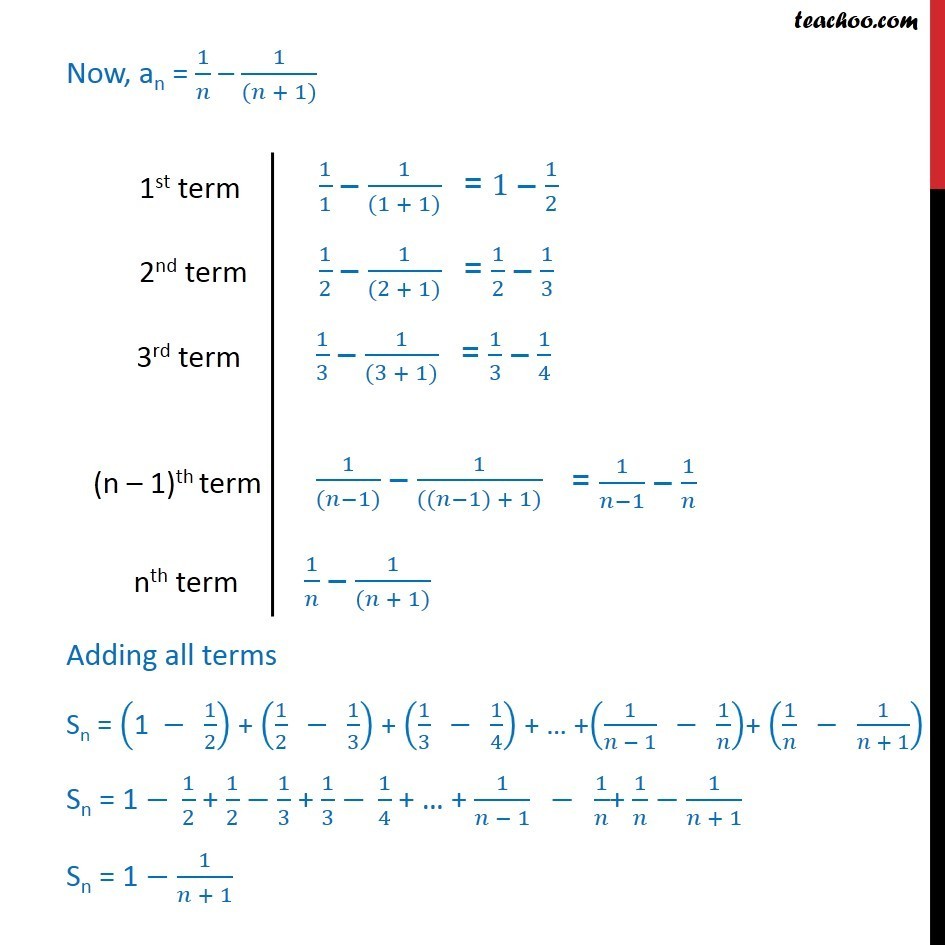
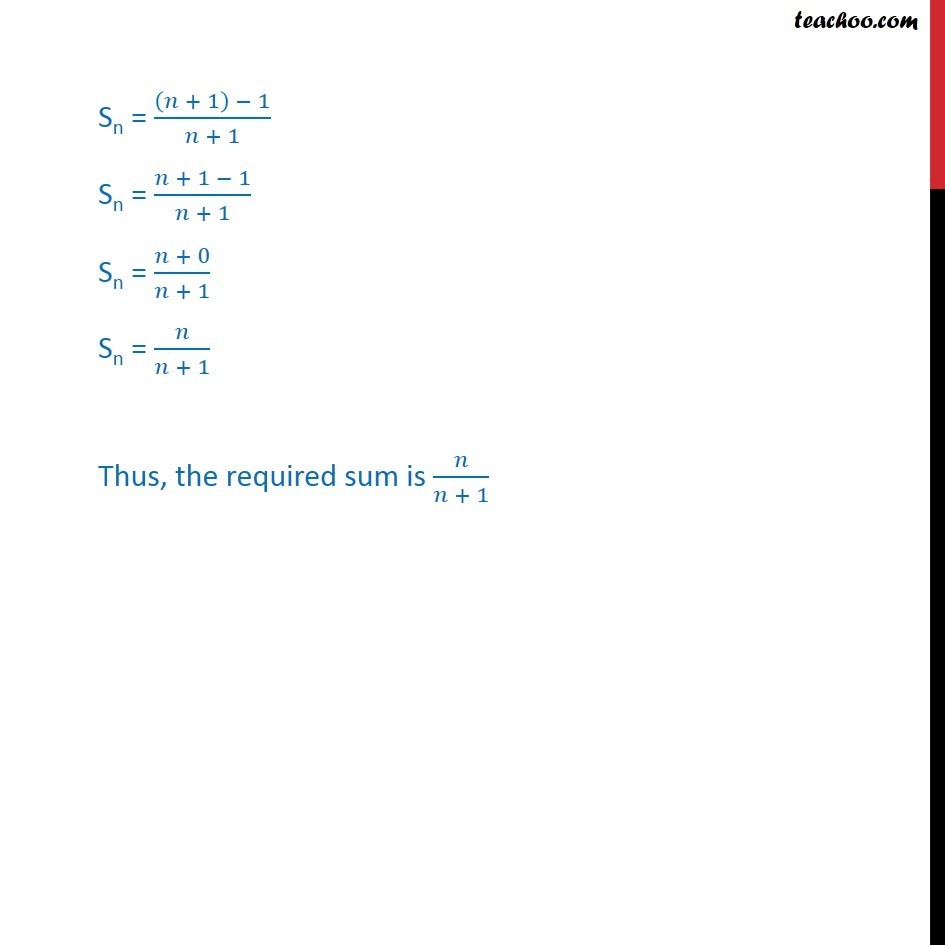
Question 4 Find the sum to n terms of the series 1/(1 2) + 1/(2 3) + 1/(3 4) + nth term of series 1/(1 2) + 1/(2 3) + 1/(3 4) + is 1/( ( + 1)) an = 1/( ( + 1)) = (( + 1) )/( ( + 1)) = (( + 1))/( ( + 1)) /( ( + 1)) = 1/ 1/(( + 1)) Now, an = 1/ 1/(( + 1)) Adding all terms Sn = ("1 " " " 1/2) + (1/2 " " " " 1/3) + (1/3 " " " " 1/4) + +(1/( 1) " " " " 1/ )+ (1/ " " " " 1/( + 1)) Sn = 1 1/2 + 1/2 1/3 + 1/3 1/4 + + 1/( 1) " " " " 1/ + 1/ 1/( + 1) Sn = 1 1/( + 1) Sn = (( + 1) 1)/( + 1) Sn = ( + 1 1)/( + 1) Sn = ( + 0)/( + 1) Sn = /( + 1) Thus, the required sum is /( + 1)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo