You are here
Ex 8.2, 24 - Chapter 8 Class 11 Sequences and Series
Last updated at Dec. 16, 2024 by Teachoo
Geometric Progression(GP): Calculation based/Proofs
Chapter 8 Class 11 Sequences and Series
Concept wise
- Finding Sequences
- Arithmetic Progression (AP): Formulae based
- Arithmetic Progression (AP): Statement
- Arithmetic Progression (AP): Calculation based/Proofs
- Inserting AP between two numbers
- Arithmetic Mean (AM)
- Geometric Progression(GP): Formulae based
- Geometric Progression(GP): Statement
-
Geometric Progression(GP): Calculation based/Proofs
- Inserting GP between two numbers
- Geometric Mean (GM)
- AM and GM (Arithmetic Mean And Geometric mean)
- AP and GP mix questions
- Finding sum from series
- Finding sum from nth number
Transcript
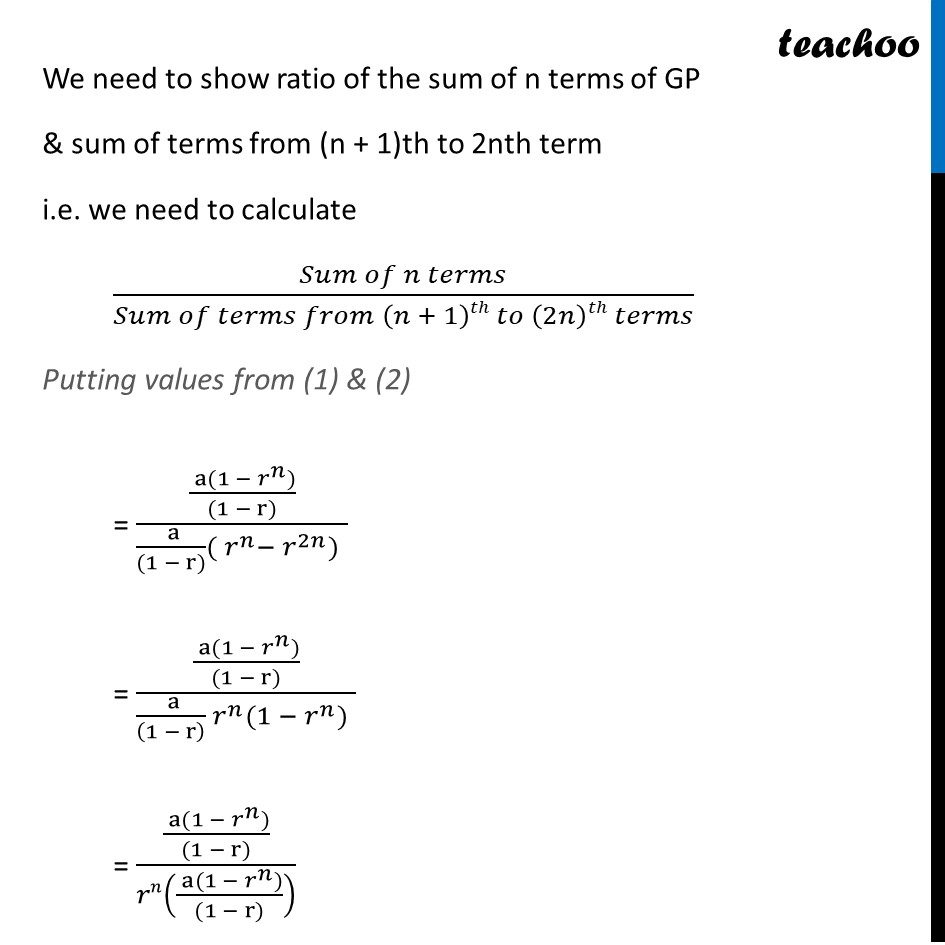
We need to show ratio of the sum of n terms of GP & sum of terms from (n + 1)th to 2nth term i.e. we need to calculate ( )/( ( + 1) (2 ) ) Putting values from (1) & (2) = (( a(1 ^ ))/((1 r)))/(( a)/((1 r))( ^ ^2 )" " ) = (( a(1 ^ ))/((1 r)))/(( a)/((1 r) ) ^ (1 ^ )" " ) = (( a(1 ^ ))/((1 r)))/ (( a(1 ^ ))/((1 r))) = ( a(1 ^ ))/(rn(1 ^ )) ( 1 r)/(1 r) Ex 8.2, 24 Show that the ratio of the sum of first n terms of a G.P. to the sum of terms from (n+1)th to (2n)th term is ( 1)/rn Ex 8.2, 24 Show that the ratio of the sum of first n terms of a G.P. to the sum of terms from (n+1)th to (2n)th term is ( 1)/rn We need to show ratio of the sum of n terms of GP & sum of terms from (n + 1)th to 2nth term i.e. we need to calculate ( )/( ( + 1) (2 ) ) Putting values from (1) & (2) = (( a(1 ^ ))/((1 r)))/(( a)/((1 r))( ^ ^2 )" " ) = (( a(1 ^ ))/((1 r)))/(( a)/((1 r) ) ^ (1 ^ )" " ) = (( a(1 ^ ))/((1 r)))/ (( a(1 ^ ))/((1 r))) = ( a(1 ^ ))/(rn(1 ^ )) ( 1 r)/(1 r) = 1/
