Ex 8.2, 9 - Chapter 8 Class 11 Sequences and Series
Last updated at Dec. 16, 2024 by Teachoo
Geometric Progression(GP): Formulae based
Ex 8.2, 6
You are here
You are here
Chapter 8 Class 11 Sequences and Series
Concept wise
- Finding Sequences
- Arithmetic Progression (AP): Formulae based
- Arithmetic Progression (AP): Statement
- Arithmetic Progression (AP): Calculation based/Proofs
- Inserting AP between two numbers
- Arithmetic Mean (AM)
-
Geometric Progression(GP): Formulae based
- Geometric Progression(GP): Statement
- Geometric Progression(GP): Calculation based/Proofs
- Inserting GP between two numbers
- Geometric Mean (GM)
- AM and GM (Arithmetic Mean And Geometric mean)
- AP and GP mix questions
- Finding sum from series
- Finding sum from nth number
Transcript
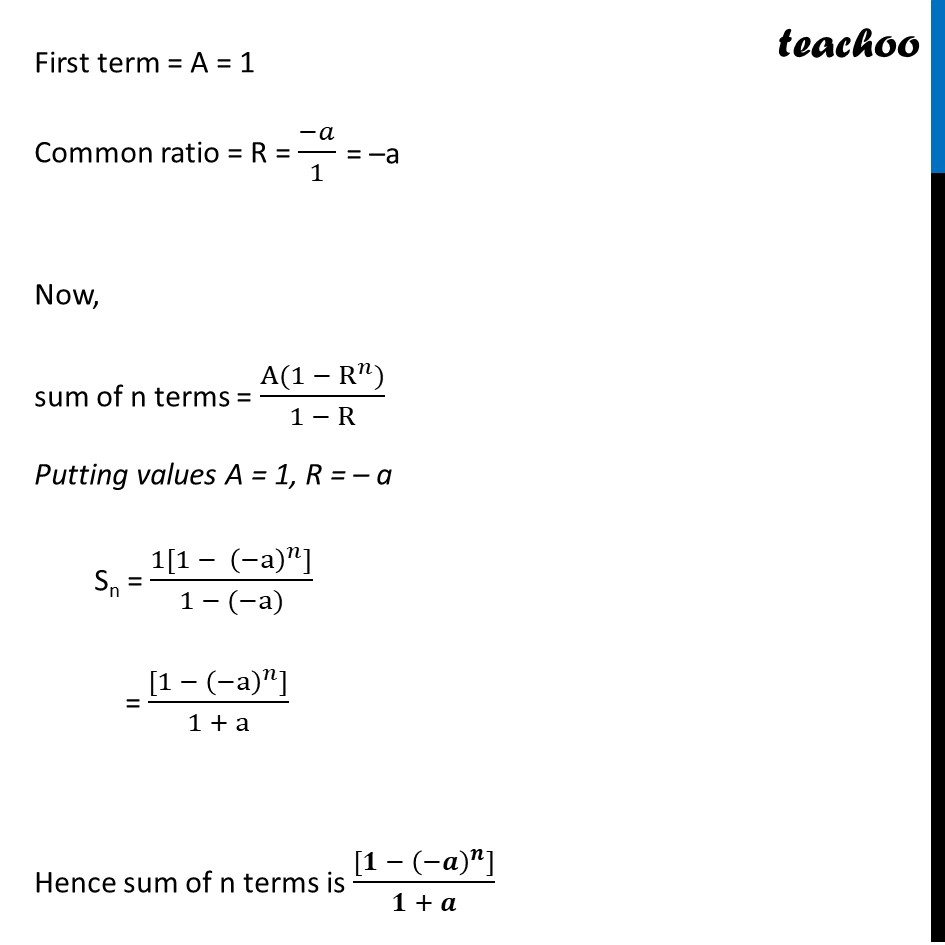
Ex 8.2, 9 Find the sum to n terms in the geometric progression 1, –a, a2, – a3….. (if a ≠ -1) 1, –a, a2, – a3….. Since small ‘a’ is used here, we used ‘A’ for first term We know that Sn = (A(1 − R^𝑛))/(1 − R) where Sn = sum of n terms of GP n is the number of terms A is the first term R is the common ratio First term = A = 1 Common ratio = R = (−𝑎)/1 Now, sum of n terms = (A(1 − R^𝑛))/(1 − R) Putting values A = 1, R = – a Sn = (1[1−(−a)^𝑛])/(1−(−a)) = ([1−(−a)^𝑛])/(1 + a) Hence sum of n terms is ([1−(−a)^𝑛])/(1 + a)
