Ex 8.2, 8 - Chapter 8 Class 11 Sequences and Series
Last updated at Dec. 16, 2024 by Teachoo
Geometric Progression(GP): Formulae based
Ex 8.2, 6
You are here
You are here
Chapter 8 Class 11 Sequences and Series
Concept wise
- Finding Sequences
- Arithmetic Progression (AP): Formulae based
- Arithmetic Progression (AP): Statement
- Arithmetic Progression (AP): Calculation based/Proofs
- Inserting AP between two numbers
- Arithmetic Mean (AM)
-
Geometric Progression(GP): Formulae based
- Geometric Progression(GP): Statement
- Geometric Progression(GP): Calculation based/Proofs
- Inserting GP between two numbers
- Geometric Mean (GM)
- AM and GM (Arithmetic Mean And Geometric mean)
- AP and GP mix questions
- Finding sum from series
- Finding sum from nth number
Transcript
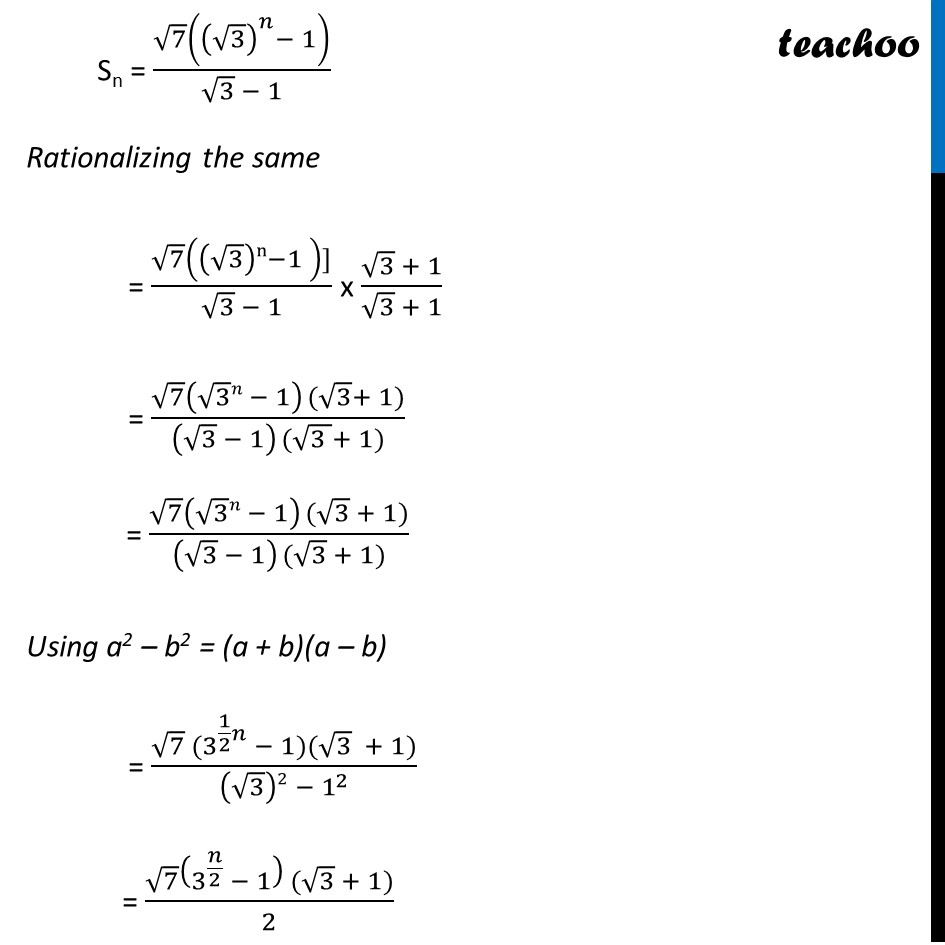
Ex 8.2, 8 Find the sum to n terms in the geometric progression 7 , 21 ,3 7 7 , 21 ,3 7 Here, First term a = 7 Common ratio r = 21/ 7 = (7 3)/ 7 = ( 7 3 )/ 7 = 3 So r = 3 1.73 Since, r > 1 Sn = ( ( ^ 1))/( 1) Sn = ( ( ^ 1))/( 1) where Sn = sum of n terms of GP n is the number of terms a is the first term r is the common ratio Now, Sum of n terms = ( ( ^ 1))/( 1) Putting values a = 7 , r = 3 Sn = ( 7 (( 3)^ 1))/( 3 1) Rationalizing the same = ( 7 (( 3)n 1 )])/( 3 1) x ( 3 + 1)/( 3 + 1) = ( 7 ( 3 1) ( 3+ 1))/(( 3 1) ( 3+ 1)) = ( 7 ( 3 1) ( 3+ 1))/(( 3 1) ( 3+ 1)) Using a2 b2 = (a + b)(a b) = ( 7 (3^(1/2 ) 1)( 3 +1))/(( 3)2 1^2 ) =( 7 (3^( /2) 1) ( 3 + 1))/2 = 7/2( 3+1) (3^( /2) 1) Hence sum of n term is 7/2( 3+1) (3^( /2) 1)
