Ex 8.2, 6 - Chapter 8 Class 11 Sequences and Series
Last updated at April 16, 2024 by Teachoo
Geometric Progression(GP): Formulae based
Ex 8.2, 6
You are here
You are here
Chapter 8 Class 11 Sequences and Series
Concept wise
- Finding Sequences
- Arithmetic Progression (AP): Formulae based
- Arithmetic Progression (AP): Statement
- Arithmetic Progression (AP): Calculation based/Proofs
- Inserting AP between two numbers
- Arithmetic Mean (AM)
-
Geometric Progression(GP): Formulae based
- Geometric Progression(GP): Statement
- Geometric Progression(GP): Calculation based/Proofs
- Inserting GP between two numbers
- Geometric Mean (GM)
- AM and GM (Arithmetic Mean And Geometric mean)
- AP and GP mix questions
- Finding sum from series
- Finding sum from nth number
Transcript
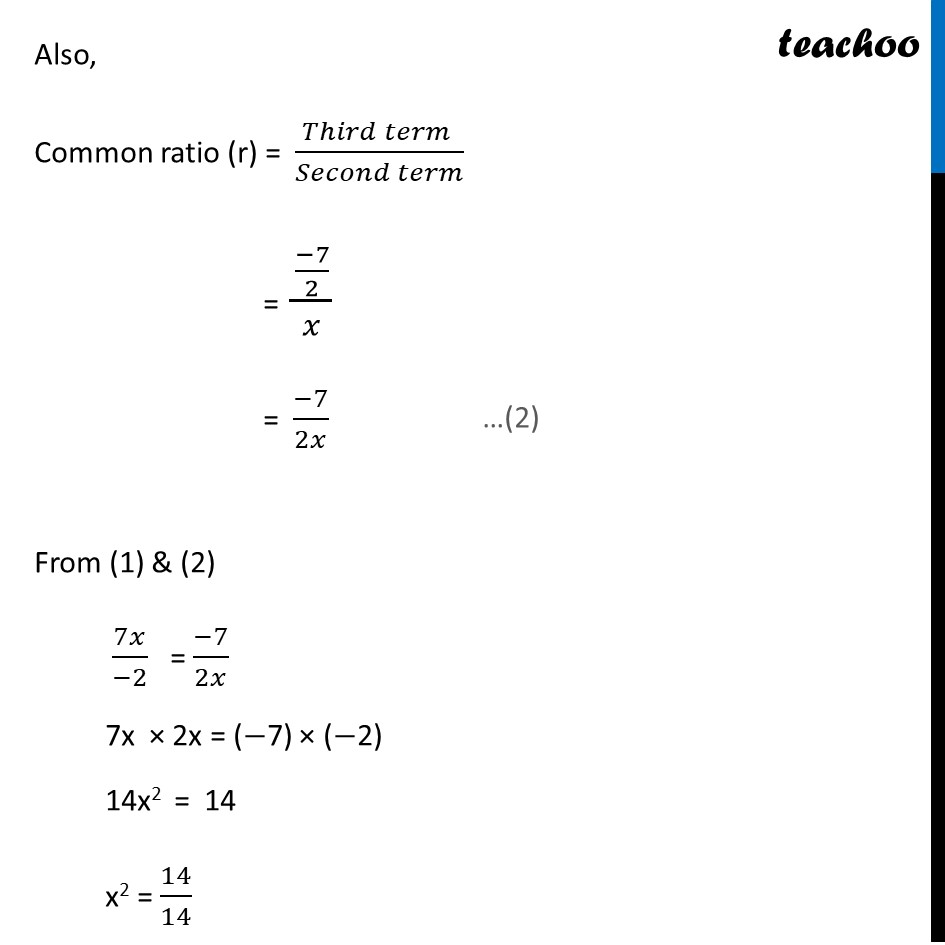
Ex 8.2,6 For what values of x, the numbers (−2)/7, x, (−7)/2 are in G.P? Since (−2)/7, x, (−7)/2 are in GP So common ratio will be same Common ratio (r) = (𝑆𝑒𝑐𝑜𝑛𝑑 𝑡𝑒𝑟𝑚 )/(𝐹𝑖𝑟𝑠𝑡 𝑡𝑒𝑟𝑚) = 𝑥/((−2)/7) = 7𝑥/(−2) Also, Common ratio (r) = (𝑇ℎ𝑖𝑟𝑑 𝑡𝑒𝑟𝑚 )/(𝑆𝑒𝑐𝑜𝑛𝑑 𝑡𝑒𝑟𝑚) = ((−7)/2)/𝑥 = (−7)/2𝑥 From (1) & (2) 7𝑥/(−2) = (−7)/2𝑥 7x × 2x = (-7) × (-2) 14x2 = 14 x2 = 14/14 x2 = 1 x = ± √1 x = ±1 So, x = 1 or x = -1 GP is (−2)/7, x, (−7)/2 For x = 1, GP is (−2)/7, 1, (−7)/2 For x = –1, GP is (−2)/7, –1, (−7)/2 Hence possible numbers in GP are (−2)/7, 1, (−7)/2 or (−2)/7, –1, (−7)/2
