Question 38 (ii) - CBSE Class 12 Sample Paper for 2025 Boards - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Transcript

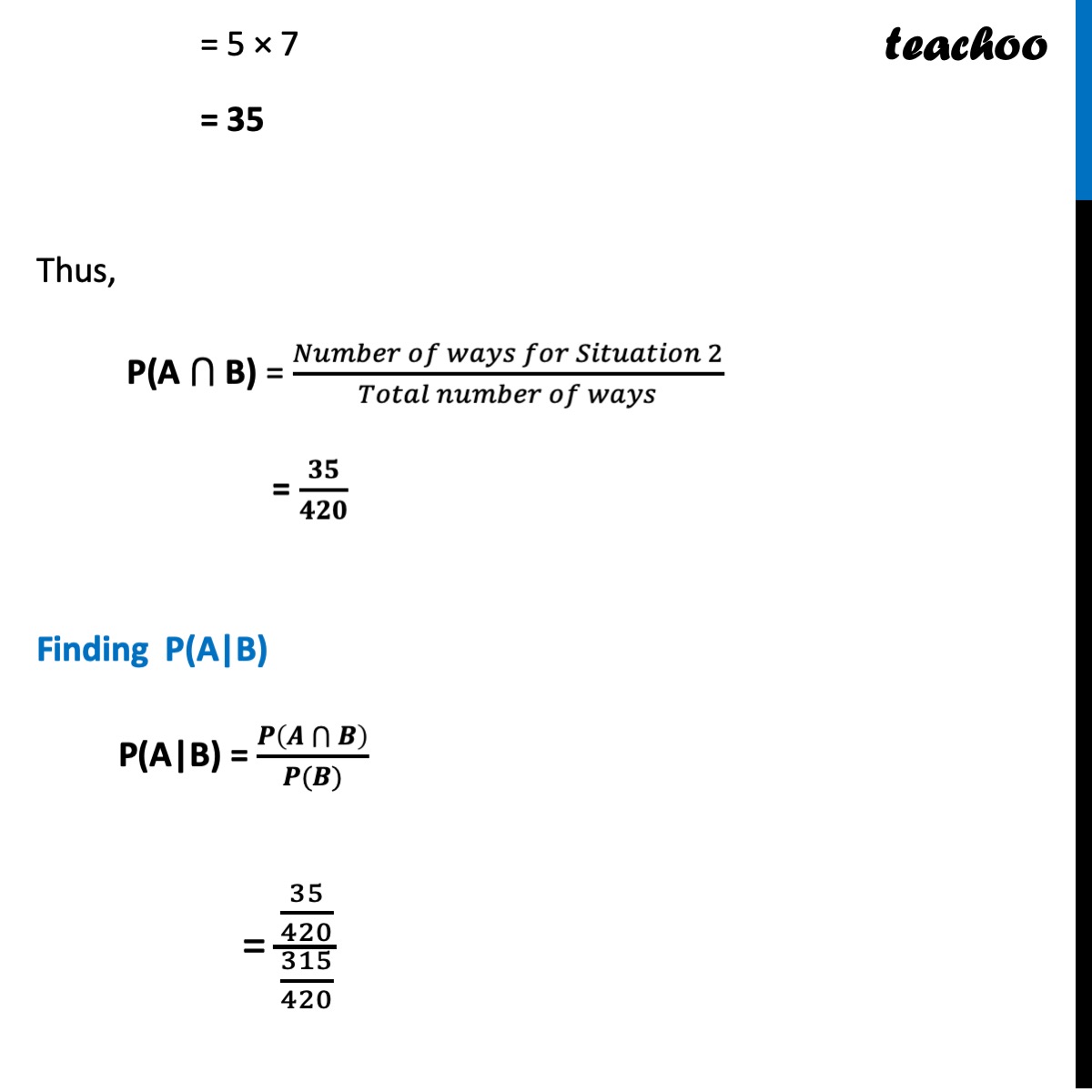
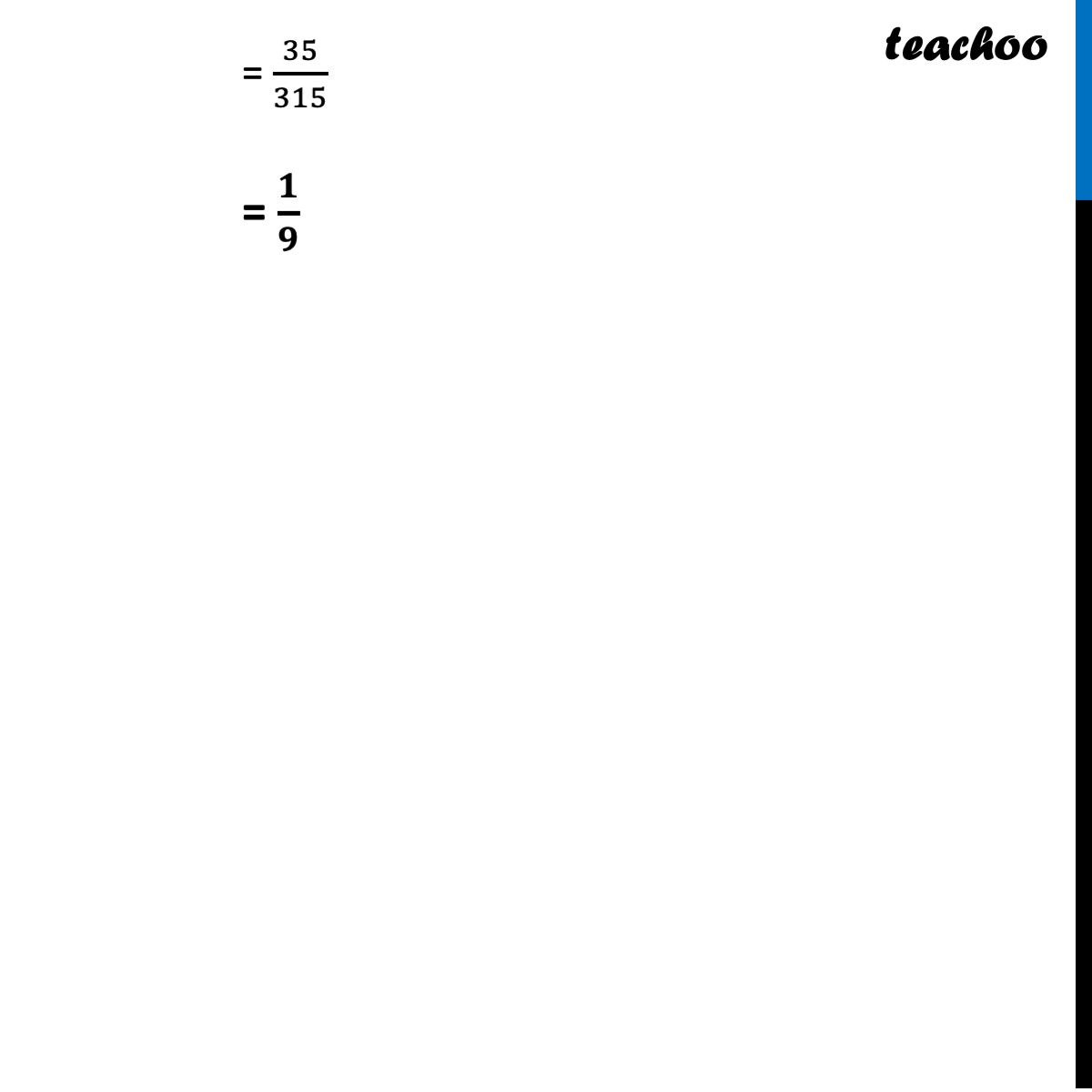
Question 38 (Case Based Questions) Arka bought two cages of birds: Cage-I contains 5 parrots and 1 owl and Cage -II contains 6 parrots. One day Arka forgot to lock both cages and two birds flew from Cage-I to Cage-II (simultaneously). Then two birds flew back from cage-II to cage-I(simultaneously). Assume that all the birds have equal chances of flying. Question 38 (ii) When two birds flew from Cage-I to Cage-II and two birds flew back from Cage-II to Cage-I, the owl is still seen in Cage-I, what is the probability that one parrot and the owl flew from Cage-I to Cage-II? Since we already know that owl is in Cage 1, and then finding probability that one parrot and the owl flew from Cage-I to Cage-II So, We know an event has happened, and now finding probability. Thus, we use Bayes Theorem Let A be the event that “1 parrot & the owl flew from Cage I to Cage II” Let B be the event that “the owl is still in Cage I after the entire process.” We want to find the conditional probability P(A|B) , i.e., the probability that one parrot and the owl flew from Cage I to Cage II, given that the owl is still in Cage I after the entire process. Bayes’ Theorem gives us the formula: P(A|B) = 𝑷(𝑨⋂▒𝑩)/(𝑷(𝑩)) Where: P(B) is the probability that the owl is still in Cage I after the process. P(A ⋂ B) is the probability that one parrot and the owl flew from Cage I to Cage II AND that owl is still in Cage 1 i.e. P(A ⋂ B) is the probability that one parrot and the owl flew from Cage I to Cage II AND owl flew black Let’s calculate both Finding P(B) The total probability that the owl is still in Cage I after the process We calculated this in part (i) P(B) = 𝟑𝟏𝟓/𝟒𝟐𝟎 Finding P(A ⋂ B) P(A ⋂ B) is the probability that one parrot and the owl flew from Cage I to Cage II AND owl flew black This is same as situation 2 The owl flies to Cage II in the first move but returns to Cage I in the second move. Here, Since owl is flying from Cage 1, we choose 1 more bird We choose 1 birds out of remaining 5 birds from Cage 1 Since 2 birds have flown to Cage 2, Cage 2 has 6+2 = 8 birds now Since owl is flying back We choose 1 bird out of remaining 7 birds from Cage 2 Number of ways for Situation 2 = Choosing 1 birds out of remaining 5 birds × Choosing 1 birds out of 7 birds = 5C1 × 7C1 = 5 × 7 = 35 Thus, P(A ⋂ B) = (𝑁𝑢𝑚𝑏𝑒𝑟 𝑜𝑓 𝑤𝑎𝑦𝑠 𝑓𝑜𝑟 𝑆𝑖𝑡𝑢𝑎𝑡𝑖𝑜𝑛 2)/(𝑇𝑜𝑡𝑎𝑙 𝑛𝑢𝑚𝑏𝑒𝑟 𝑜𝑓 𝑤𝑎𝑦𝑠) = 𝟑𝟓/𝟒𝟐𝟎 Finding P(A|B) P(A|B) = 𝑷(𝑨⋂▒𝑩)/(𝑷(𝑩)) = (35/420)/(315/420) = 35/315 = 𝟏/𝟗