Question 37 (iii) (B) - If the track of the final race (for biker b1)

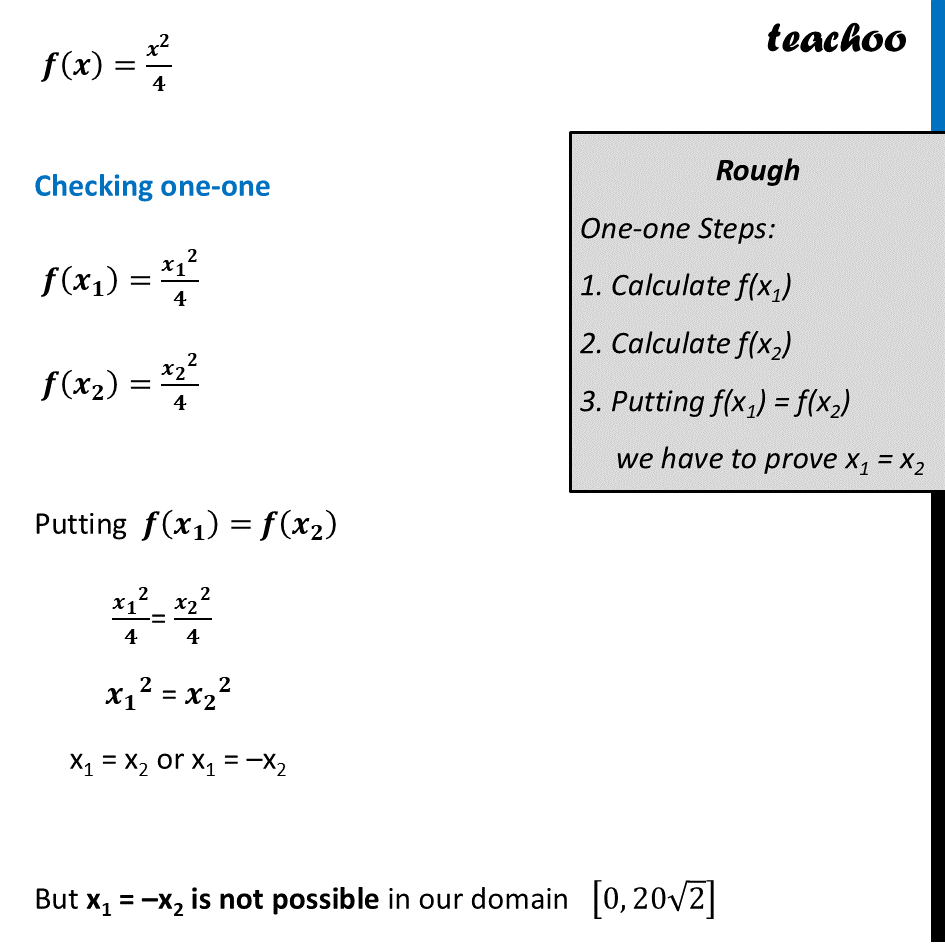
Question 37 (iii) (B) If the track of the final race (for the biker 𝑏_1) follows the curve 𝑥^2=4𝑦; ( where 0≤𝑥≤20√2. & 0≤𝑦≤200), then state whether the track represents a one-one and onto function or not. (Justify).Given 𝑥^2=4𝑦 𝑦=𝑥^2/4 Let 𝑦=𝒇(𝒙)=𝒙^𝟐/𝟒 In [0, 20√2]→[0, 200] 𝒇(𝒙)=𝒙^𝟐/𝟒 Checking one-one 𝒇(𝒙_𝟏 )=〖𝒙_𝟏〗^𝟐/𝟒 𝒇(𝒙_𝟐 )=〖𝒙_𝟐〗^𝟐/𝟒 Putting 𝒇(𝒙_𝟏 )= 𝒇(𝒙_𝟐 ) 〖𝒙_𝟏〗^𝟐/𝟒= 〖𝒙_𝟐〗^𝟐/𝟒 〖𝒙_𝟏〗^𝟐 = 〖𝒙_𝟐〗^𝟐 x1 = x2 or x1 = –x2 But x1 = –x2 is not possible in our domain [0, 20√2] Rough One-one Steps: 1. Calculate f(x1) 2. Calculate f(x2) 3. Putting f(x1) = f(x2) we have to prove x1 = x2 So, x1 = x2 when f (x1) = f (x2) ∴ f(x) is one-one Check onto 𝒇(𝒙)=𝒙^𝟐/𝟒 Let f(x) = y , such that y ∈ R 𝑥^2/4=𝑦 𝑥^2=4𝑦 𝑥=±2√𝑦 Since 0≤𝑦≤200 and where 0≤𝑥≤20√2 For all values of y in [0, 200], there is a corresponding value of x in [0, 20√2] Thus, for every y in [0, 200], there exists a pre-image in [0, 20√2] ∴ f(x) is onto Thus, for every y in [0, 200], there exists a pre-image in [0, 20√2] ∴ f(x) is onto Let f(x) = y , such that y ∈ R x2 = y x = ±√𝑦 Note that y is a real number, so it can be negative also Putting y = −3 x = ±√((−3)) 𝑤ℎ𝑖𝑐ℎ 𝑖𝑠 𝑛𝑜𝑡 𝑝𝑜𝑠𝑠𝑖𝑏𝑙𝑒 𝑎𝑠 𝑟𝑜𝑜𝑡 𝑜𝑓 𝑛𝑒𝑔𝑎𝑡𝑖𝑣𝑒 𝑛𝑢𝑚𝑏𝑒𝑟 𝑖𝑠 𝑛𝑜𝑡 𝑟𝑒𝑎𝑙 Hence, x is not real So, f is not onto

Question 37 (iii) (B) - CBSE Class 12 Sample Paper for 2025 Boards - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Davneet Singh

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular