CBSE Class 12 Sample Paper for 2025 Boards

CBSE Class 12 Sample Paper for 2024 Boards →
Class 12
Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Question 36 (iii) (A) - CBSE Class 12 Sample Paper for 2025 Boards - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Last updated at Oct. 3, 2024 by

Slide50.PNG

Slide51.PNG
Slide52.PNG

 

Go Ad-free

Transcript

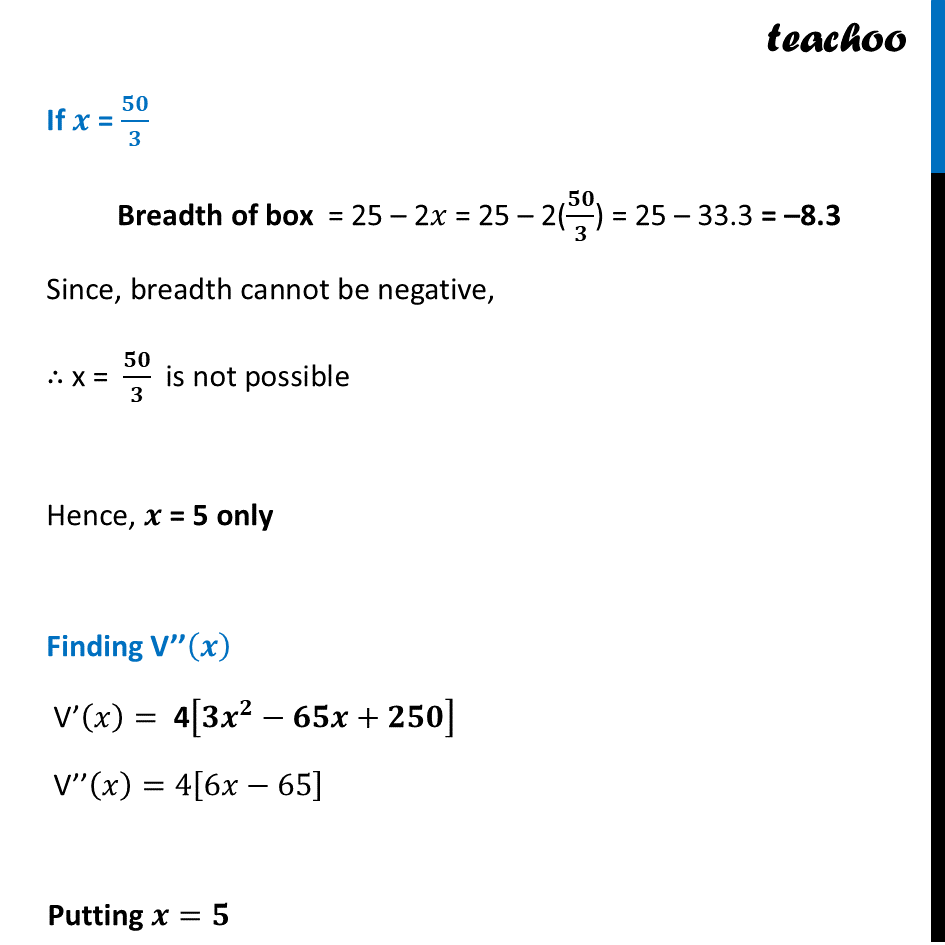
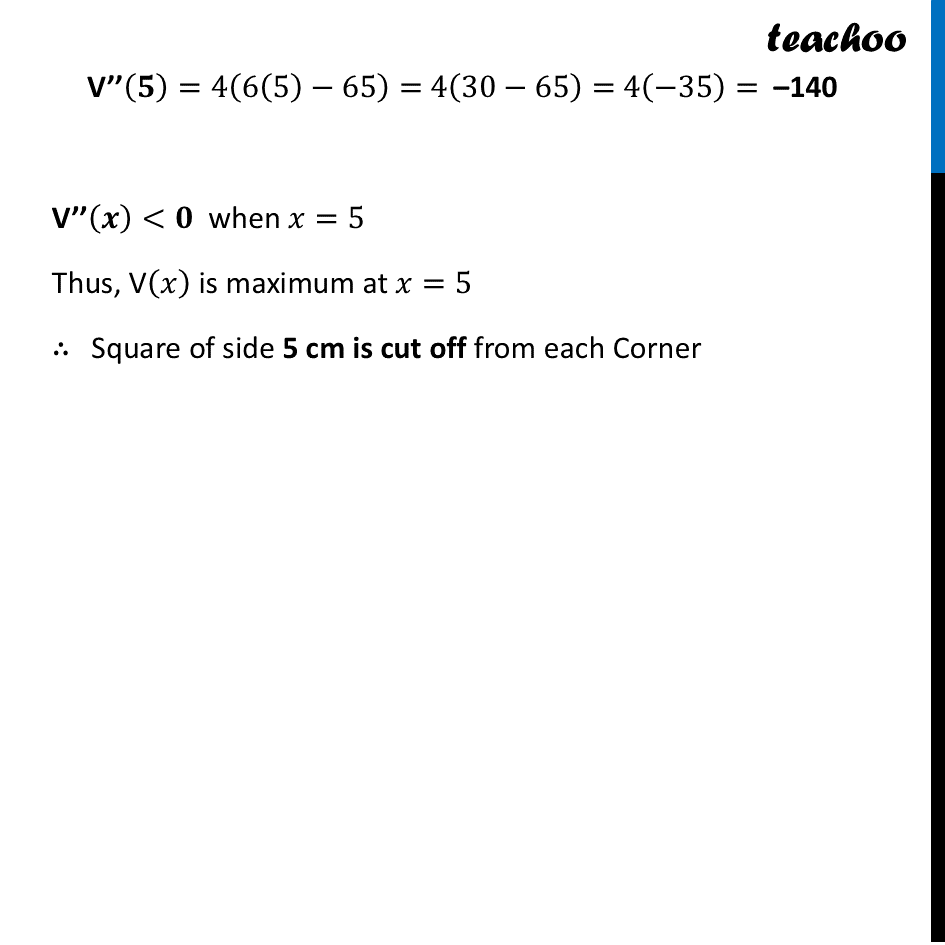
Question 36 (iii) (A) For what value of 𝒙, the volume of each container is maximum?Now, V = 2(2𝑥^3−65𝑥^2+500𝑥) And, 𝐝𝐕/𝒅𝒙= 4(𝑥−5)(3𝑥−50) Putting 𝐝𝐕/𝒅𝒙= 0 4(𝑥−5)(3𝑥−50)=0 So, x = 5 and x = 𝟓𝟎/𝟑 If 𝒙 = 𝟓𝟎/𝟑 Breadth of box = 25 – 2𝑥 = 25 – 2(𝟓𝟎/𝟑) = 25 – 33.3 = –8.3 Since, breadth cannot be negative, ∴ x = 𝟓𝟎/𝟑 is not possible Hence, 𝒙 = 5 only Finding V’’(𝒙) V’(𝑥)=" 4" [𝟑𝒙^𝟐−𝟔𝟓𝒙+𝟐𝟓𝟎] V’’(𝑥)=4[6𝑥−65] Putting 𝒙=𝟓 V’’(𝟓)=4(6(5)−65)= 4(30−65)= 4(−35)= –140 V’’(𝒙)<𝟎 when 𝑥=5 Thus, V(𝑥) is maximum at 𝑥=5 ∴ Square of side 5 cm is cut off from each Corner

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.