CBSE Class 12 Sample Paper for 2025 Boards

CBSE Class 12 Sample Paper for 2024 Boards →
Class 12
Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Question 31 (B) - CBSE Class 12 Sample Paper for 2025 Boards - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Last updated at Feb. 13, 2025 by

Slide7.PNG

Slide8.PNG
Slide9.PNG
Slide10.PNG
Slide11.PNG Slide12.PNG

 

Share on WhatsApp

Transcript

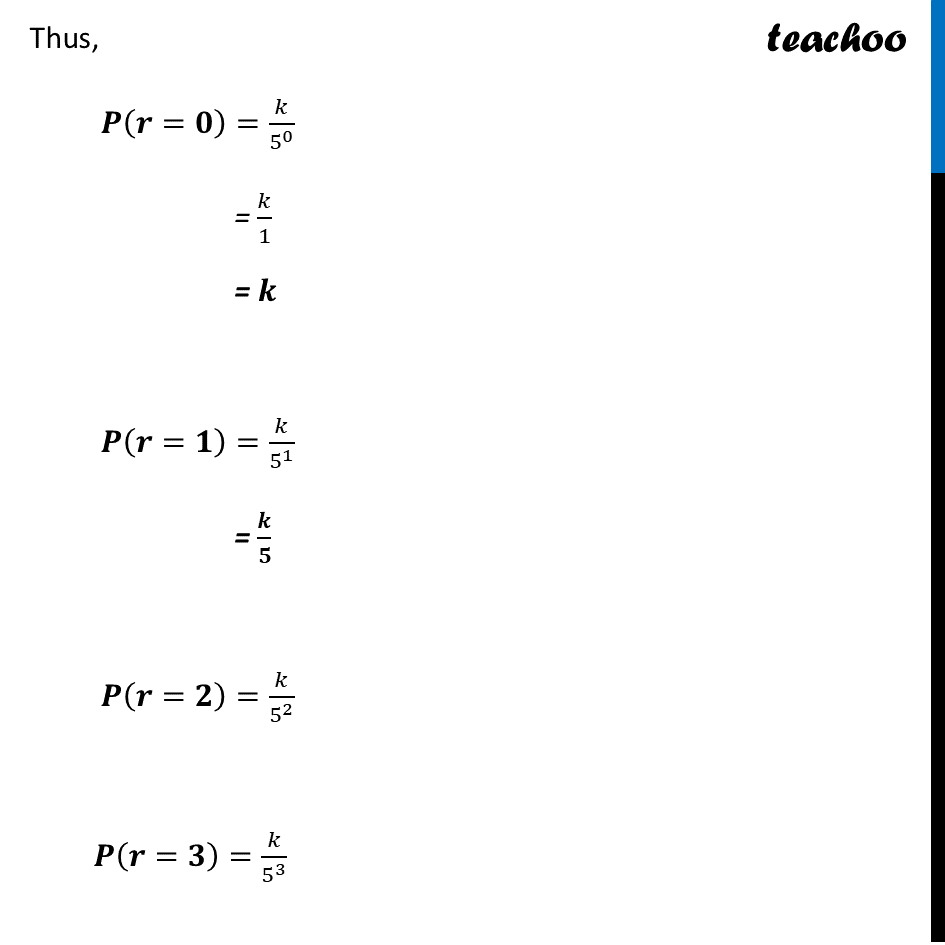
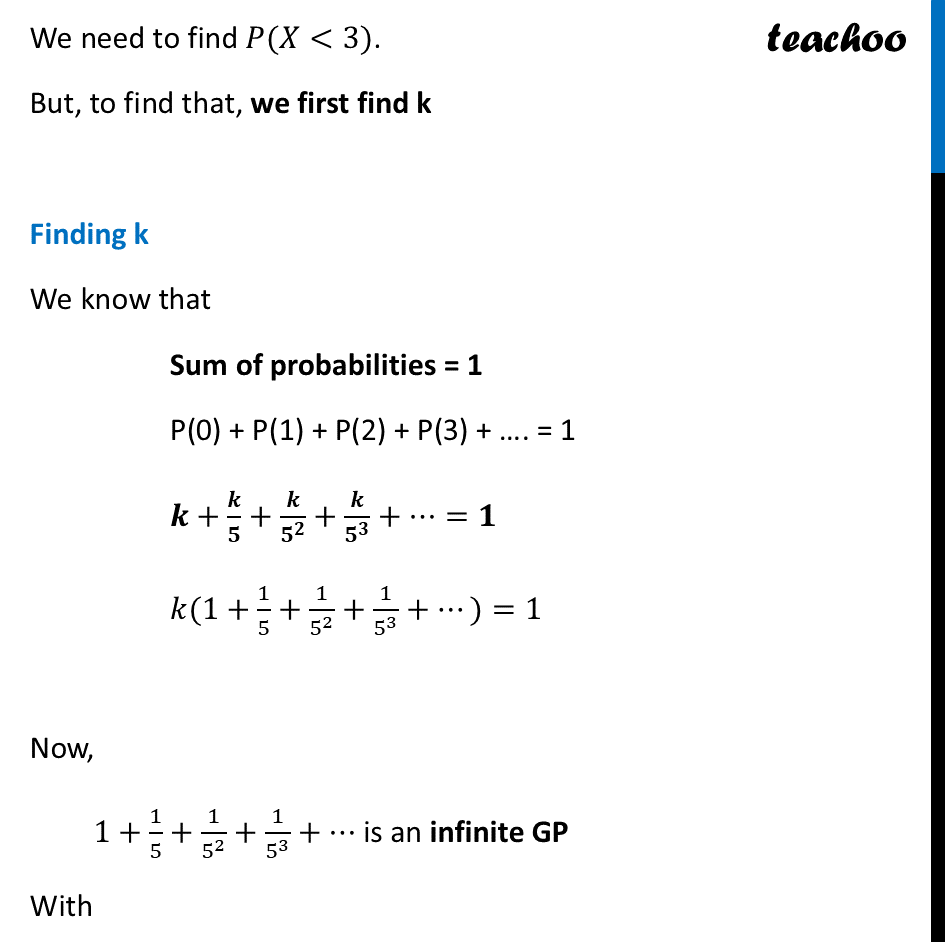
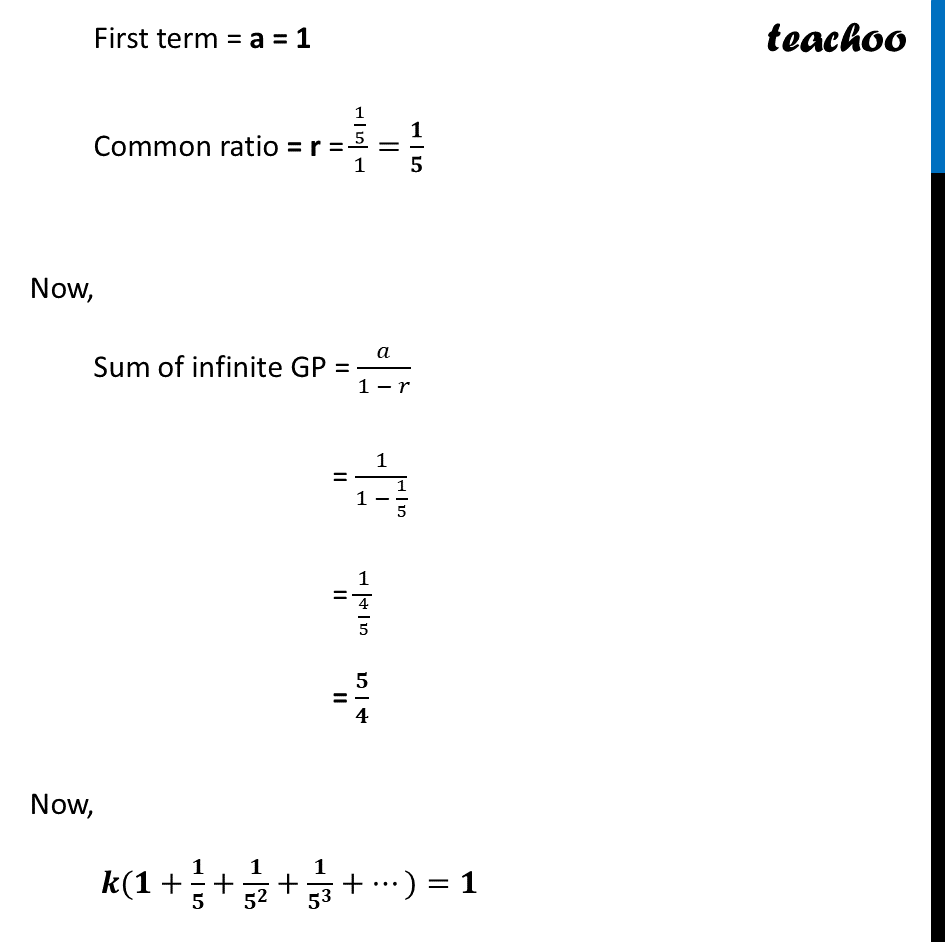
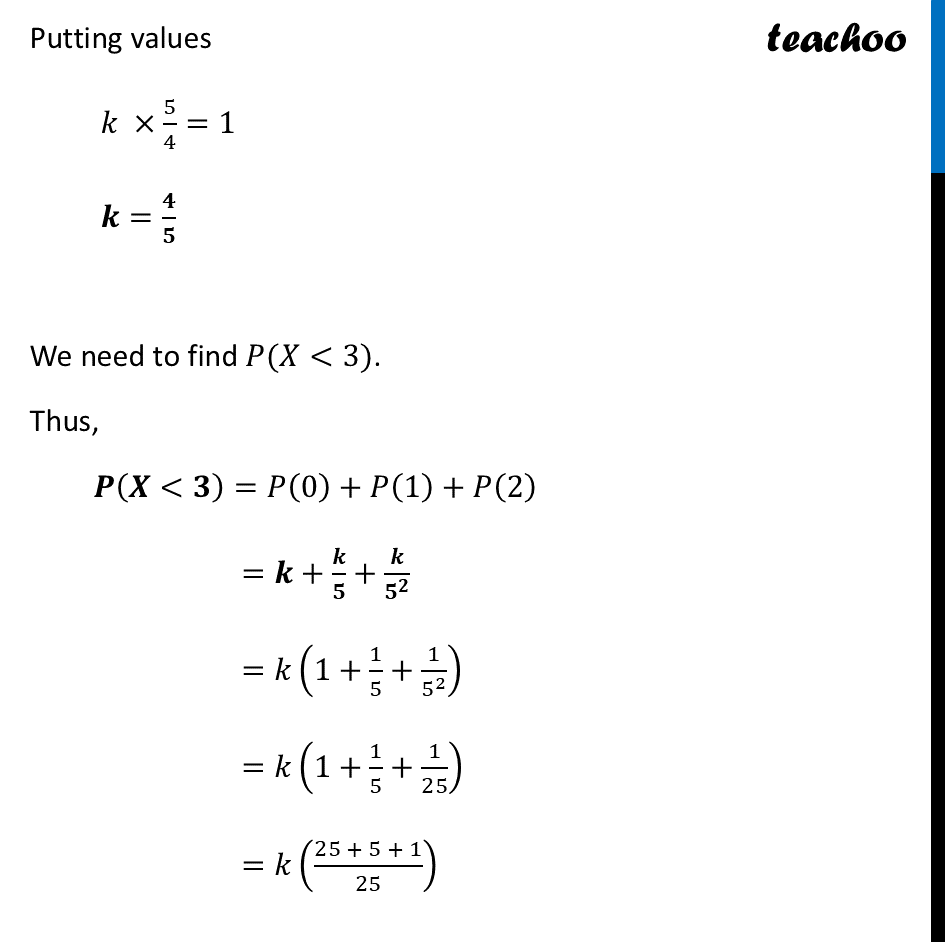
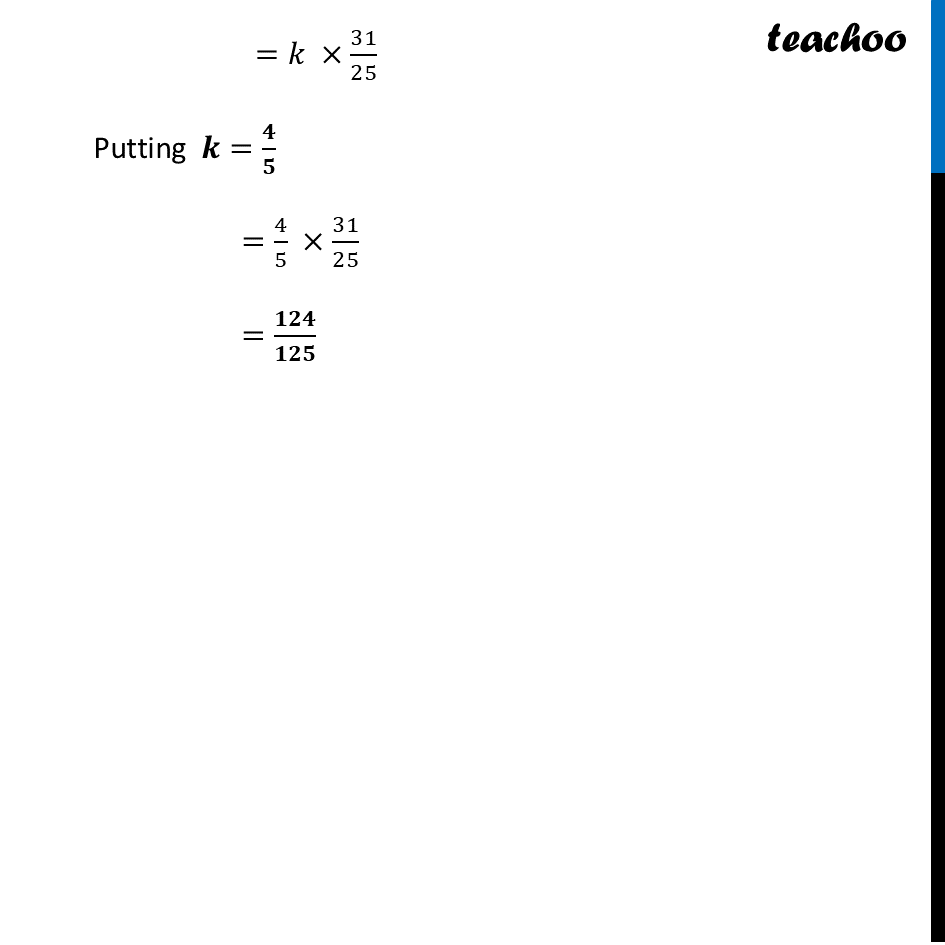
Question 31 (B) A random variable 𝑋 can take all non - negative integral values and the probability that 𝑋 takes the value π‘Ÿ is proportional to 5^(βˆ’π‘Ÿ). Find 𝑃(𝑋<3).It’s given that P(X = r) is proportional to 5^(βˆ’π‘Ÿ) i.e. P(X = r) ∝ 5^(βˆ’π‘Ÿ) So, we can write P(X = r) = k 5^(βˆ’π‘Ÿ) = π’Œ/πŸ“^𝒓 Where k is some constant Thus, 𝑷(𝒓=𝟎)=π‘˜/5^0 = π‘˜/1 = π’Œ 𝑷(𝒓=𝟏)=π‘˜/5^1 = π’Œ/πŸ“ 𝑷(𝒓=𝟐)=π‘˜/5^2 𝑷(𝒓=πŸ‘)=π‘˜/5^3 First term = a = 1 Common ratio = r = (1/5)/1=𝟏/πŸ“ Now, Sum of infinite GP = π‘Ž/(1 βˆ’ π‘Ÿ) = 1/(1 βˆ’ 1/5) = 1/(4/5) = πŸ“/πŸ’ Now, π’Œ(𝟏+𝟏/πŸ“+𝟏/πŸ“^𝟐 +𝟏/πŸ“^πŸ‘ +…)=𝟏 Putting values π‘˜ Γ—5/4=1 π’Œ=πŸ’/πŸ“ We need to find 𝑃(𝑋<3). Thus, 𝑷(𝑿<πŸ‘)=𝑃(0)+𝑃(1)+𝑃(2) =π’Œ+π’Œ/πŸ“+π’Œ/πŸ“^𝟐 =π‘˜(1+1/5+1/5^2 ) =π‘˜(1+1/5+1/25) =π‘˜((25 + 5 + 1)/25) =π‘˜ Γ—31/25 Putting π’Œ=πŸ’/πŸ“ =4/5 Γ—31/25 =πŸπŸπŸ’/πŸπŸπŸ“

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 15 years. He provides courses for Maths, Science and Computer Science at Teachoo