CBSE Class 12 Sample Paper for 2025 Boards
You are here
CBSE Class 12 Sample Paper for 2025 Boards
Last updated at Feb. 13, 2025 by Teachoo
This question is similar to Chapter 11 Class 12 Three Dimensional Geometry - Miscellaneous
Please check the question here
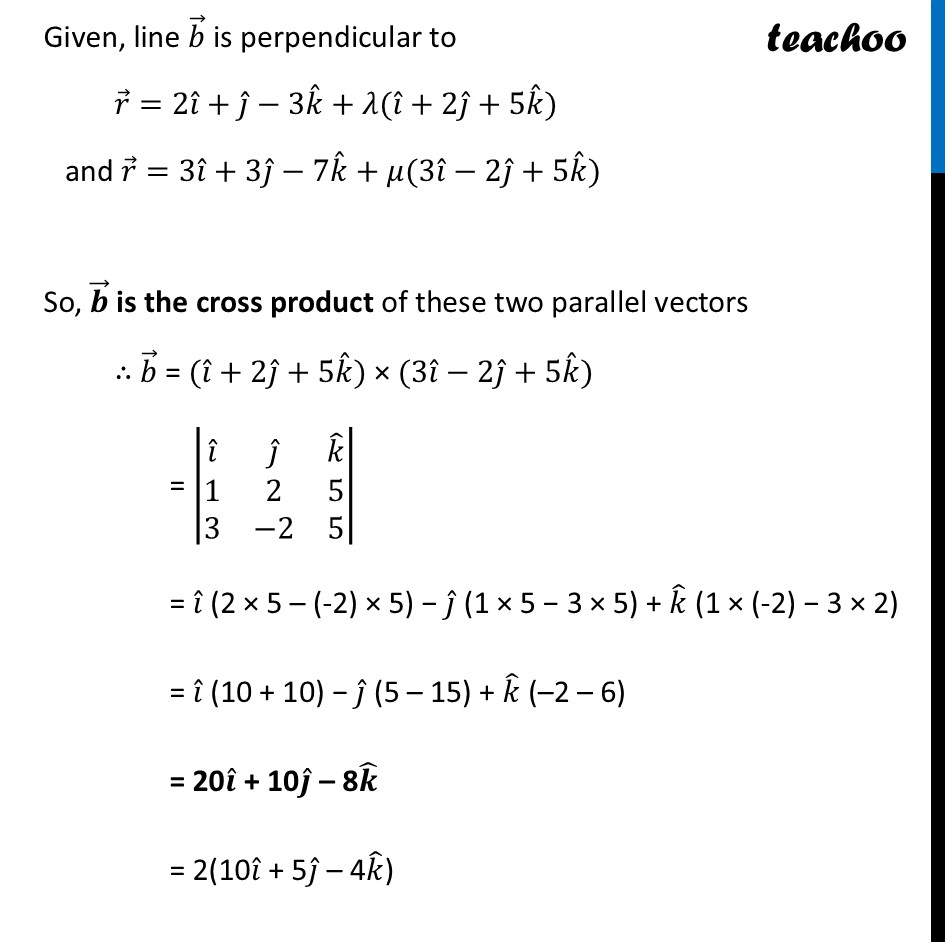
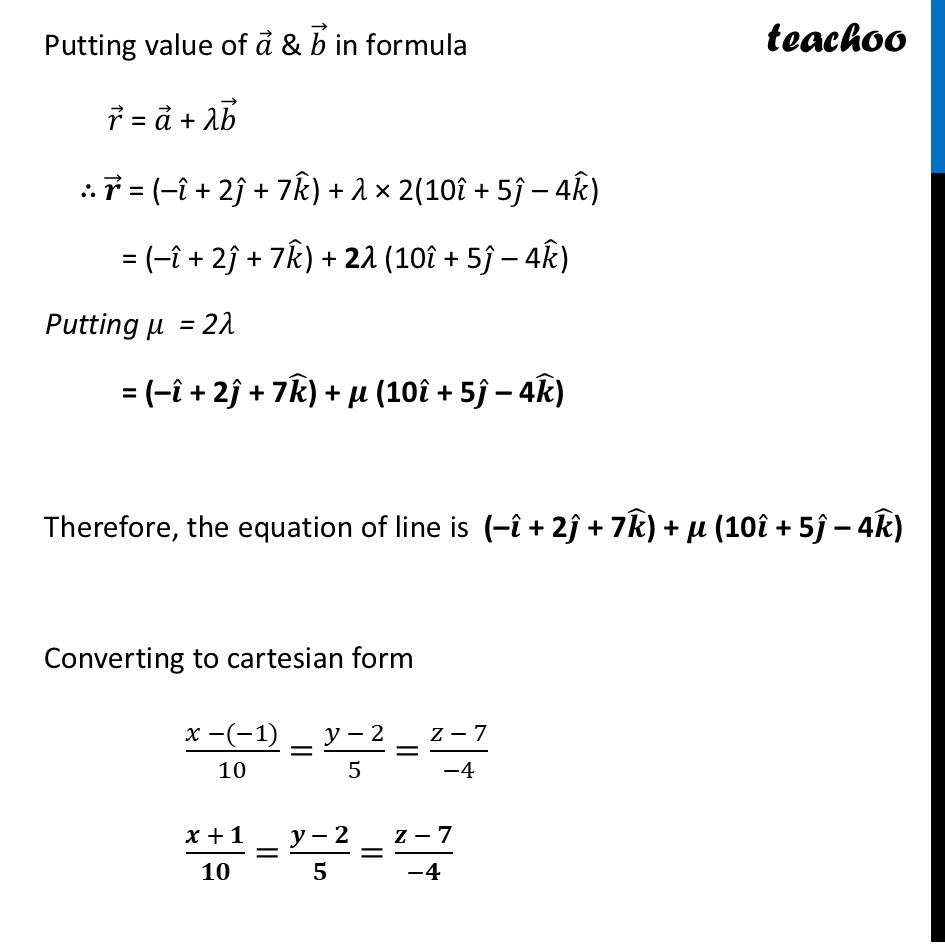
Transcript
Question 28 (B) Find the vector and the cartesian equation of the line that passes through (−1, 2, 7) and is perpendicular to the lines 𝑟 ⃗=2ı ˆ+ȷ ˆ−3𝑘 ˆ+𝜆(ı ˆ+2ȷ ˆ+5𝑘 ˆ) and 𝑟 ⃗=3ı ˆ+3ȷ ˆ−7𝑘 ˆ+𝜇(3ı ˆ−2ȷ ˆ+5𝑘 ˆ).The vector equation of a line passing through a point with position vector 𝑎 ⃗ and parallel to a vector 𝑏 ⃗ is 𝒓 ⃗ = 𝒂 ⃗ + 𝜆𝒃 ⃗ The line passes through (–1, 2, 7) So, 𝒂 ⃗ = –1𝒊 ̂ + 2𝒋 ̂ + 7𝒌 ̂ Now, finding 𝑏 ⃗ Given, line 𝑏 ⃗ is perpendicular to 𝑟 ⃗=2𝚤 ˆ+𝚥 ˆ−3𝑘 ˆ+𝜆(𝚤 ˆ+2𝚥 ˆ+5𝑘 ˆ) and 𝑟 ⃗=3𝚤 ˆ+3𝚥 ˆ−7𝑘 ˆ+𝜇(3𝚤 ˆ−2𝚥 ˆ+5𝑘 ˆ) So, 𝒃 ⃗ is the cross product of these two parallel vectors ∴ 𝑏 ⃗ = (𝚤 ˆ+2𝚥 ˆ+5𝑘 ˆ) × (3𝚤 ˆ−2𝚥 ˆ+5𝑘 ˆ) = |■8(𝑖 ̂&𝑗 ̂&𝑘 ̂@1&2&5@3&−2&5)| = 𝑖 ̂ (2 × 5 – (-2) × 5) − 𝑗 ̂ (1 × 5 − 3 × 5) + 𝑘 ̂ (1 × (-2) − 3 × 2) = 𝑖 ̂ (10 + 10) − 𝑗 ̂ (5 – 15) + 𝑘 ̂ (–2 – 6) = 20𝒊 ̂ + 10𝒋 ̂ – 8𝒌 ̂ = 2(10𝑖 ̂ + 5𝑗 ̂ – 4𝑘 ̂) Putting value of 𝑎 ⃗ & 𝑏 ⃗ in formula 𝑟 ⃗ = 𝑎 ⃗ + 𝜆𝑏 ⃗ ∴ 𝒓 ⃗ = (–𝑖 ̂ + 2𝑗 ̂ + 7𝑘 ̂) + 𝜆 × 2(10𝑖 ̂ + 5𝑗 ̂ – 4𝑘 ̂) = (–𝑖 ̂ + 2𝑗 ̂ + 7𝑘 ̂) + 2𝜆 (10𝑖 ̂ + 5𝑗 ̂ – 4𝑘 ̂) Putting 𝜇 = 2𝜆 = (–𝒊 ̂ + 2𝒋 ̂ + 7𝒌 ̂) + 𝝁 (10𝒊 ̂ + 5𝒋 ̂ – 4𝒌 ̂) Therefore, the equation of line is (–𝒊 ̂ + 2𝒋 ̂ + 7𝒌 ̂) + 𝝁 (10𝒊 ̂ + 5𝒋 ̂ – 4𝒌 ̂) Converting to cartesian form (𝑥 −(−1))/10=(𝑦 − 2)/5=(𝑧 − 7)/(−4) (𝒙 + 𝟏)/𝟏𝟎=(𝒚 − 𝟐)/𝟓=(𝒛 − 𝟕)/(−𝟒)
