CBSE Class 12 Sample Paper for 2025 Boards
You are here
CBSE Class 12 Sample Paper for 2025 Boards
Last updated at Feb. 12, 2025 by Teachoo
This question is similar to Chapter 7 Class 12 Integrals - Miscellaneous
Please check the question here
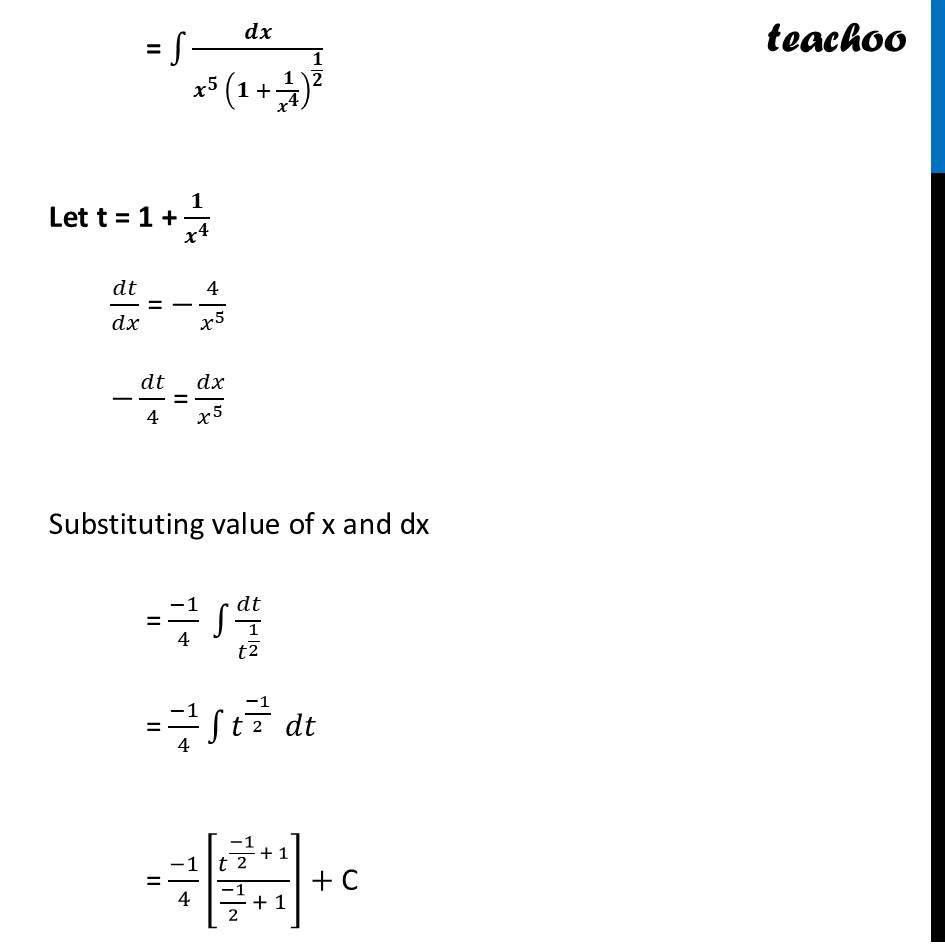
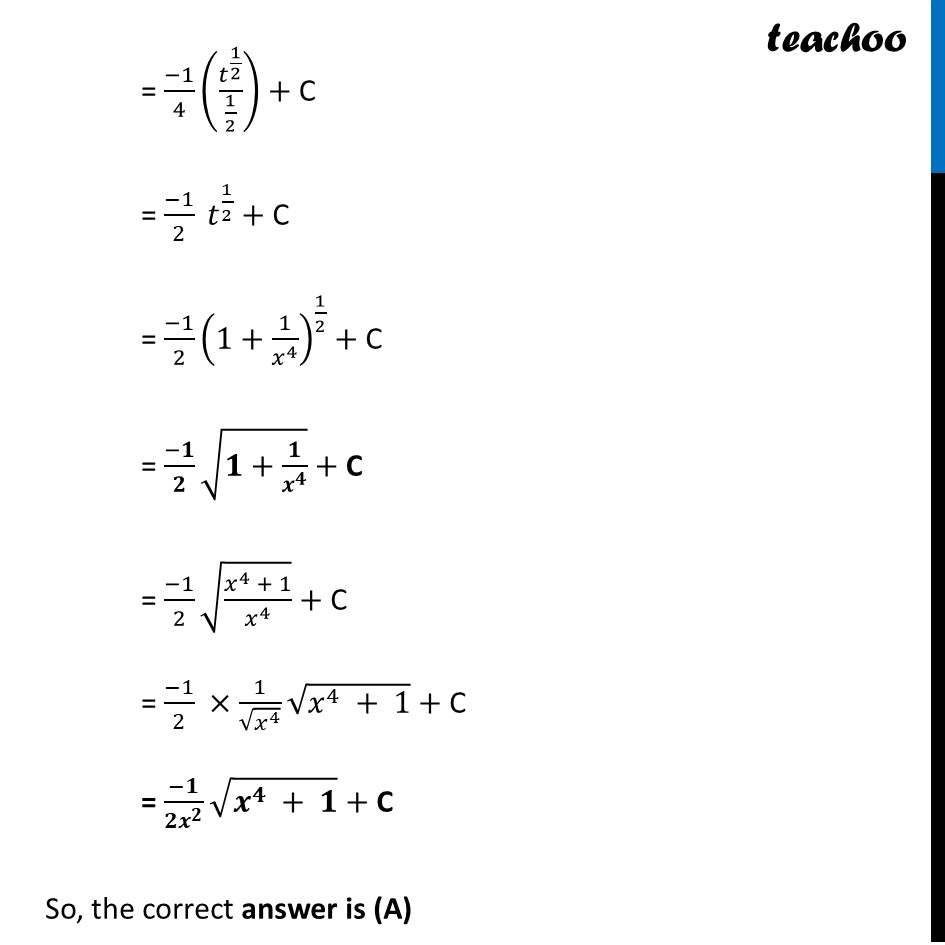
Transcript
Question 12 ∫𝑑𝑥/(𝑥^3 (1 + 𝑥^4 )^(1/2) ) equals (A) −1/(2𝑥^2 ) √(1+𝑥^4 )+𝑐 (B) 1/2𝑥 √(1+𝑥^4 )+𝑐 (C) −1/4𝑥 √(1+𝑥^4 )+𝑐 (D) 1/(4𝑥^2 ) √(1+𝑥^4 )+𝑐∫1▒( 𝑑𝑥)/(𝑥^3 (1 + 𝑥^4 )^(1/2) ) Taking 𝒙^𝟒 common from denominator = ∫1▒𝑑𝑥/(𝑥^(3 ) 〖〖(𝑥〗^4)〗^(1/2) ( 1/𝑥^4 + 1)^(1/2) ) = ∫1▒𝑑𝑥/(𝑥^3 〖(𝑥〗^2) (1 + 1/𝑥^4 )^(1/2) ) = ∫1▒𝒅𝒙/(𝒙^𝟓 (𝟏 + 𝟏/𝒙^𝟒 )^(𝟏/𝟐) ) Let t = 1 + 𝟏/𝒙^𝟒 𝑑𝑡/𝑑𝑥 = −4/𝑥^5 −𝑑𝑡/4 = 𝑑𝑥/𝑥^5 Substituting value of x and dx = (−1)/4 ∫1▒𝑑𝑡/𝑡^(1/2) = (−1)/4 ∫1▒𝑡^((−1)/2) 𝑑𝑡 = (−1)/4 [𝑡^((−1)/2 + 1)/((−1)/2 + 1)]+ C = (−1)/4 (𝑡^(1/2)/(1/2))+ C = (−1)/2 𝑡^(1/2)+ C = (−1)/2 (1+1/𝑥^4 )^(1/2)+ C = (−𝟏)/𝟐 √(𝟏+𝟏/𝒙^𝟒 )+ C = (−1)/2 √((𝑥^4 + 1)/𝑥^4 )+ C = (−1)/2 ×1/√(𝑥^4 ) √(𝑥^4 + 1)+ C = (−𝟏)/(𝟐𝒙^𝟐 ) √(𝒙^𝟒 + 𝟏)+ C So, the correct answer is (A)
