Question 28 - CBSE Class 10 Sample Paper for 2025 Boards - Maths Standard - Solutions of Sample Papers for Class 10 Boards

Last updated at Feb. 12, 2025 by Teachoo

Next: Question 29 Important → Share on WhatsApp

Transcript

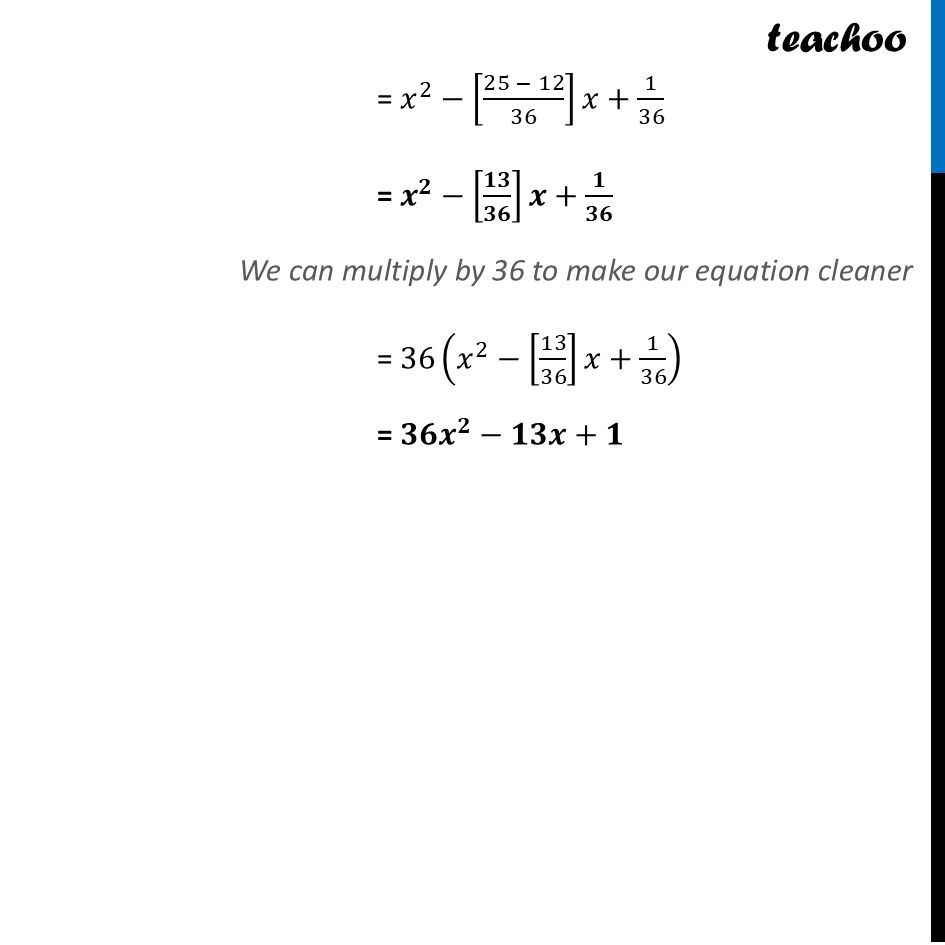
Question 28 If 𝛼 and 𝛽 are zeroes of a polynomial 6𝑥2 − 5x + 1 then form a quadratic polynomial whose zeroes are 𝛼2 and 𝛽2 .Let p(x) = 6𝑥2 − 5x + 1 Roots of p(x) are 𝛼 and 𝛽 So, Sum of roots = 𝛼 + 𝛽 = (−(−5))/6 = 𝟓/𝟔 And, Product of roots = 𝛼𝛽 = 𝟏/𝟔 We need to find quadratic polynomial whose zeroes are 𝛼2 and 𝛽2 . So, Required polynomial = q(x) = x2 − (Sum of Zeroes)x + Product of Zeroes = x2 − (𝛼2 + 𝛽2)x + 𝛼2 × 𝛽2 Using (a2 + b2) = (a + b)2 − 2an = x2 − [(𝛼 + 𝛽)2 − 2𝛼𝛽] x + (𝛼𝛽)2 Putting 𝛼 + 𝛽 = 5/6 & 𝛼𝛽 = 1/6 = 𝑥^2−[(5/6)^2−2 ×1/6]𝑥+(1/6)^2 = 𝑥^2−[25/36−1/3]𝑥+1/36 = 𝑥^2−[(25 − 12)/36]𝑥+1/36 = 𝒙^𝟐−[𝟏𝟑/𝟑𝟔]𝒙+𝟏/𝟑𝟔 We can multiply by 36 to make our equation cleaner = 36(𝑥^2−[13/36]𝑥+1/36) = 𝟑𝟔𝒙^𝟐−𝟏𝟑𝒙+𝟏

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 15 years. He provides courses for Maths, Science and Computer Science at Teachoo

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular