Misc 3 - Chapter 7 Class 11 Permutations and Combinations (Important Question)

Transcript

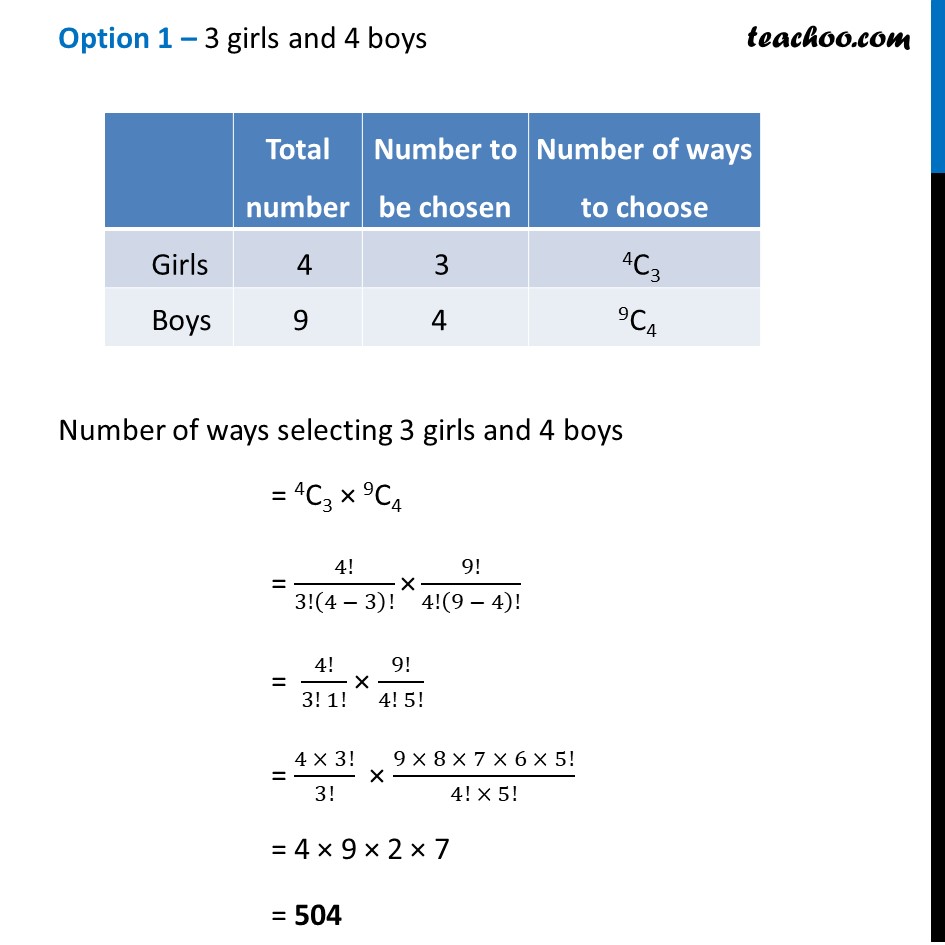
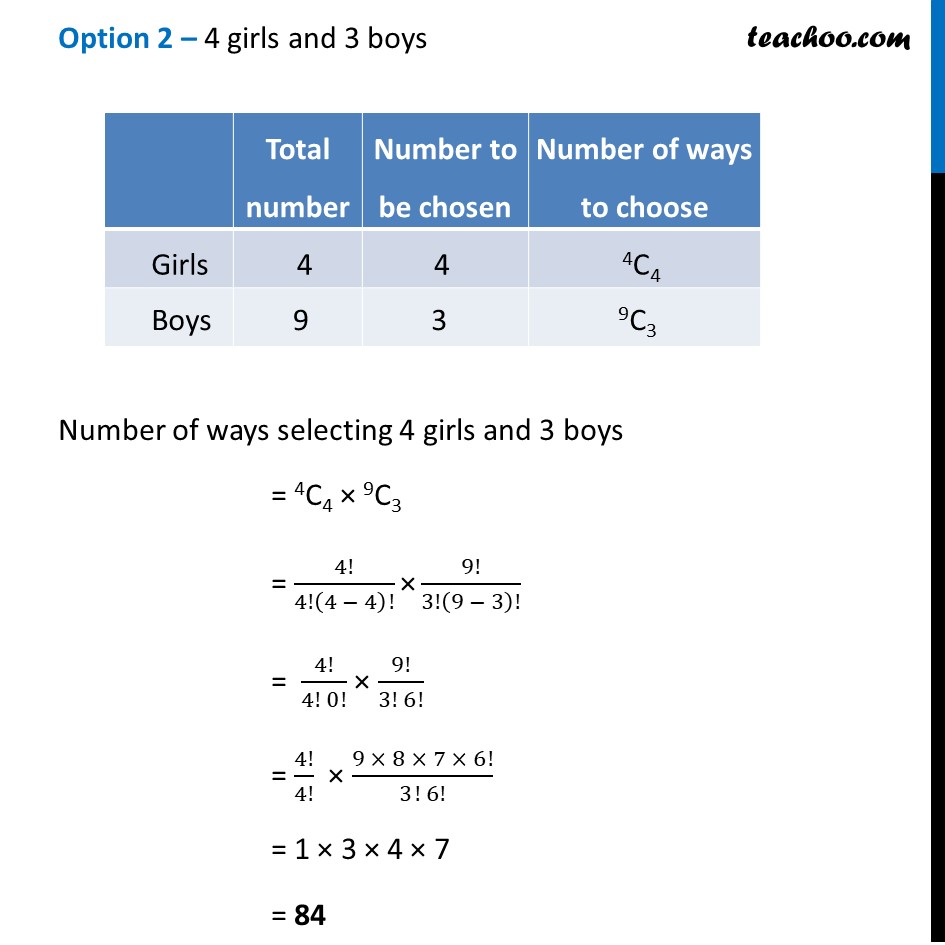
Misc 3 A committee of 7 has to be formed from 9 boys and 4 girls. In how many ways can this be done when the committee consists of: exactly 3 girls? Total number of ways = 4C3 × 9C4 = 4!/(3!(4 − 3)!) × 9!/4!(9 − 4)! = 4!/3!1! × 9!/4!(5)! = 9!/3!(5)! = (9 × 8 × 7 × 6 × 5!)/((3 × 2 × 1) × (5)!) = 504 Misc 3 A committee of 7 has to be formed from 9 boys and 4 girls. In how many ways can this be done when the committee consists of: (ii) atleast 3 girls? Since, the team has to consist of at least 3 girls, the team can consist of 3 girls and 4 boys 4 girls and 3 boys We have to calculate all these combinations separately and then add it Option 1 – 3 girls and 4 boys Number of ways selecting 3 girls and 4 boys = 4C3 × 9C4 = 4!/3!(4 − 3)! "×" 9!/4!(9 − 4)! = 4!/(3! 1!) × 9!/(4! 5!) = (4 × 3!)/3! × (9 × 8 × 7 × 6 × 5!)/(4! × 5!) = 4 × 9 × 2 × 7 = 504 Option 2 – 4 girls and 3 boys Number of ways selecting 4 girls and 3 boys = 4C4 × 9C3 = 4!/4!(4 − 4)! "×" 9!/3!(9 − 3)! = 4!/(4! 0!) × 9!/(3! 6!) = 4!/4! × (9 × 8 × 7 × 6!)/(3! 6!) = 1 × 3 × 4 × 7 = 84 Hence, Total number of ways = 504 + 84 = 588 ways Misc 3 A committee of 7 has to be formed from 9 boys and 4 girls. In how many ways can this be done when the committee consists of: (iii) at most 3 girls? Atmost means maximum, We have to choose maximum 3 girls Option 1 - 0 girls, 7 boys Option 2 - 1 girls, 6 boys Option 3 - 2 girls, 5 boys Option 4 - 3 girls, 4 boys We have to calculate all these combinations separately and then add it Option 1 – 0 girls and 7 boys Number of ways selecting 0 girls and 7 boys = 4C0 × 9C7 = 4!/0!(4 − 0)! "×" 9!/7!(9 − 7)! = 4!/(0! 4!) × 9!/(7! 2!) = 1 × (9 × 8 × 7! )/(7! × 2!) = 9 × 4 = 36 Option 2 – 1 girl and 6 boys Number of ways selecting 1 girl and 6 boys = 4C1 × 9C6 = 4!/1!(4 − 1)! "×" 9!/6!(9 − 6)! = 4!/(1! 3!) × 9!/(6! 3!) = (4 × 3!)/3! × (9 × 8 × 7 × 6!)/(6! × 3!) = 4 × 3 × 4 × 7 = 336 Option 3 – 2 girls and 5 boys Number of ways selecting 2 girl and 5 boys = 4C2 × 9C5 = 4!/2!(4 − 2)! "×" 9!/5!(9 − 5)! = 4!/(2! 2!) × 9!/(5! 4!) = (4 × 3 × 2!)/(2! 2!) × (9 × 8 × 7 × 6 × 5!)/(5! × 4!) = 2 × 3 × 9 × 2 × 7 = 756 Option 4 – 3 girls and 4 boys Number of ways selecting 3 girls and 4 boys = 4C3 × 9C4 = 4!/3!(4 − 3)! "×" 9!/4!(9 − 4)! = 4!/(3! 1!) × 9!/(4! 5!) = (4 × 3!)/3! × (9 × 8 × 7 × 6 × 5!)/(4! × 5!) = 4 × 9 × 2 × 7 = 504 Hence, Total number of ways = 36 + 336 +756 + 504 = 1632 ways