



Last updated at December 16, 2024 by Teachoo





Transcript
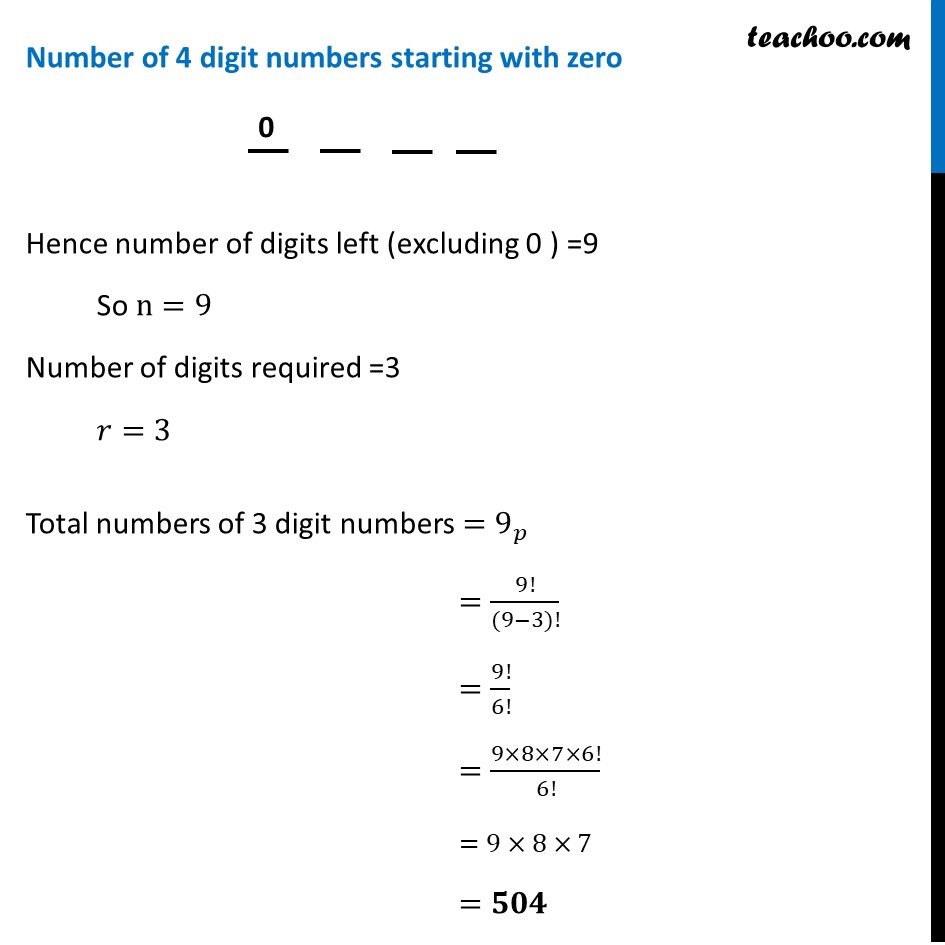
Ex 6.3, 2 (Method 1) How many 4-digit numbers are there with no digit repeated? We need to make 4 digit numbers using digits 0,1,2,3,4,5,6,7,8,9 But, these include numbers starting with ‘0’ like 0645, 0932, …etc which are actually 3 digit numbers Required numbers = Total 4 digit numbers – 4 digit number which have 0 in the beginning Total 4 digit numbers Total digits from 0 to 9 = 10 Hence, n = 10 We need to find 4 digit numbers Hence r = 4 So total number of four digits numbers = 10P4 = 10!/(10 − 4)! = (10 × 9 × 8 × 7 × 6!)/6! = 5040 Number of 4 digit numbers starting with zero Hence number of digits left (excluding 0) = 9 So n = 9 Number of digits required = 3 r = 3 Total numbers of 3 digit numbers = 9P3 = 9!/(9 − 3)! = 9!/6! = (9 × 8 × 7 × 6!)/6! = 9 × 8 × 7 = 504 Ex 6.3, 2 (Method 2) How many 4-digit numbers are there with no digit repeated? Four digit numbers are We need to form 4 digit number using (0, 1, 2, 3, … 9) digits Hence number of digits = 10 If 0 is in first place, the number will be like 0645, 0932,etc which is a three-digit number. So, 0 is not possible in first place. Total 4 digit numbers = 9 × 9 × 8 × 7 = 4536 ways