Example 3 - Mathematical Induction

Last updated at Dec. 16, 2024 by

Example 3 - Prove 1/1.2 + 1/2.3 + 1/3.4 .. + 1/n(n + 1) = 1/n+1 - Equal - 1 upon addition

Example 3 - Chapter 4 Class 11 Mathematical Induction - Part 2
Example 3 - Chapter 4 Class 11 Mathematical Induction - Part 3
Example 3 - Chapter 4 Class 11 Mathematical Induction - Part 4

Go Ad-free

Transcript

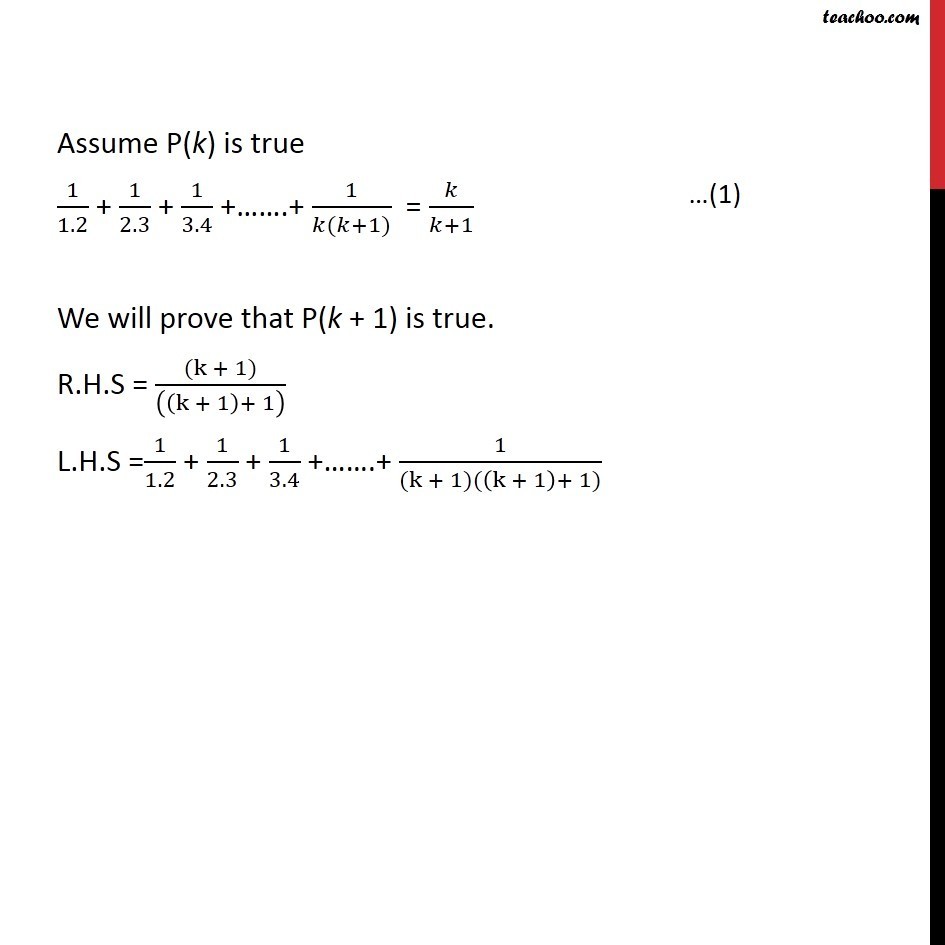
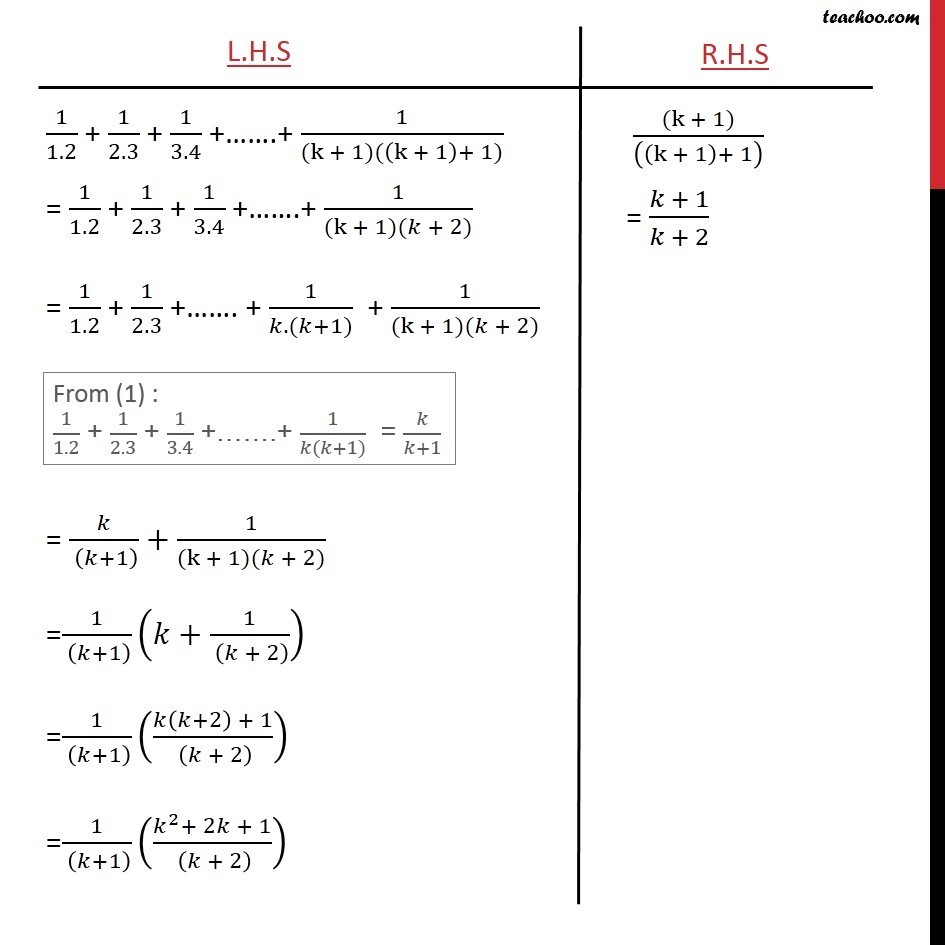
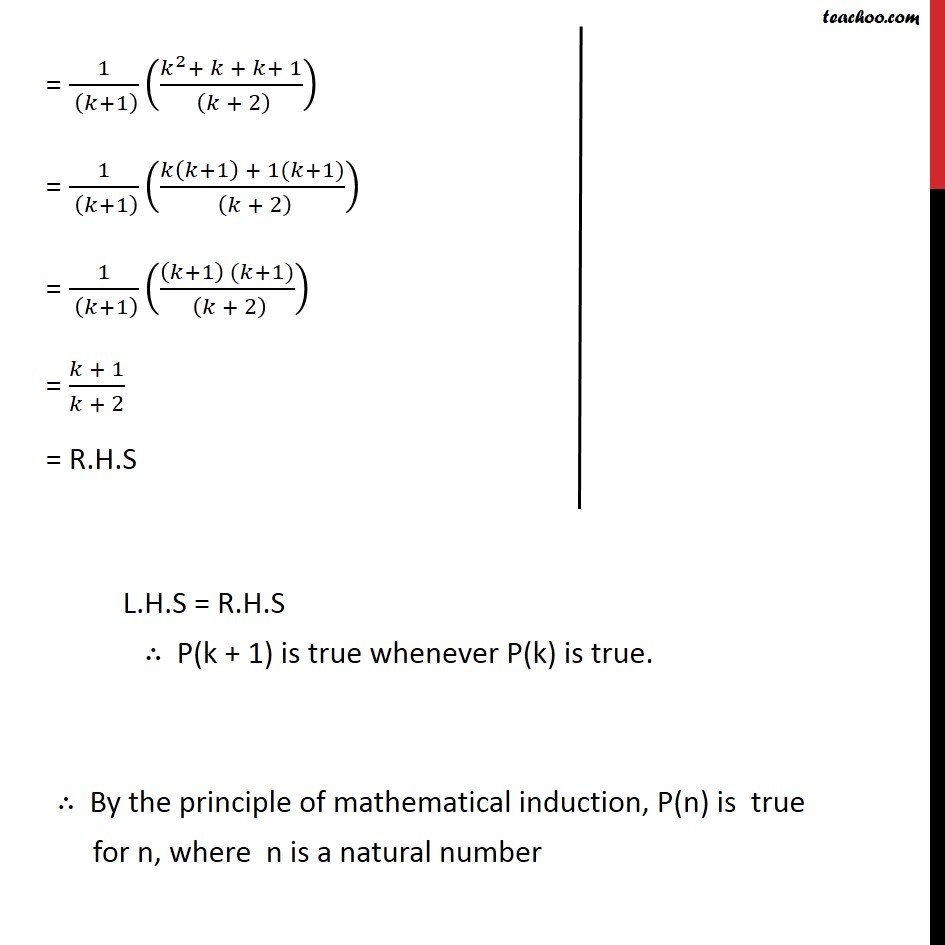
Example 3 For all n ≥ 1, prove that 1/1.2 + 1/2.3 + 1/3.4 +…….+ 1/(𝑛(𝑛 + 1)) = 1/(𝑛 + 1) Let P (n) : 1/1.2 + 1/2.3 + 1/3.4 +…….+ 1/(𝑛(𝑛 + 1)) = 1/(𝑛 + 1) For n=1, L.H.S = 1/1.2 = 1/2 R.H.S = 1/(1+1) = 1/2 Hence, L.H.S. = R.H.S , ∴ P(n) is true for n = 1 Assume P(k) is true 1/1.2 + 1/2.3 + 1/3.4 +…….+ 1/(𝑘(𝑘+1)) = 𝑘/(𝑘+1) We will prove that P(k + 1) is true. R.H.S = ((k + 1))/(((k + 1)+ 1) ) L.H.S =1/1.2 + 1/2.3 + 1/3.4 +…….+ 1/((k + 1)((k + 1)+ 1)) ∴ By the principle of mathematical induction, P(n) is true for n, where n is a natural number

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo