Misc 5 - Chapter 3 Class 11 Trigonometric Functions

Transcript

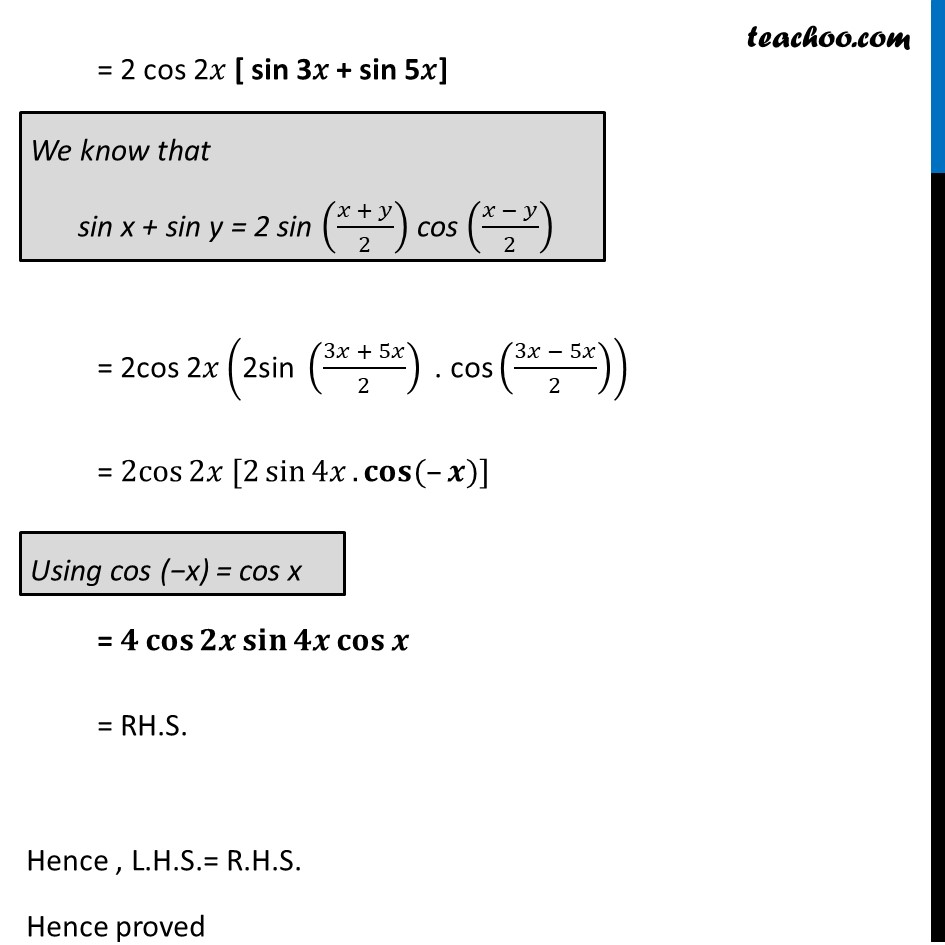
Misc 5 Prove that: sin 𝑥 + sin 3𝑥 + sin5𝑥 + sin 7𝑥 = 4cos 𝑥 cos 2𝑥 sin 4𝑥 Solving LHS sin 𝑥 + sin 3𝑥 + sin5𝑥 + sin 7𝑥 = (𝐬𝐢𝐧⁡𝒙+𝒔𝒊𝒏 𝟓𝒙)+(𝐬𝐢𝐧⁡𝟑𝒙+𝒔𝒊𝒏⁡𝟕𝒙) = 2 sin ((𝑥 + 5𝑥)/2) .cos ((𝑥 − 5𝑥)/2) + 2sin ((3𝑥 + 7𝑥)/2) cos ((3𝑥 − 7𝑥)/2) = 2 sin 3𝑥 cos (–2𝑥) + 2sin 5𝑥 cos (–2𝑥) = 2 sin 3𝑥 cos 2𝑥 + 2sin 5𝑥 cos 2𝑥 = 2 cos 2𝑥 [ sin 3𝑥 + sin 5𝑥] = 2cos 2𝑥 ("2sin " ((3𝑥 + 5𝑥)/2)" . cos" ((3𝑥 − 5𝑥)/2)) = 2cos⁡2𝑥 [2 sin⁡4𝑥.cos⁡〖(–𝑥)〗] = 𝟒 𝐜𝐨𝐬⁡𝟐𝒙 𝐬𝐢𝐧⁡𝟒𝒙 𝐜𝐨𝐬⁡𝒙 = RH.S. = 2 cos 2𝑥 [ sin 3𝑥 + sin 5𝑥] = 2cos 2𝑥 ("2sin " ((3𝑥 + 5𝑥)/2)" . cos" ((3𝑥 − 5𝑥)/2)) = 2cos⁡2𝑥 [2 sin⁡4𝑥.𝐜𝐨𝐬⁡〖(–𝒙)〗] = 𝟒 𝐜𝐨𝐬⁡𝟐𝒙 𝐬𝐢𝐧⁡𝟒𝒙 𝐜𝐨𝐬⁡𝒙 = RH.S. Hence , L.H.S.= R.H.S. Hence proved