Question 28 (Choice 1) - CBSE Class 12 Sample Paper for 2024 Boards - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Last updated at Feb. 10, 2025 by Teachoo

Find : ∫√(x/(1-x^3 )) dx; x∈(0,1)

This question is similar to Misc 12 Chapter 7 Class 12

[Class 12] Find ∫ √(x/1-x^3) dx - Teachoo Sample Paper (with Video) - CBSE Class 12 Sample Paper for 2024 Boards

part 2 - Question 28 (Choice 1) - CBSE Class 12 Sample Paper for 2024 Boards - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards - Class 12

Next: Question 28 (Choice 2) Important → Share on WhatsApp

Transcript

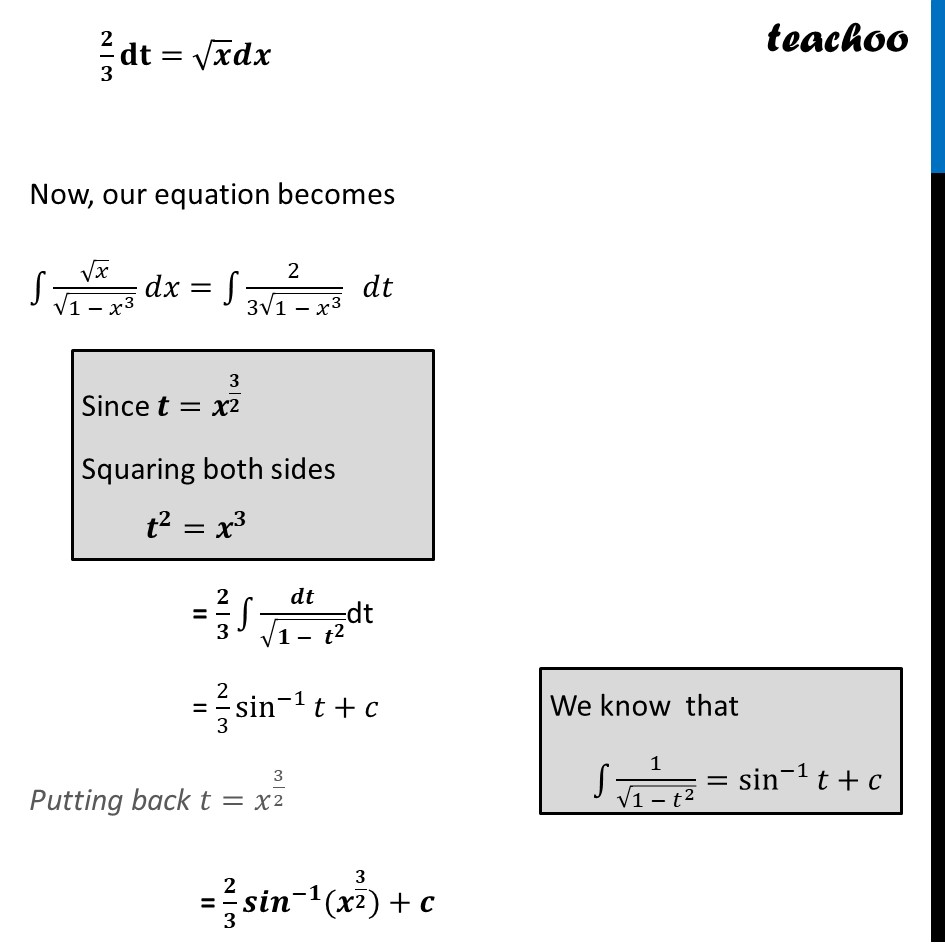
∫√(𝑥/(1 − 𝑥^3 )) 𝑑𝑥 = ∫1▒√𝑥/√(1 − 𝑥^3 ) 𝑑𝑥 Let 𝒕=𝒙^(𝟑/𝟐) Differentiating w.r.t. 𝑥 𝑑𝑡/𝑑𝑥=3/2 𝑥^(3/2 −1) 𝑑𝑡/𝑑𝑥=3/2 𝑥^(1/2 ) 𝑑𝑡/𝑑𝑥=3/2 √𝑥 𝟐/𝟑 𝐝𝐭=√𝒙 𝒅𝒙 Now, our equation becomes ∫1▒√𝑥/√(1 − 𝑥^3 ) 𝑑𝑥=∫1▒〖2/(3√(1 −〖 𝑥〗^3 )) 𝑑𝑡〗 = 𝟐/𝟑 ∫1▒𝒅𝒕/√(𝟏 − 𝒕^𝟐 )dt = 2/3 sin^(−1)⁡〖𝑡+𝑐〗 Putting back 𝑡=𝑥^(3/2) = 𝟐/𝟑 〖𝒔𝒊𝒏〗^(−𝟏)⁡〖〖(𝒙〗^(𝟑/𝟐))+𝒄〗

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 15 years. He provides courses for Maths, Science and Computer Science at Teachoo

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular