CBSE Class 12 Sample Paper for 2024 Boards

CBSE Class 12 Sample Paper for 2023 Boards →
Class 12
Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Question 4 - CBSE Class 12 Sample Paper for 2024 Boards - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Last updated at Dec. 13, 2024 by

If f(x)={(kx/(|x|), if  x<0 , 3 if  x≥0)} is continuous at x=0, then the value of k is

(a) -3                (b) 0                 (c) 3              (d) any real number

This question is similar to Ex 5.1, 28 - Chapter 5 Class 12

Slide10.JPG

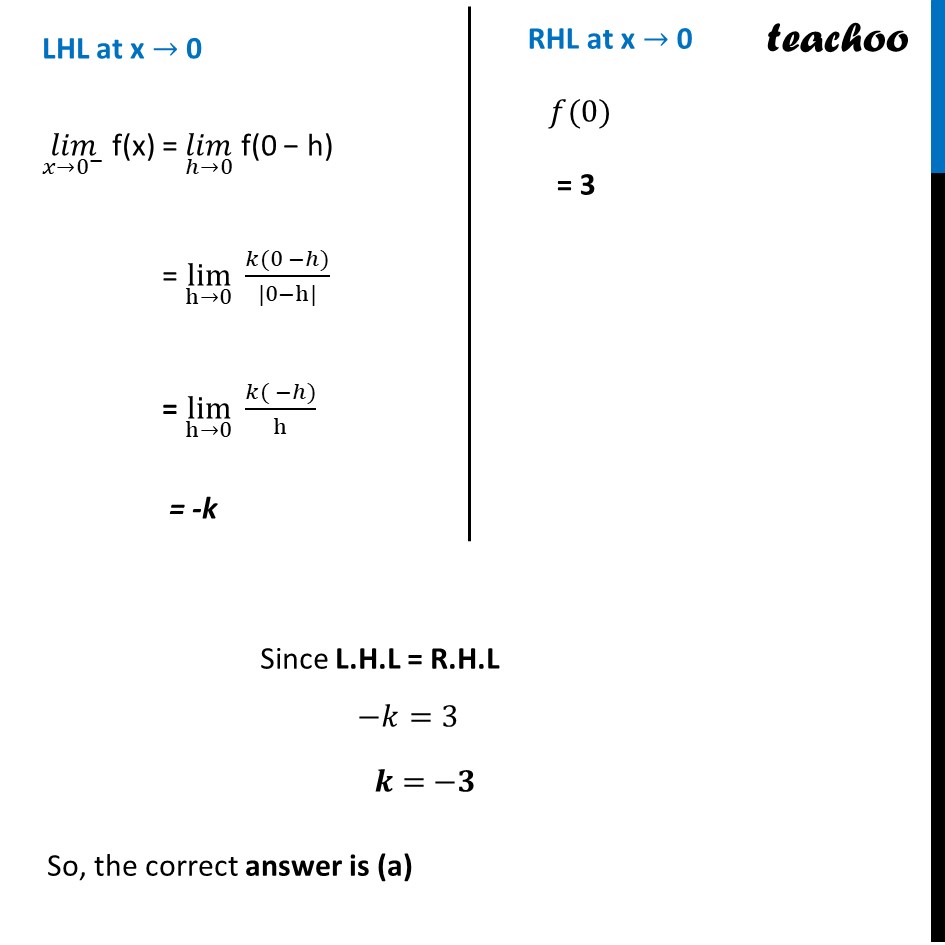
Slide11.JPG

Go Ad-free

Transcript

Given that function is continuous at π‘₯=0 𝑓 is continuous at x =0 If L.H.L = R.H.L = 𝑓(0) i.e. lim┬(xβ†’0^βˆ’ ) 𝑓(π‘₯)=lim┬(xβ†’0^+ ) " " 𝑓(π‘₯)= 𝑓(0) LHL at x β†’ 0 (π‘™π‘–π‘š)┬(π‘₯β†’0^βˆ’ ) f(x) = (π‘™π‘–π‘š)┬(β„Žβ†’0) f(0 βˆ’ h) = lim┬(hβ†’0) (π‘˜(0 βˆ’β„Ž))/(|0βˆ’h|) = lim┬(hβ†’0) (π‘˜( βˆ’β„Ž))/h = -k Since L.H.L = R.H.L βˆ’π‘˜=3 π’Œ= βˆ’πŸ‘

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo