You are here
Example 3 - Chapter 3 Class 11 Trigonometric Functions
Last updated at Dec. 16, 2024 by Teachoo
Chapter 3 Class 11 Trigonometric Functions
Concept wise
- Radian measure - Conversion
-
Arc length
- Finding Value of trignometric functions, given other functions
- Finding Value of trignometric functions, given angle
- (x + y) formula
- 2x 3x formula - Proving
- 2x 3x formula - Finding value
- cos x + cos y formula
- 2 sin x sin y formula
- Finding Principal solutions
- Finding General Solutions
- Sine and Cosine Formula
Transcript
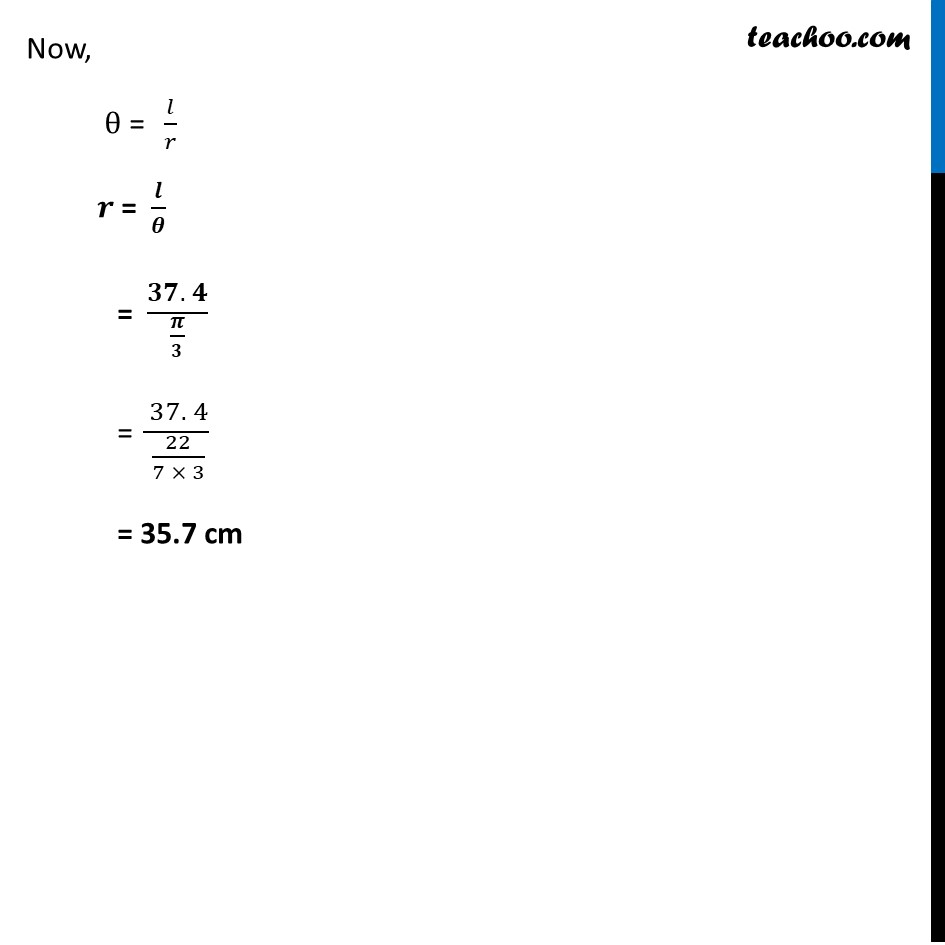
Example 3 Find the radius of the circle in which a central angle of 60° intercepts an arc of length 37.4 cm (use π = 22/7 ) Given 𝑙 = 37.4 cm and θ = 60° Converting angle into radians We know that Radian measure = 𝝅/𝟏𝟖𝟎 × Degree measure = 𝜋/180 × 60 = 𝝅/𝟑 radian Now, θ "= " 𝑙/𝑟 " " 𝒓 = 𝒍/𝜽 = (𝟑𝟕. 𝟒)/(𝝅/𝟑) = (37. 4)/(22/(7 × 3)) = 35.7 cm
